Negative of Quantum Gates and Entangled States

Question

I'm well aware that using this quantum circuit:

$\hskip2in$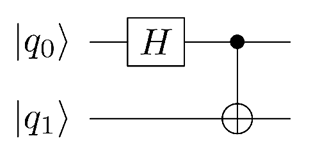
^{$\hskip2in$Quantum circuit used in the generation of EPR pairs.}

It is possible to create entangled state of two qubits (EPR pairs), in particular: $$\def\braket#1#2{\langle#1|#2\rangle}\def\bra#1{\langle#1|}\def\ket#1{|#1\rangle} \ket{\beta_{00}}= \frac{1}{\sqrt{2}}(\ket{00}+\ket{11})\\ \ket{\beta_{01}}= \frac{1}{\sqrt{2}}(\ket{01}+\ket{10})\\ \ket{\beta_{10}}= \frac{1}{\sqrt{2}}(\ket{00}-\ket{11})\\ \ket{\beta_{11}}= \frac{1}{\sqrt{2}}(\ket{01}-\ket{10})$$

But it seems possible to have two more entangled state resembling the EPR pairs: $$\def\braket#1#2{\langle#1|#2\rangle}\def\bra#1{\langle#1|}\def\ket#1{|#1\rangle} \ket{\beta_{??}}= \frac{1}{\sqrt{2}}(\ket{10}-\ket{01})\\ \ket{\beta_{??}}= \frac{1}{\sqrt{2}}(\ket{11}-\ket{00})$$

Which can be generated passing the initial qubit $\ket{q_0}$ in the negative of the Hadamard gate: $$ -H=\frac{1}{\sqrt{2}}\begin{pmatrix} -1 & -1 \\ -1 & 1 \end{pmatrix} $$ And then in the CNOT gate as usual.

The negative Hadamard gate is an unitary operator, then I assume likely to be used as a quantum gate (as it is said here and, for example in the book by Chuang and Nielsen).

The questions are two:

-Are the two latter EPR pairs legitimate entangled states? If yes, why they are not used in literature alongside the four original Bell states? If no, why?

-Is the negative of a quantum gate in turn a legitimate quantum gate? Is there a particular motivation for the use of the "classical" Hadamard gate instead of its negative?

Thank you in advance!

Answer 1

Global phases are physically irrelevant. Thus, $H$ and $-H$ are the same gate, as are $|\psi\rangle$ and $-|\psi\rangle$; for instance, the states you list are the same as two of the Bell states above up to a global minus sign.