Why does time dilation require events to occur at same point in space?

Question

I was reading through "University Physics with Modern Physics ~ Sears and Zemansky" and they mentioned the following paragraph in time dilation :

We may generalize this important result. In a particular frame of reference, suppose that two events occur at the same point in space. The time interval between these events, as measured by an observer at rest in this same frame (which we call the rest frame of this observer), is $\Delta t_0 $. Then an observer in a second frame moving with constant speed u relative to the rest frame will measure the time interval to be $\Delta t $, where

$$\Delta t = \frac{\Delta t_0}{\sqrt{1-u^2/c^2}} $$

My question is why did they impose the condition that events occur at same point in space?

Answer 1

"length contraction" and "time dilation" are special cases, useful to simplify certain kinds of discussions.

The Lorentz transforms mix time and space coordinates:

$ x' = \gamma (x - vt) $

$ t' = \gamma \big(t - \frac{vx}{c^2})$

That mixing is really interesting. It leads to changes to our idea of simultaneity (whether two thing happen at the same time) that underlie a lot of the "paradoxes" one encounters.

But the math is clearly simpler if $x$ and $x'$ don't involve $t$, i.e. you're talking about something that's "at the same time" in the unprimed frame. Then you get the simpler form of "length contraction". Ditto if $t$ and $t'$ don't involve $x$, the two events are "at the same place": time dilation.

Answer 2

If possible can someone even clear up what is meant by "the same point" ?

"At the same point" is a concept that is frame-dependent -- its definition depends on the observer's frame of reference. Conceptually, an observer in SR has surveying apparatus at their disposal (measuring rods, clocks, radio signals, clocks), which they can use to assign time and position coordinates to events. Therefore they can say whether two events happened at the same position.

My question is why did they impose the condition that events occur at same point in space?

What they're saying is that the clock runs fastest according to an observer at rest relative to the clock. If the clock is at rest, then the events consisting of the clock ticks are all at the same position in space.

Answer 3

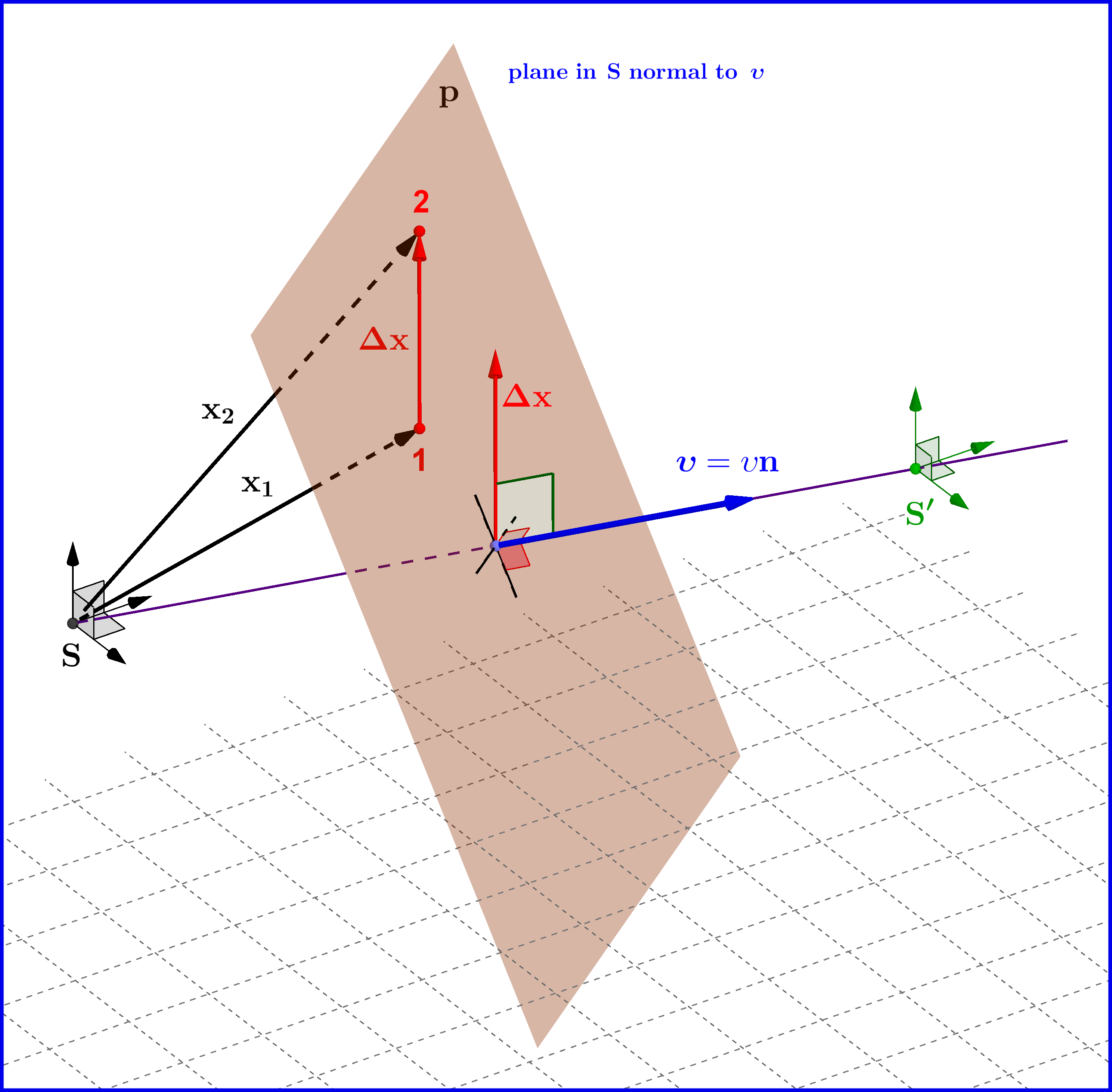
In above Figure an inertial system $\:\mathrm S'\:$ is translated with respect to the inertial system $\:\mathrm S\:$ with constant velocity
\begin{equation} \boldsymbol{\upsilon}=\left(\upsilon_{1},\upsilon_{2},\upsilon_{3}\right)=\left(\upsilon \mathrm n_{1},\upsilon \mathrm n_{2},\upsilon \mathrm n_{3}\right)=\upsilon \mathbf n\,, \qquad \upsilon \in \left(-c,c\right) \tag{01} \end{equation} The Lorentz transformation in difference form is \begin{align} \Delta t^{\boldsymbol{\prime}} & = \gamma\left(\Delta t-\dfrac{\boldsymbol{\upsilon}\boldsymbol{\cdot} \Delta \mathbf{x}}{c^{2}}\right) \tag{02a}\\ \Delta \mathbf{x}^{\boldsymbol{\prime}} & = \Delta \mathbf{x}+(\gamma-1)(\mathbf{n}\boldsymbol{\cdot} \Delta \mathbf{x})\mathbf{n}-\gamma \boldsymbol{\upsilon}\Delta t \tag{02b} \end{align} Now, let a plane $\:\mathrm p\:$ in $\:\mathrm S\:$ normal to the velocity $\:\boldsymbol{\upsilon}\:$, as in Figure, and let two events \begin{align} \mathbf{Event_1} & = \left(\mathbf{x_1},t_1\right) \tag{03a}\\ \mathbf{Event_2} & = \left(\mathbf{x_2},t_2\right) \tag{03b} \end{align} both happening on the plane $\:\mathrm p\:$ apart in space by the 3-vector $\:\Delta\mathbf{x}=\mathbf{x_2}-\mathbf{x_1}\:$ and at time apart by $\:\Delta t=t_2-t_1$. Then \begin{equation} \Delta\mathbf{x}\in \mathrm p \quad \Longrightarrow \quad \Delta\mathbf{x}\boldsymbol{\perp}\boldsymbol{\upsilon}\quad \Longrightarrow \quad \boldsymbol{\upsilon}\boldsymbol{\cdot} \Delta \mathbf{x}=0 \quad \overset{(02a)}{=\!=\!\Longrightarrow} \quad \Delta t^{\boldsymbol{\prime}} = \gamma\,\Delta t \tag{04} \end{equation} that is the case of time dilation \begin{equation} \Delta t^{\boldsymbol{\prime}} = \gamma\,\Delta t=\dfrac{\Delta t}{\sqrt{1-\dfrac{\upsilon^2}{c^2}}} \tag{05} \end{equation} The time dilation equation is valid of course in case that the two events happen at the same point in space, that is $\:\Delta\mathbf{x}=\mathbf{x_2}-\mathbf{x_1}=\boldsymbol{0}$.

So

Time dilation requires events to occur at the same point in space ($\:\Delta\mathbf{x}=\mathbf{x_2}-\mathbf{x_1}=\boldsymbol{0}$) or on a plane normal to the velocity vector ($\:\boldsymbol{\upsilon}\boldsymbol{\cdot} \Delta \mathbf{x}=0$).