Help on understanding unit normal vector

Question

Suppose that $\vec{r}(t)$ is a vector such that $||\vec{r}(t)||=c$ for all $t$.

Then $\vec{r'}(t)$ is orthogonal to $\vec{r}(t)$.

This is a litle hard for me to wrap my head around. I was wondering if anyone had any good analogies to help me understand this.

So far, I thought about magnetic force. The magnetic force always point perpendicular to the velocity of the particle so it can't do work. That means that r(t) would not increase (because force r'(t) does not do work) so it would be constant (c).

Is this a right conceptual way of thinking about it?

Answer 1

The magnetic force always point perpendicular to the velocity of the particle so it can't do work.

I think the last part of your argumentation is not correct, as you connected a force directly with the direction of the particle. To find the small changes of $\vec{r}$ it is needed to analyse $\vec{v}$ not $\vec{a} \propto \vec{F}$

But the example of a circular movement by a magnetic field is good. If you have $ \forall t : ||\vec{r}(t)|| = c $, this means, that the length of your radial coordinate is constant over time. Now we think of the velocity vector $\vec{v}$ as a small change of the current position, then the connection line between two points of your moving object is on the circle. As the radius of the circle is perpendicular to a tangent at the circle, $\vec{r}(t) \perp \vec{v}(t)$. This is illustrated by the following figure.

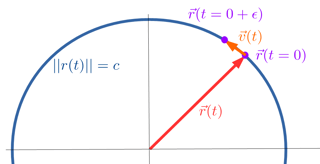
In this figure, the velocity vector is not exactly perpendicular, as the timestep between two points needs to be infinitesimal small to be a derivation $\frac{d\vec{r}}{dt} = \vec{v}$.

Now we analyse the differences between $\vec{v}(t)$ by using $\vec{a}(t)$. So it is useful, to think of the changes, we need to make, to get $\vec{v}$ at the next point. This change can be given by a vector directing to the center of the circle. In other words: The vector $\vec{v}(t)$ needs to be turned in the direction of the center-point of the circle. And again $\vec{v}(t) \perp \vec{a}(t)$.

The magnetic force always point perpendicular to the velocity of the particle so it can't do work.

Your assumptions are correct, but there is no direct connection $\vec{a} \perp \vec{v} \Rightarrow \vec{v} \not\perp \vec{r}$.

That means that r(t) would not increase (because force r'(t) does not do work) so it would be constant (c).

In this part of your argumentation it is important, that $r'(t) = v(t)$ is not a force, the right quantity would be $\vec{a}$, as you read from Newton's law $\vec{F} = m \vec{a}$. Nevertheless this problem can be solved without taking forces into account. As the forces result in $\vec{a}$.

Answer 2

Resolve $\vec{r'(t)}$ into components parallel and perpendicular to $\vec{r}$. Assume, for a moment that the component parallel to $\vec{r}$ is non-zero. This would change the length of $\vec{r}$, won't it? Thus if you want to hold the length of $\vec{r}$ to be constant, then this component, contrary to our assumption has to vanish. All there is left is the perpendicular part.

Answer 3

Perhaps inverting the statement can help, but it's not really an analogy:

You have the statement $\forall t~~||\vec{r}(t)||=c ~~\Rightarrow~~ \vec{v}(t) \bot \vec{r}(t)$.

This is equivalent to $ \vec{v}(t) ~\not\bot~ \vec{r}(t) ~~\Rightarrow~~ ||\vec{r}(t)|| \text{ is not constant}$.

(Note that I used $\vec{v}$ instead of $\vec{r'}$.)

Perhaps the second statement is more intuitive: if the velocity of the object is not perpendicular to the vector pointing from the origin the object, the object will move away from or closer to the origin, and this of course changes the length of $\vec{r}$.