During inflation there are quantum fluctuations in the inflaton field. Do quantum fluctuations in the inflaton field lead to fluctuations in the energy density?
2 Answers
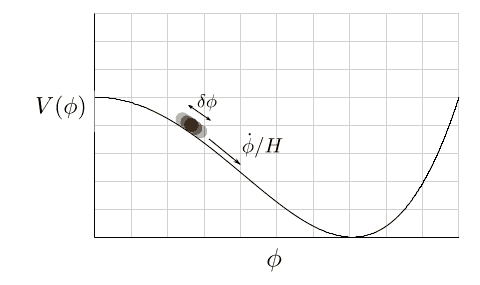
Yes: inflaton fluctuations give rise to energy density perturbations in the post-inflationary universe. Since the inflaton is a quantum field, the function $\phi({\bf x})$ at a particular instant in time will vary from place to place. Dynamically, we understand this as follows: while the classical motion of the inflaton flows strictly from high to low potential energy, as it "rolls down'' the potential energy function there is some fuzziness to its actual trajectory,
Quantum jitters cause the field to take small, discrete hops up or down the potential, resulting in small variations in field energy across space.
At any particular instant in time, space looks like this:

which is a random superposition of separate inflating regions; each inflating region is caused by a fluctuation of the inflaton field about the average density.
Once a fluctuation occurs somewhere in space, that region undergoes inflation according to the classical energy density there; after a time, this inflating region might give rise to more fluctuations. At any instant in time, these later fluctuation events appear as smaller inflating regions within the larger, earlier-nucleated regions.
The end result of all this is that different parts of the universe will end inflation at different times. The reason is easy to see: as the classical motion of the field approaches the point along the potential at which the inflationary condition ceases to hold, quantum fluctuations might kick the field further down the potential in one place, ending inflation there, while somewhere else a fluctuation might send the field back up the potential, nucleating new inflationary regions. Eventually inflation comes to end, albeit at different times, across a region of space roughly the size of today's Hubble sphere. Those places that ended inflation earlier reheated sooner, undergoing non-inflationary expansion for longer: the result is a bumpy energy landscape across space at the end of inflation.
The amplitude of the fluctuation is determined by the length of time it undergoes non-inflationary expansion relative to other regions in the Hubble patch, $\delta t$. Consider a region of the universe that stopped inflating a time $\delta t$ sooner than another region. According to the continuity equation, the difference in density is proportional to this time shift, \begin{equation} \label{dt} \frac{\delta \rho}{\bar{\rho}} = \delta = cH\delta t, \end{equation} where the constant $c$ is determined by the dominant form of energy after inflation.
(Credit for Image 2 above: Image is a still frame from the animated gif found at http://www.astro.ucla.edu/~wright/CMB-MN-03/epas.html)
-
$\begingroup$ How does quantum fluctuations cause the inflaton field to move back up the potential? $~$By causing energy density of the field to vary at different places, or by causing value of the field to vary at different places? $\endgroup$– parkerCommented Sep 24, 2018 at 2:05
-
$\begingroup$ The fluctuation is in the field value, and since the potential energy is a function of the field, the field fluctuation is manifested as an energy density fluctuation. $\endgroup$– bapowellCommented Sep 24, 2018 at 14:53
Quantum fluctuations in the microscopic inflationary region, magnified to cosmic size, become the seeds for the growth of structure in the Universe (see galaxy formation and evolution and structure formation).
As the inflaton fluctuations are modeled to be the seeds of the cosmic microwave background radiation and the locations of the distribution of matter in the present universe, the end result is fluctuations in energy density. Thus I would answer with a yes for the energy density. Now potential in this scenario is not well defined.