Is this interpretation of quantum fluctuation in eternal inflation in Wikipedia correct?

Question

Wikipedia's article on inflation says

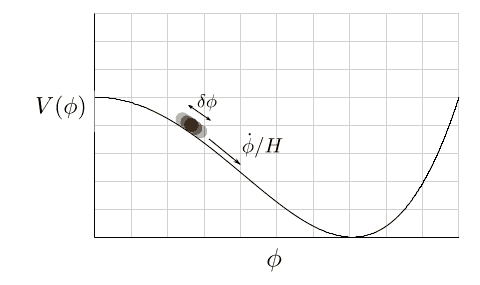
Although new inflation is classically rolling down the potential, quantum fluctuations can sometimes lift it to previous levels. These regions in which the inflaton fluctuates upwards expand much faster than regions in which the inflaton has a lower potential energy, and tend to dominate in terms of physical volume.

But from Sean Carroll’s article,

Eternal inflation is a different story. The idea there is that the inflaton field slowly rolls down its potential during inflation, except that quantum fluctuations will occasionally poke the field to go higher rather than lower. When that happens, space expands faster and inflation continues forever. This story relies on the idea that the “fluctuations” are actual events happening in real time, even in the absence of measurement and decoherence. And we’re saying that none of that is true. The field is essentially in a pure state, and simply rolls down its potential

So, I asked a friend of mine who knows QFT and he said

I never liked the concept of quantum fluctuations, especially when it comes to cosmology. In QFT, the fields always roll down to the exact minimum of the potential. It doesn't fluctuate in any meaningful sense. But the potential is the quantum mechanical one, not the classical one. The quote in the wikipedia may be a vague way to say that the classical potential acquires quantum corrections. Whether that picture is useful or not is beyond me.”

Is the interpretation of quantum fluctuation in Wikipedia correct from the point of view of QFT? Or does the inflaton field just simply roll down its potential without any effects from quantum fluctuation?

Answer 1

''Is the interpretation of quantum fluctuation in Wikipedia correct from the point of view of QFT? Or does the inflaton field just simply roll down its potential without any effects from quantum fluctuation?''

The inflaton field rolls down a potential that is modified by quantum effects. The latter are determined by corrections typically computed in perturbation theory in terms of vacuum correlations.

The latter can be named vacuum fluctuations, but this is highly misleading as it has nothing to do with fluctuations in any temporal sense. See the detailed explanations towards the end of my article https://www.physicsforums.com/insights/physics-virtual-particles/

Answer 2

The classical inflaton potential does receive quantum corrections from the other fields in the theory, but quantum fluctuations of the inflaton field itself have much greater significance during inflation. As a quantum field, the inflaton exhibits fluctuations about its classical trajectory, $\phi(x,t) = \phi_0(t) + \delta \phi(x,t)$. The result is a sort of fuzziness to the trajectory:

The fluctuations cause the inflaton to roll down the potential at effectively different rates at different places in the universe, with the result that inflation ends at different times, $\delta t$, in different places. Via the continuity equation, this gives rise to a density perturbation, $\delta \rho/\bar{\rho} \propto H \delta t$.

Answer 3

Classically, a particle rolls down a potential viz. $\frac{d}{dt}\mathbf{p}=-\boldsymbol{\nabla}V$. The equivalent for a classical field is $\frac{d}{dt}\pi=-\frac{\delta V}{\delta\phi}$. The first of these equations is quantum-corrected to $\frac{d}{dt}\langle\mathbf{p}\rangle=-\langle\boldsymbol{\nabla}V\rangle$, which allows for quantum tunnelling. Similarly, the second equation becomes $\frac{d}{dt}\langle\pi\rangle=-\langle\frac{\delta V}{\delta\phi}\rangle$.