Braking radiation (bremsstrahlung) and energy conservation

Question

I'm aware there is a similar question about this: Bremsstrahlung vs energy conservation

However, I don't think the answer given actually answers the question, so I'll ask again:

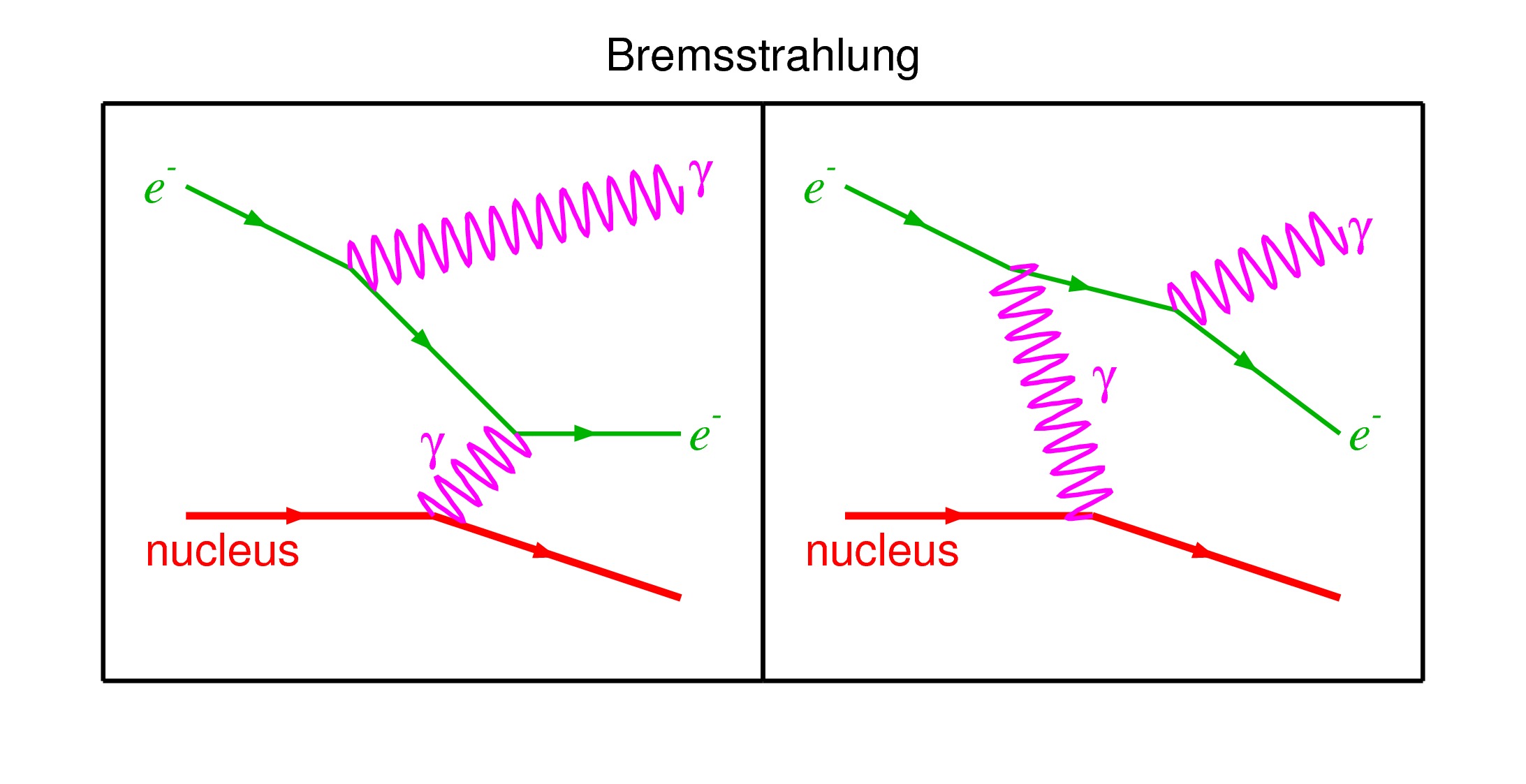
Let's consider a high velocity electron passing close to a nucleus. As it moves away from the nucleus, it loses kinetic energy whilst increasing its electrical potential energy by the same amount.

There must be something missing in my understanding about the formation of bremsstrahlung. If all the electron's loss of kinetic energy is converted to electrical potential energy as it leaves the nucleus, where does the energy come from to create a radiated photon?

Answer 1

Short answer: the electron loses a bit more kinetic energy than you would expect by naive energy conservation. That extra energy is accounted for by the emitted light. Classically the reason the electron loses more energy is because it experiences a backward radiation reaction force on it when it emits the light.

Better answer: there's really no such thing as "potential energy". Energy is always the energy of something, like the kinetic energy of a particle or the energy density of a field. Potential energy as used in introductory physics is a placeholder for some kind of energy we don't want to keep track of explicitly. For example, the potential energy of two static charges $$U(r) = \frac{q_1 q_2}{4 \pi \epsilon_0 r}$$ is really the energy of the static electric field they create, up to a constant.

In this situation, there is electromagnetic field energy and electron kinetic energy. Some of the latter is transferred to the former. There is no reason to expect that all of this energy will be in the form of static field energy, since the electron is accelerating; some appears as radiation. There's nothing particularly weird or paradoxical about this.

Answer 2

If all the electron's loss of kinetic energy is converted to electrical potential energy as it leaves the nucleus,

Even in ideal Coulomb theory, not all kinetic energy is converted to potential energy; the nucleus is pulled by the electron and receives some kinetic energy as well. Then, both electron and nucleus move with non-zero acceleration.

In fully relativistic electromagnetic theory with only retarded interactions, two charged particles that move with acceleration will act on each other with EM forces that are not purely Coulombic. These forces are non-conservative; this means some energy will apparently get lost, so sum of kinetic energies after the encounter will be less than before. Of course, this lost energy is not actually lost, but will leak out from the system as EM energy flux, due to EM radiation such system produces.

Answer 3

Potential energy is the part of the energy of an object the depends on its position, to be distinguished from kinetic energy, which depends on its velocity.

The concept is used in every part of physics, so examples are everywhere.

Answer 4

This belongs to the quantum mechanical regime because, bremsstrahlung appears on individual electrons, and electrons are elementary particles, they must be described quantum mechanically, and here are the feynman diagrams"

Potentials do not enter because in quantum electrodynamics their effect is taken over by the virtual photon exchanges.

There must be something missing in my understanding about the formation of bremsstrahlung. If all the electron's loss of kinetic energy is converted to electrical potential energy as it leaves the nucleus, where does the energy come from to create a radiated photon?

You are thinking classically about individual particles as an electron and a photon, that cannot be correct

The electron exchanges a virtual photon giving energy and momentum to the nucleus , and loses energy to a real photon.

Classically, if a charged particle decelerates it emits radiation. The diagram above is what happens at the quantum mecahnical correct level for electrons and photons and nuclei.