Running or walking up stairs = same work?

Question

I have a question belonging to the picture below. It is mentioned that

whether you walk up or run up stairs the same work is done.

When work equals (force in the moving direction) times the way, then I dont understand why it should be correct. When I run up the stairs I definitely accelerate much faster, while I "gain" more kinetic energy, caused by my velocity. On the other hand when I define my work by the negative difference of potential energy, this statement would be correct. Why is this not a contradiction?

It seems like I am running errors in correctly seperating physical systems, but I can't figure this out. Can you help me out?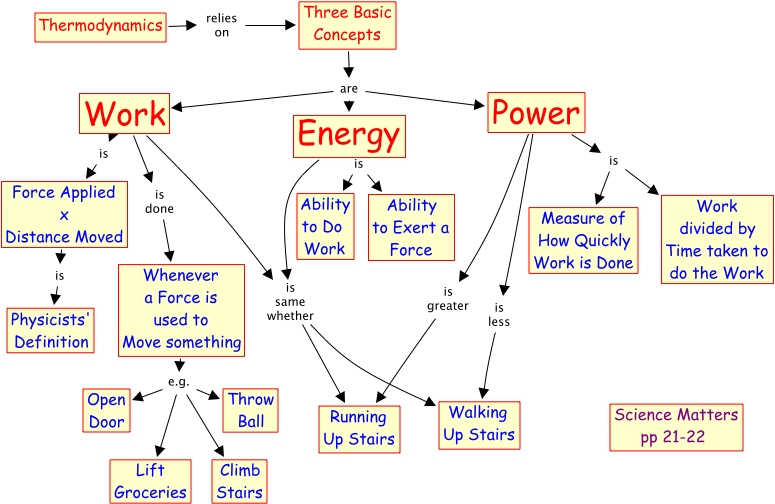

To make my question more precise: Say we reduce the whole thing to a simple straight vertical movement. The Force upwards is given by acceleration times mass. Faster movement upwards must be an increase of acceleration and so an increase in force and that will give an increase of work. Isn't that correct?

Answer 1

Suppose that you are standing stationary outside your house holding a 25kg sack of rice. Now walk 1km to your friend's house to give him the rice. He is not home so you return with the rice. You are now back where you started: stationary again with the sack of rice. Have you done any work? In a day to day sense, you have but you have neither gained not lost kinetic nor potential energy so you have done no work. You may have expended lots of energy but this has all gone to various inefficiencies.

Clarification. What I was trying to address was the distinction between work in its common day to day sense and its sense in physics. In my scenario, you have burned some food to make the trip and conservation of energy will not have been violated. So, the energy has gone somewhere. You and the sack of rice have no net gain in kinetic or potential energy so it has gone elsewhere. Most of it has become heat.

So did you do work? You probably felt that you had since you will be tired after the trip. Your wife who asked you to deliver the rice may think that you have not as the sack of rice is where it started. In physics, work has been done and the conservation of energy has not been violated, it is just that you might not notice or care where the work was done.

Back to your example, you might or might not have done more work by running up the stairs. You would need to determine the energy that went elsewhere than your kinetic or potential energy. This is possibly more a question of biology than physics. In which scenario is your body more efficient?

Answer 2

The work done against gravity (= increase in potential energy) is the same in both cases. Extra work is done if kinetic energy is increased.

If the walker and runner both start and end at rest (or at the same speed), then there is no overall increase in kinetic energy in either case. Any kinetic energy which is created by accelerating in between is used up again during deceleration to increase the potential energy.

If the walker and runner are moving faster at the top of the stairs than at the bottom then there is some increase in kinetic energy in both cases. Presumably the runner's increase in kinetic energy is greater.

$$\text{total work done by person = increase in potential energy + increase in kinetic energy}$$

The runner finishes with a greater increase in kinetic energy, so the total work done by the runner is greater.

---

Note that it is the overall increase in kinetic energy between start and finish which matters. It makes no difference how the kinetic energy has varied in between. In mechanical terms, person A who walks slowly for most of the way then runs the last few steps does more work than person B who runs most of the way then walks slowly the last few steps.

Person B uses more energy than person A, but this is a separate issue about the difference between external and internal work. Person B does less external work (he/she has created less kinetic energy) but more internal work (he/she has been more active and as a result gotten hotter). In mechanics we are usually only concerned about external work.

Answer 3

The confusion arises from the conflation of acceleration and work, and the intuition that higher acceleration means more energy. It is more clear if you examine the starting and ending states; they are equivalent.

Examining the acceleration component, this is counterintuitive because there is more energy exerted per unit of time, but you must also consider that there are fewer units of time. The total energy is, ideally, equivalent; the additional energy per unit of time is precisely counterbalanced by the reduced amount of time.

This can be unintuitive because running feels like more work, but this is an artifact of the way our bodies work (switching from aerobic to anaerobic, which is less efficient), rather than a characteristic of physics itself.

Answer 4

Suppose that all of your joints were frictionless, your body was perfectly rigid, that the energy required to start moving was provided by an ideal battery with no internal resistance, and that the energy used to move was recovered with 100 percent efficiency when you stopped. Then, indeed, you would do the same amount of work whether you're walking or running up the stairs. You could even spend two hours running in the opposite direction and then run back to the stairs and up them, and it would be the same amount of work. This is because, in your hypothetical perfect body, it costs you no energy to keep moving in a straight line at the same height, and you get back all of the energy you needed to start moving when you stop.

This is obviously not true in our squishy human bodies, with inefficient chemical power sources that generate a lot of heat even when we're standing still. Because of friction and inefficiency in the chemical processes which power your muscles, you continually lose energy when you're moving. The rate of energy dissipation and loss goes up when you move faster (which is why you tend to get hot when you're running), and it's precisely this fact that makes running up the stairs take more work than walking up them. But don't doubt your lectures because of this; in most physics courses, the above ideal body is assumed because it makes talking about concepts like work and energy much simpler. And in the case of an ideal body, your lectures are correct.