Resistance of a Large (relative to lead contact) Conductor

Question

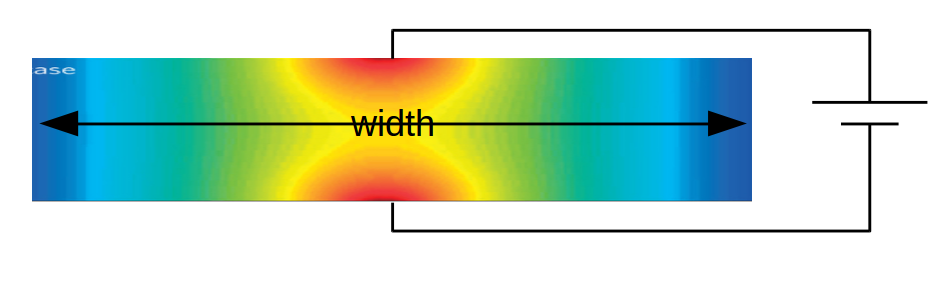
How do I calculate the effective resistance of a large piece of metal between two small electrical contacts? The thermal image (from an interesting but unrelated article called An Exception to Ohm's Law) better demonstrates what I'm asking.

From the temperature distribution, it is obvious where the electrical leads are connected, and it is also obvious that the electric field within the conductor is not uniformly distributed across the entire conductor. All of my physics books require the field to be uniform for the following to hold:

R = resistivity*length /cross-sectional

The problem can be conceptualized by allowing the conductor to become infinitely wide. There has to be some point at which additional material being added to the width no longer matters. You can also see a similar effect when simulating a large network of resistors, or a strip of graphite on paper.

I've demonstrated the effect on a sheet of paper. I colored onto the paper a 9x1cm rectangle made of graphite. I then placed an ohm-meter on opposite sides of the 9cm rectangle and measured the resistance. I then began making it wider. The resistance lowered for some time, but after increasing its width to approximately 4cm the resistance remained constant. I continued to make the strip as wide as 7cm and the resistance remained the same. I know this is far from a perfect experiment, but it demonstrates what I mean by "How do I find the resistance of a large conductor?"

I've read the Free-Electron Theory of Metals and gone through Paul Drude's structural model of electrical conduction in metals, but I still have not been able to find anything that gets away from the electric field being uniform.

Any hint will be greatly appreciated. I've been reading through my Quantum Mechanics, Physics, and even Electromagnetics books but have not yet seen the path.

It's not a school thing and not a work thing. I'm an EE and is simply something that is driving me crazy. I've talked to professors and other engineers I work with, but most couldn't care less about this issue and are satisfied with treating the conductor as if it is uniformly conducting.

Answer 1

To calculate the resistance of a large piece of metal between to small electrical contacts, you need to solve the electrical potential problem with the given boundary conditions: $$\nabla^2 \Phi =0 \tag 1$$ and then calculate the current from this.

In the metal, the current density $\vec J$ is proportional to the electric field $\vec E$:$$\vec J=\sigma \vec E \tag 2$$ where $\sigma$ is the specific conductivity of the metal. In the stationary case, there are no time changing charges in the metal. Therefore from the continuity equation and $$\vec E=-grad \Phi \tag 3$$ it follows that $$div \vec J=\sigma div \vec E=-\sigma div grad \Phi=0 \tag 4$$ which is just the Laplace equation for the electrical potential (1). Once you have solved the potential problem, you get the current density $\vec J (\vec r)$ from eqs. (2) and (3) and the total current by integrating the current density over a surface $S$ separating the contacts $$I= \int_S \vec J d\vec a$$ Then you'll get the resistance by dividing the potential difference between the contacts $V=\Phi_1-\Phi_2$ by the current $I$ $$R=\frac {V}{I}$$

This is, of course not a simple task. With special symmetries, however, where the potential solution can be obtained readily, it is comparatively easy.

The boundary conditions of the Laplace equation boundary value problem are of the mixed Dirichlet and Neumann type: (1) Potential $\Phi_1$ at contact 1, (2) Potential $\Phi_2$ at contact 2, (3) Normal current density and thus normal electric field zero (i.e. $\frac {\partial \Phi}{\partial n}$ at the boundary surface of the metal piece.