Inverse Square Law of Electric Field

Question

EDIT: The image didn't load.

A NON-conducting cylinder with radius R, charge density $\rho$. And we're focusing in a section away from the edges.

About the electric field in a region $r>R$.

For problem (b) I see that the electric flux is equal to the charge $\frac{q}{\epsilon_0}=\frac{\rho\pi R^2l}{\epsilon_0} $

And the area of a cylindrical sheet is $2\pi r l$.

So the electric field is $E = \frac{\rho \pi R^2 l}{2\epsilon_o\pi r l}=\frac{\rho R^2}{2\epsilon_0r}$.

Confirmed this was the right answer.

But I learned in physics that by Coulomb's law, the electric field produced by a point charge is equal to $\frac{kq}{r^2}$, where $k=\frac{1}{4\pi \epsilon_0}$.

Why is the electric field produced by this cylinder not inversely proportional to the square of distance?

Answer 1

Coulomb's inverse square law holds only for the electric field strength of a point charge. Here you have an infinitely extended charged cylinder. Therefore, the electric field has to be axially symmetric. Its direction is always radial from the axis and there is no component in the direction of the axis.

Thus the Gauss surface is also a cylinder surface around the charged cylinder. Which gives you the $\frac {1}{r}$ dependence of the electric field $E$ in this case, because the area of a cylinder section of height $l$ is $$A=2\pi r l$$ and the enclosed charge is constant.

Answer 2

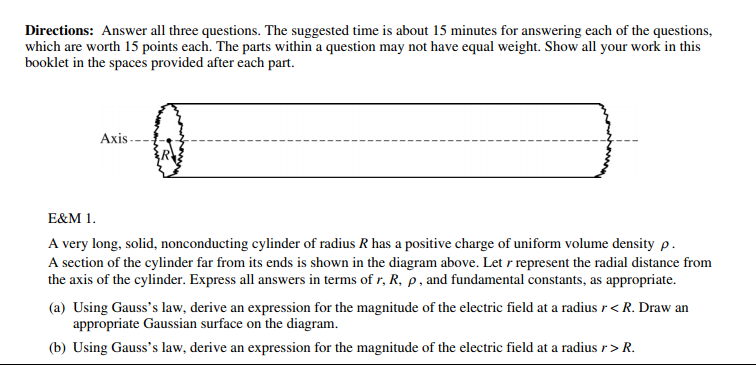
$\newcommand{\vect}[1]{{\bf #1}}$ $\newcommand{\dd}{{\rm d}}$

You are right: Coulomb's law states the electric field of a point charge behaves as $1/r^2$, clearly you do not have a point mass. But it is easy to see why.

Consider a simpler version of the problem, a wire of size $l$ and charge $q$

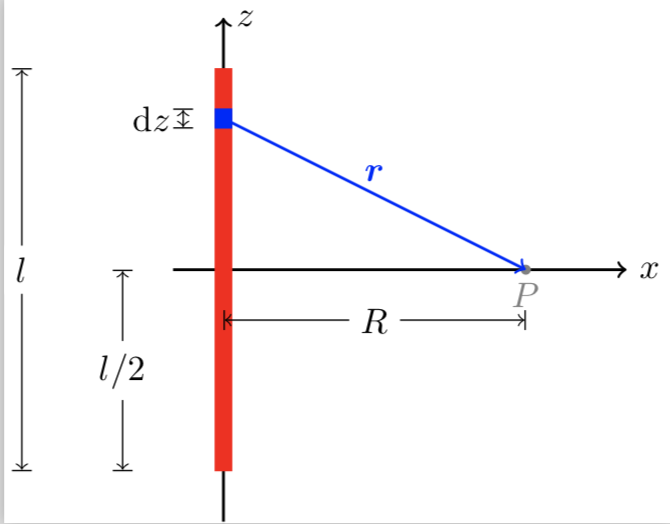
If you take the element of size ${\rm d}z$, small enough to consider it a point, you can apply Coulomb's law to measure the electric field at location $P$, the result is

$$ \dd\vect{E} = \frac{\dd q}{4\pi\epsilon_0}\frac{\hat{r}}{r^2} = \left(q\frac{\dd z}{l}\right)\frac{1}{4\pi\epsilon_0}\frac{R \hat{x} + z\hat{z}}{(R^2 + z^2)^{3/2}} \tag{1} $$

So that the electric field $\vect{E}$ is just

$$ \vect{E} = \int_{-l/2}^{l/2}\dd \vect{E} = \frac{q}{4\pi\epsilon_0 l}\int_{-l/2}^{l/2}\dd z\frac{z}{(R^2 + z^2)^{3/2}}\hat{z} + \frac{qR}{4\pi\epsilon_0 l}\int_{-l/2}^{l/2}\dd z\frac{1}{(R^2 + z^2)^{3/2}}\hat{x} \tag{2} $$

It is easy to calculate both integrals, in particular the first one is zero (odd function on a symmetric interval), so the result is

$$ \vect{E} = \frac{q}{4\pi \epsilon_0 R} \frac{\hat{x}}{(R^2 + l^2/4)^{3/2}} \tag{3} $$

Now take two cases

$l \gg R$: an infinitely large wire

In this case Eq. (3) becomes

$$ \vect{E} = \frac{(q/l)}{2\pi\epsilon_0 R} \hat{x} $$

which is pretty much what you get applying Gauss Law!

$l \ll R$: measuring the field at a large distance

For this case

$$ \vect{E} = \frac{q}{4\pi\epsilon_0 R^2} \hat{x} $$

And that is Coulomb's Law. That is, if you are very far away from the wire, you can consider it to be a point.

Intermediate cases

In an intermediate scenario, the electric field can always be written as a sum of powers of $r^{-k}$, which is the whole idea behind a Multipole Expansion