Question about Speed of Sound

Question

I'm looking over PowerPoint slides of my past Physics lecture and I'm confused about a point my professor made:

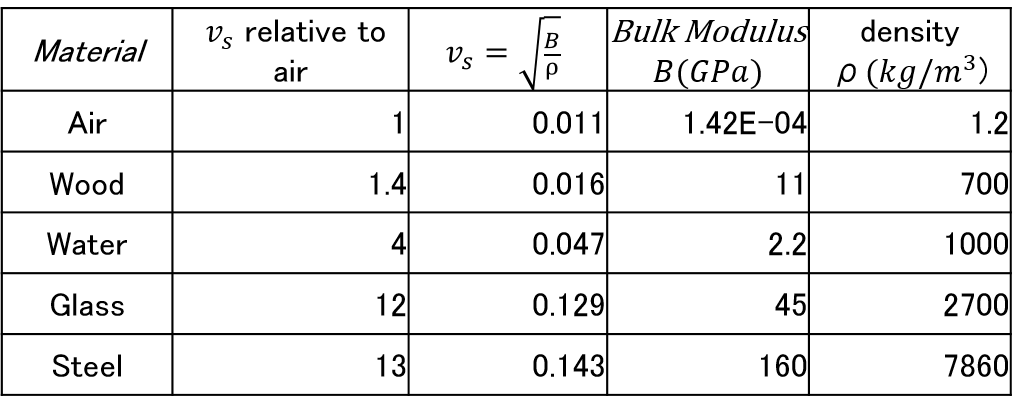
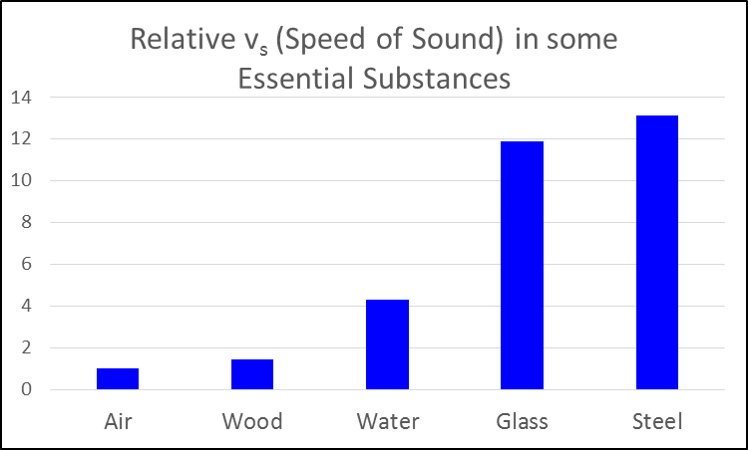
The speed of sound is much higher in a liquid or a solid. It turns out that the speed of sound is given by

$v_s = \sqrt\frac{B}ρ$

where $ρ$ is equal to density, and $B$ is equal to the elastic modulus.

I'm wondering why the speed of sound is higher in a liquid or solid. Solids and liquids are more dense because the volume occupied by their mass is smaller. So shouldn't the denominator in the fraction expression be larger, which means the speed of sound is going to be lower?

I'm wondering where I'm going wrong in my understanding, hoping that someone could clarify this for me.

Answer 1

The equation holds. It is useful to look at the relative speed of sound calculated using the equation in your question. Here is the relative speed of sound for some essential substances:

Answer 2

The speed of sound is higher in solid or liquid substances because in a gas the molecules or atoms have to collide with each other to transfer their momenta and to keep the pressure waves moving. In solids the molecoules or atoms are much closer to each other and they don't even have to collide to transfer their kinetic energy or momenta. In a nutshell, roughly speaking this is the reason why the speed of sound is higher in solids.(The case is similar in liquids.) I hope my answer was useful :)