0
$\begingroup$

I know (and it's easy to proof the formula), that the maximum angle at which an object will stay static on a slope being at an $\alpha$ angle to the ground is

$$\tan \alpha = \mu$$ where $\mu$ is the static COF (coefficient of friction).


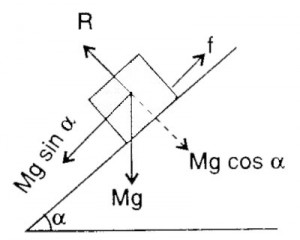
(source: sciencehq.com)

(http://www.sciencehq.com/wp-content/uploads/Angle-of-repose-300x240.jpg)

So it does not depend on an object's mass and a planet's gravitational constant.

But in a science-fiction book of Stanislaw Lem's there is a statement that implies an angle of slope is somehow different on the Moon that on Earth. It's not clear how and the author doesn't give any explanation. However, Lem usually takes care about a "science-corectness" of his texts. So it makes me wondering who/what is right: the formula or Lem? (or in other words: maybe in the 'real world' the angle really differs?)

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ What is the actual statement in the book you refer to? It's difficult to answer without knowing that. $\endgroup$
    – Chris ♦
    Commented Dec 25, 2017 at 15:58
  • $\begingroup$ @Chris The angle $\alpha$ on the Moon should be greater, according to the book. $\endgroup$
    – jacek.ciach
    Commented Dec 25, 2017 at 19:05
  • 1
    $\begingroup$ -1 Not clear what you are asking. What exactly does the author say (not imply)? What explanation does he give? $\endgroup$
    – sammy gerbil
    Commented Dec 27, 2017 at 5:17
  • $\begingroup$ He just says, that the angle is different. And it makes me wonder if this angle is really different or the author made a mistake. $\endgroup$
    – jacek.ciach
    Commented Dec 27, 2017 at 9:23
  • 1
    $\begingroup$ I don't trust them - but it makes me wonder. Is that wrong? $\endgroup$
    – jacek.ciach
    Commented Dec 27, 2017 at 11:15

1 Answer 1

Reset to default
3
$\begingroup$

Short answer:

Lem is probably wrong. It's unlikely there is a relevant difference.

Long answer:

Forces other than Coulomb friction, such as van der Waals and electrostatic might help to keep the block in place. They are small compared to gravity on Earth, but in low gravity they could in principle play an increased role and lead to a higher angle.

Supposing that the angle of repose of granular materials can give us insight on the inclined plane problem, the former offers two advantages: i) due to the greater relevance of the granular problem, we can find enough results in the literature to attempt an answer, and ii) granular systems should average out some of the inconsistencies common to friction experiments.

It turns out that only Kleinhans et al. (2011) Static and dynamic angles of repose in loose granular materials under reduced gravity claims to see a non-negligible effect:

the static angle of repose increases about 5° with reduced gravity, whereas the dynamic angle decreases with about 10°

with most of the remaining research concluding that gravity has from small to negligible effects:

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.