Since light spreads out in all directions from it's source, how far it must travel to become individual photons? Can we consider, that is, that as the intensity of light becomes weaker as it spreads, we measure in fact less photons until only a few remain?
Or another way to look at it: What is the density of photons in a given beam of light or for that matter any electromagnetic wave?
This is the double slit experiment one photon at a time.
. Single-photon camera recording of photons from a double slit illuminated by very weak laser light. Left to right: single frame, superposition of 200, 1’000, and 500’000 frames.
In 2003, A. Weis and R. Wynands at the University of Bonn (Germany) designed a lecture demonstration experiment of single photon interference from a double slit Light from a laser pointer was so strongly attenuated that at each instant there was only a single photon between the double slit and the detector. The diffracted light was recorded by a single photon imaging camera consisting of an image intensifier (multichannel plate, MCP) followed by phosphor screen and a CCD camera.
Looking at the frame on the outer right, where the continuum of the laser pointer classical interference is seen, and dividing by the number in the first frame, you can get an idea of how many photons are involved.
Alternatively using the poynting vector describing a monochromatic light beam , i.e. the energy per second per cm^2 , and dividing by h*nu, where h is the Planck constant and nu the frequency of the classical beam, i.e. the energy of one photon, you will get the number of photons passing that area per second. If you consider it a point source you could use geometry to tell you how far away the number of photons will fall to the number comparable to the first frame on the left.
Attenuation of a beam can easily be made by controlling the energy supplied to the source after all, as in the double slit experiment.
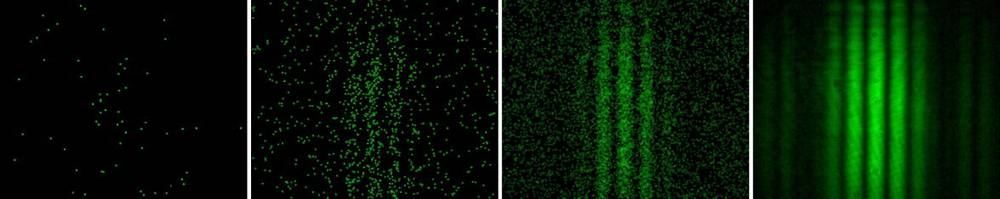
I'll leave it to laser mavens and astronomers to quantify attenuation and size of beams of various energy densities, and invite you to look again at your microwave oven.
Estimate the number of microwave photons produced per second.
If its power is about 1100 W, 400 W is wasted in the magnetrons, so about 700 W is spewed out as microwaves of frequency 2.45 GH (wavelength 12.2 cm).
The number of photons gushing out per second, then, and absorbed by your food (water) and device walls is $$ \frac{700 W/s}{h\nu}=\frac{700 ~J /s}{6.63\cdot 10^{-34} J s\cdot 2.45\cdot 10^9/s}\sim 4\cdot 10^{26}/s . $$ Now, such photons are not quite individually detectable, but, in the cosmological background, they sure shape the Planck formula the way he envisioned it.
That's, of course, about ten orders of magnitude more photons than your radio antenna picks up (FRKT College physics, 22.5).
Likewise what your 635nm, 5mW laser pointer spouts: its photons are detectable by CCD cameras. Specifically, $$ \frac{5\cdot 10^{-3} (J/s) ~~\lambda}{hc}=\frac{5\cdot 10^{-3}(J/s)~\cdot 6\cdot 10^{-7}m}{6.6\cdot 10^{-34}Js\cdot 3\cdot 10^8 m/s}\approx 1.5 \cdot 10^{16}\mathrm{photons}/s ~. $$
How far will Rayleigh scattering take to eat up your photons? Maybe experimenting with your laser pointer in a darkened room might tell you something... The best extinction coefficients for photons are available in astronomy.
