If the answer is the electromagnetic field, then is it also ubiquitously present as space-time?
4 Answers
While it's commonly said (even by physicists!) that gravitational waves are ripples in spacetime this is a bit misleading because it makes them sound like ripples in water, and the obvious question is what is the gravitational equivalent of the water that's doing the rippling?
Mathematically we describe spacetime as a four dimensional manifold (I say describe because I'm not claiming spacetime actually is a four dimensional manifold, only that we can describe it that way - what spacetime actually is we leave to the philosophers). Any point in spacetime can then be labelled by a set of four coordinates $(t, x, y, z)$. Suppose we have some property $P$ that is defined for every point in spacetime, so we can write it as a function $P(t,x,y,z)$. Then we call $P$ a field.
So for example we can have an electric field that varies from place to place and also varies with time, and we'd write it as a function $E(t,x,y,z)$. This is just a function giving the strength and direction of the electric field at that place and time. The electric field is a vector field.
Likewise in general relativity we have a field called the metric tensor that we write as $g(t,x,y,z)$. This is rather more abstract than the electric field because the metric tensor isn't something physical but an abstract object that tells us about the distance between nearby points. But it is just a field - in this case a tensor field.
Both the electric field and the metric obey equations that specify how it changes with time and position, and in both cases there are solutions that vary sinusoidally with time and space and we call those solutions waves. With the electric field this is just the familiar electromagetic wave e.g. light and radio waves. With the metric the oscillating solutions are the gravitational waves.
The key point to understand is that in neither case are these waves like ripples in water or sound waves in air. They are not waves in anything. They are just oscillating values of a field.
So assuming you haven't got bored and given up by now, we can get back to your question:
If the answer is the electromagnetic field, then is it also ubiquitously present as space-time?
And the answer is that yes the electromagnetic field is defined for all points $(t,x,y,z)$ in spacetime. In that sense it is ubiquitously present, but be careful because that just means its value is defined everywhere and that value can be zero.
-
$\begingroup$ John, thank you for your answer. It was in relatively simple and easy to understand terms which gave me a clear idea of what is actually happening. From what you say, it seems that in this case, Mathematics gave way to the wave and field idea and not the other way around. Just curious to know if the wave and field idea was in fact born out of the equations, or if the equations were discovered because of an already existing wave-field idea. $\endgroup$ Commented Oct 30, 2017 at 22:00
-
$\begingroup$ The other question that rises for me is, what physical situation can make the value of an EM field be zero? If we analogically interpret the electromagnetic field as space-time, the only time it occurs to me that space-time can be zero is when there is an absence of it, which maybe is when the question is artificially constrained i.e. in a model or something or before the big bang. In the case of the EM field would it be the same? $\endgroup$ Commented Oct 30, 2017 at 22:44
-
$\begingroup$ @JoseMiguelCruzyCelis: The electromagnetic field isn't spacetime. The EM field has a value at every point in spacetime and that value can be zero. Spacetime is a manifold and doesn't have a value. $\endgroup$ Commented Oct 31, 2017 at 6:54
Ripples is a term describing small waves; "small" is not a very good description of the waves generated in the place where the gravitational collapse happened, but fair enough by the time they were detected in the recent experiments.
All waves classically , whether in water or air or solids , even electromagnetic, are functions of spacetime, because that is the framework we live in. These functions describe the transfer of energy in space time , from one (x,y,z,t) to the next. Gravitation is described by General Relativity and also has this sinusoidal behavior in energy transfers. The only difference being that it is (x,y,z,t) itself that is changing as the energy passes.
Research the last hundred years has discovered that the underlying basis of the classical fields, that also give rise to wave equations transferring energy, is quantum mechanical . Gravitation is not yet described rigorously by quantum mechanics, only effective theories are used. The other three forces, weak,strong, electromagnetic are beautifully fitted with the standard model of particle physics.. This model uses quantum field theory for its calculations, which fit the data beautifully, and can predict new phenomena.
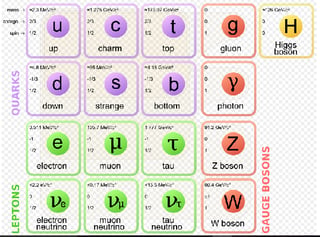
Quantum field theory means that the basic building blocks of the world, are the particles shown here
For each particle there exists a quantum field in space on which the particles travel as distortions of this field, represented by creation and annihilation operators.
So one can say that a traveling electron is a "ripple" in the electron field, and a traveling electromagnetic wave is a "ripple" on the photon field.
Once gravity is definitively quantized , a unified view of how these "ripples" form on the respective fields will become clear in the future.
The only theory that gives glimpses of rigorous quantization of gravity is string theory, and it would be interesting to see what sort of ripples happen when there is one elementary particle, the string, and all the rest, including gravitons, are excitations of it.
The gravitational field is a reflection of the spacetime curvature. Also, according to the equivalence principle, locally the effects of gravitation are equivalent to the effects in an accelerating frame of reference. So gravitation and acceleration are a reflection of very specific properties of spacetime. Please note that this conclusion is a bit different from "ripples in spacetime", because these effects are not just due to spacetime in general, but to very specific properties (symmetries) of spacetime.
It so happens that there are more symmetries than just those responsible for gravitation. For example, there is a symmetry that in the group theory is called $U(1)$. This symmetry also is a very specific property of our universe that on one hand is very simple, but on the other has fundamental consequences. Mathematically $U(1)$ is the unit rotation by the angle $\theta$ in the complex plane expressed as the $e^{i\Theta}$ multiplier. At the quantum level it corresponds to the phase shift of the wavefunction.
When we require this symmetry to be obeyed locally, we look at how it works with the Lorentz transformations of Special Relativity. This reveals a force field of two components (that we call electric and magnetic) interacting with charges in the way described by the Maxwell equations of the electromagnetic field. The Maxwell equations follow directly from applying the local gauge invariance (under the Lorentz transformations) to the $U(1)$ symmetry.
So the answer to your question is that the electromagnetic field (including the waves) is a result of the $U(1)$ summetry (simple $e^{i\Theta}$ unit rotation) that exists in this universe plus the fact that our spacetime generally obeys the Lorents transformations (Special Relativity that defines the speed of light). Ultimately all interactions are a result of different symmetries that exist in our universe.
-
$\begingroup$ 1. "more symmetries than just those responsible for gravitation" What are the "symmetries responsible for gravitation"? 2. A physical theory can conceivably have more than one $\mathrm{U}(1)$-symmetry and, in fact, the Standard Model contains multiples of them (as subgroups of the symmetries of the weak and strong force). There is no "the $\mathrm{U}(1)$ symmetry". 3. Demanding a theory be a gauge theory with a $\mathrm{U}(1)$-symmetry does not lead to the Maxwell equations unless you very specifically demand it to be a Yang-Mills theory coupled to an external conserved current. $\endgroup$– ACuriousMind ♦Commented Oct 28, 2017 at 10:42
-
$\begingroup$ @ACuriousMind 1. (A) Invariance under diffeomorphims (B) Invariance under infinitesimal diff. (C) Invariance under local Lorentz transformations (acting on the tangent space). Note that while the Yang-Mills theory is a gauge theory, not all gauge theories are of the Yang-Mills type. 2. "There is no U(1) symmetry". Then, according to you... the phase of the wavefunction is observable? And birds can't sit on high viotage lines? 3. So what? The fact that the theory under U(1) is interacting comes right from the covariant derivative and the current is conserved per Noether based on U(1). $\endgroup$ Commented Oct 28, 2017 at 13:53
-
$\begingroup$ 1. (A) and (B) are wrong answers, since many theories without gravity are diffeomorphism invariant. (C) is only correct in certain formulations and is not true in the standard second-order formalism of GR in which only the metric is dynamical, cf. this answer of mine. 2. No, please read what I wrote. There is no unique U(1) symmetry, and, once again, the U(1) phase symmetry of quantum state is not the U(1) symmetry of electromagnetism. I won't reply further on this issue because you're simply wrong. $\endgroup$– ACuriousMind ♦Commented Oct 28, 2017 at 14:02
-
$\begingroup$ 3. Your answer claims "the electromagnetic field is a result of the U(1) symmetry". This is wrong, the electromagnetic field or, more precisely, classical electromagnetism is only a "result" of U(1) symmetry if you demand a Y-M theory and an external conserved current that's conserved off-shell, i.e. without Noether. Your claim that U(1) symmetry alone is somehow responsible for electromagnetism or the electromagnetic field is wrong. U(1) symmetry is no more the reason for the electromagnetic field than rotational symmetry is the reason a sphere is round. $\endgroup$– ACuriousMind ♦Commented Oct 28, 2017 at 14:04
-
$\begingroup$ @ACuriousMind Yes, I won't reply on this anymore either, since your comments lack physical intuition and are but mathematical splitting hairs. Too often mathematicians with no physical sense pretend to be physicists while forgetting that math is a model, not reality. Your positions that gravitation is not a gauge theory and that U(1) doesn't cause electromagnetism are well known. Fortunately there is no shortage of better opinions on this forum. Agree to disagree. physics.stackexchange.com/questions/71476/… $\endgroup$ Commented Oct 28, 2017 at 14:28
I am going to give a very simple answer. The expresion using GW as "ripples" in space-time is a little bit confusing since it depends on what you define as space-time. The electromagnetic wave is equivalent. So, let me first introduce some ideas:
- Any interaction is A FIELD.
- Gravity and electromagnetism are FIELDS.
- Electromagnetic waves and gravitational waves are ripples or more correctly little local perturbations (deformations) in these FIELDS, satisfying wave-like equations (that is the origin of waves).
If you define the spacetime with the aid of a metric field, a matrix, then you can say that a small perturbation in this field, with some simplications since gravity is a non-linear field, drives you to derive a wave-like equation for these perturbations. Essentially, something like this: $$g_{\mu\nu}=\eta_{\mu\nu}+\varepsilon h_{\mu\nu}\rightarrow \square^2 h_{\mu\nu}=0$$ where $\square^2$ is the wave operator in spacetime. Indeed, I am keeping out certain subtle details since in the linear approximation, it is not $h_{\mu\nu}$ but $\overline{h}_{\mu\nu}$ (a modification that includes an extra piece in the so-called traceless transverse gauge, but it can be skipped to show the concept). In the electromagnetic field, you have a gauge field $A_\mu$, and when you "excite" it a little bit, you obtain something like $$A_\mu'=A_\mu+\lambda a_\mu$$ It can be showed, with the aid of Maxwell equations and certain gauge selection that the electromagnetic waves arise from this in the same way, so $$\square^2 a_\mu=0$$ From the fundamental viewpoint of the 20th century, fundamental forces are based on (quantum) fields. Perturbations of fields propagate using wave-like equations. Therefore, waves are local and small distortions in "fields". Beyond, the issue from the fundamental viewpoint is if...There is something that transcends the notion of field. I mean, according to general relativity, for instance, space-time IS and can-not be separated from the metric itself and the curvature tensor that defines the Einstein tensor $G_{\mu\nu}$. Space-time is the metric, and the derivatives of the metric defines the curvature and the strength of gravity via the Einstein Field Equations (EFE). Gravitational waves are certain solutions to the EFE. Classically, electromagnetism is based on a gauge field from which, similarly, through derivatives, we get the field strength $F_{\mu\nu}$. Maxwell equations in free-space time imply the existence of electromagnetic waves as special solutions in certain gauge.
In summary, you can observe the following answer to your question:
- Gravitational waves are ripples or tiny deformations in the metric field $g_{\mu\nu}$.
- Electromagnetic waves are ripples or tiny deformations in the gauge field $A_\mu$.
- Chromoelectric and weak fields (W,Z) particles are ripples or tiny deformations in the massive gauge fields $A_\mu^{a}$.
- The Higgs waves/particles are deformations of the Higgs field $H$ around certain vacuum, $h$.
You can even generalize this to matter/substance fields, and you get spinorial equations (Dirac-like equations):
- Matter and substances are described by fields.
- Matter follows spinor-like (Dirac-like, Weyl-like,Majorana-like) equations.
- Matter waves are perturbations in matter fields described by spinors.
Then, the question is what is "a field"? That is much deeper question, since everything that we do know is a field...Literally, and how we define a field (classical or quantum) is to some extent something in our current description of the universe we don't understand completely, but Nature is apparently well described (5% at least) by quantum and/or classical fields. Any theory going beyond space-time or fields will have to generalize our understanding of field theory and space-time!
The fundamental concept of matter (substances) and energy (interactions) is, thus, the field. Perturbations into the fields create forces/substances, following equations that are wave-like for interactions and spinor-like for matter fields. The link between matter and energy is subtle due to mass-energy in special relativity, but it is OK. Difference of the fields are in the nature of the equations...And if you want, as matter is substance made of atoms, what are gauge fields and/or gravitational fields made of? Is there any other more fundamental description of Nature than that based on classical and quantum fields? Are light and space-time substances after-all too?