Does light travel faster if fired in the direction of Earth's rotation as opposed to against it?

Question

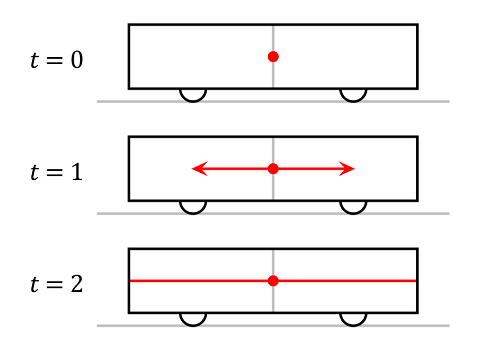
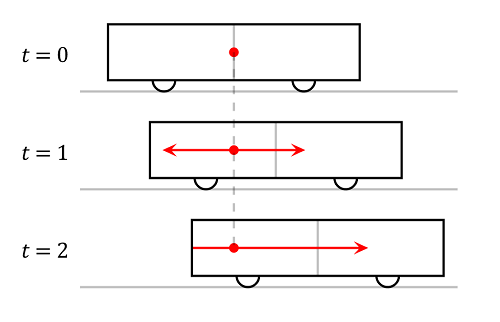
Recently I entertained a silly thought about if the earth's rotation can be "harnessed" to do work. So my question is:

We know that earths rotation is eastward.

If i set up 2 mirrors on 2 different points on earth; one "east" and the other "west" several thousand miles apart.

Then, in the center point between these 2 mirrors, I fire a powerful lazer at the two mirrors.

MirrorA(east) ..................LAZER.................. MirrorB(west)

Will the lazer that hits the MirrorA to east reach faster than the lazer that hits MirrorB? Considering that MirrorA is moving toward the lazer, and MirrorB is moving away from it?

And are there any experiments that show this? I do not know where to look. Google was not very helpful today.

[EDIT #1] Based on comments below, I learn that the field is called special relativity. Note that there are computer sensors etc behind the mirrors, and that I am not the one measuring the light hitting; computers will be doing that job.

[EDIT #2] OK, this will be my final major edit to the question (based on the comments below) to hopefully get the point across, as I do not want this to get too lengthy.

1. We have a lazer that is fired to MirrorA & MirrorB.
2. We know earth spins at about 1000-mph, but just so that I can exaggerate the point, lets say it is moving at 100,000-mps. Also to exaggerate the point, lets say the lazer moves at 10mph (so slow that you can literally see the lazer moving to ward the target).
3. Measurement is done on this wise: when the light hits the mirror. This triggers a mechanical device which walks the distance on the earth toward the gun in the middle that fired the lazer. There is a mechanical device stationed at both mirrors.

Thus, we have MirrorA rushing toward the lazer at 100,000 miles per second. And we have MirrorB rusing away from the lazer at 100,000 miles per second. And we have a lazer traveling at a slow visible 10 miles-per-hour.

100000mps >>>>                  10mph                  100000mps >>>>
MirrorA(east) ..............<---LAZER--->.............. MirrorB(west)

And here is the confusion (based on your comments below). You guys are suggesting, that with:

1. The lazer at constant speed
2. The lazer disconnected from the earth after firing. It is in the air.
3. MirrorA decreasing the distance that the lazer has to travel by 100,000 miles per second.
4. MirrorB increasing the distance that the lazer has to travel by 100,000 miles per second.

You are suggesting that the light will hit both mirrors at the same time. Thus making the mechanical devices coming back to the gun at aprox~ the same time. This is confusing. This is confusing, as one would think that if the distance on one mirror is being shortened, while the other is lengthened, that one would most definitely hit faster than the other. If you and your friend are both walking to 2 cars and the same walking speed. But your car is driving toward you, while your friend's car is driving away from him as he tries to reach it. Then won't you reach your car first?

But i understand that I am not a physics person. So if no one can properly explain it here, or keep posting links to many pages of documents that I have to read through that I will not understand.. then its ok. I will take my time to read your links eventually. I guess I will reach your level sometime in the future. Thanks for all the help thus far.

Answer 1

This is known as relativity of simultaneity and is addressed in Einstein's 1905 special relativity paper. The crux of the problem is that light travels at the same speed in flat spacetime no matter what, and appears so from any inertial frame.

In fact, your situation is essentially identical to the traincar problem.

First, imagine you are inside a moving traincar, directly in the center, and you fire two beams of light in either direction. The invariance of the speed of light in your frame of reference means they will hit the train walls at the same time. This is equivalent to your moving Earth and laser emitter.

Now imagine there is another person sitting on the station witnessing the events happening in the train as it moves by. The invariance of the speed of light in their reference frame means that it will hit the back first and the front later.

In the context of your example, this is equivalent to someone up in space watching the lasers as the Earth rotates in front of them.

Both frames of reference are valid. The invariance of the speed of light has several implications in special relativity, such as time dilation (two different observers experiencing time at a different rate), length contraction, and mass dilation. It's truly some incredible stuff.

You can find the difference in simultaneity using Lorentz transformations.

Answer 2

The experiment is held by Hippolyte Fizeau in 1851 and repeated with better accuracy by Albert A. Michelson and Edward W. Morley in 1886
The result is formalized by Albert Einstein as one of the two Special Relativity axioms (postulates):
Wikipedia → Special Relativity
https://en.wikipedia.org/wiki/Special_relativity

1. The laws of physics are invariant (i.e. identical) in all inertial systems (non-accelerating frames of reference).
2. The speed of light in a vacuum is the same for all observers, regardless of the motion of the light source.

Answer 3

There is an asymmetry in your experiment, which derives from the fact that the earth's surface is rotating, not just moving linearly. Special relativity says that all linear motion is equivalent, but that isn't true of rotational motion.

In particular, suppose the distance to your mirrors equals the circumference of the earth. Then both mirrors (and the laser) are at the same location so checking whether the beams arrive simultaneously is trivial. And they won't: the beam that was fired westward will arrive earlier. This is called the Sagnac effect and it has been detected experimentally at smaller scales. It doesn't violate special relativity, it's a prediction of it.

But if you perform your experiment as stated and have mechanical objects trek back along the path of the light to the source, you'll find that they always arrive at the same time. The reason for this is rather subtle: the region of spacetime in which your experiment takes place has a shape like a piece of paper rolled up into a tube. The tube can be unrolled and flattened without stretching or tearing it, and it then has all the symmetries of the background spacetime, so your experiment is equivalent to one involving linear motion, and it won't be able to detect it because of the principle of relativity.

The Sagnac experiment is conducted in a spacetime region that's more like a tube that's connected all the way around (like a paper towel tube). It can't be unrolled without cutting it, and cutting it would break the experiment. So there's no longer a simple argument that it can't detect the rotation; and in fact it can.

Many other changes to your experiment would "break the flattenability" and make an asymmetry visible. For example, if your walkers headed due north, stopping at the pole, the western one would arrive before the eastern one. If they returned to the starting point but along a different path (for example at a different latitude) that should also be enough to break the symmetry, but I'm not sure what the difference in arrival times would be.

Incidentally, in a Newtonian universe, if you replace the light beams with beams of Newtonian particles and make reasonable assumptions, none of those experiments will see a difference between east and west. Newtonian physics is actually more relativistic than special relativity when it comes to rotation. A Newtonian rotating frame is like an inertial frame with fictitious forces, and the fictitious forces don't affect these experiments as long as the objects are constrained to move along their prescribed paths. In SR a rotating reference frame has "tilted light cones" that can't be simulated by an ordinary force. They do behave like a gravitational force, but gravity is much more different from other forces in relativity than in Newtonian physics.

Answer 4

This is a very old question that has already been addressed, See Michaelson and Morley experiment. Even if there were a difference, what you're suggesting wouldn't work. Besides using earths rotation wouldn't be necessary considering earths orbit around the sun is much faster.

Answer 5

Your question is meaningless until you have explained how you will synchronised the clocks at A and B, which you will need to measure the time of flight from the laser source to A and B. Since synchronisation is just a human convention, there is nothing fundamental you can learn from those times of flight anyway. The only physical measurement you can do, i.e. a measurement free of arbitrary conventions, are the round-trip times from source to mirror A back to source on one hand and from source to mirror B back to source on the other hand. You can then compare those two times. As pointed out in the other answers, you will find they are equal providing the laser source stands at the exact middle point between mirror A and B, which in practice is a long stretch. So you won't find them equal. However, if you record the difference between those times, you will find that it does not vary neither throughout the day, neither throughout the year. Well again providing that your experimental setup is immune to any effect that can change those two distances in a different fashion (thermal dilation, seismic noise, etc), which requires some care.