The first thing to say is that the figures given the manufacturers as well as being informative are there to sell vehicles.
The next thing is that comparison can often be a nightmare because of the mix of units which are used.
For example it depends on your background as to whether you "appreciate" better what 100 horsepower (hp) or 75 kW is.
The specifications that you have given can certainly be used to give you an answer to your question but to make a better comparison more information needs to be provided.
The acceleration of a vehicle $a$ depends on its mass (m) and the force applied on it $F$ via tyre/ground contact $\Rightarrow F = ma$.
The power and torque specifications that you have given are for the engine of the vehicle at particular engine speeds which tend to be maxima.
For the engine power $P$ and torque $\tau$ are linked $P=\tau\, \omega\,$ where $\omega$ is the angular speed of the engine.
Please note that I have figures for the petrol version of the Ford and I have quoted figures for the diesel version later.
For the two vehicles in question the power and torque characteristics look something like this.
I have redrawn the graphs from my primary sources because of mixed units eg foot-pound and newton-metre for the torque and horsepower and kilowatt for the power.
Another problem is that these vehicles have different specifications for different engines and different years so what you see is a rough sketch.
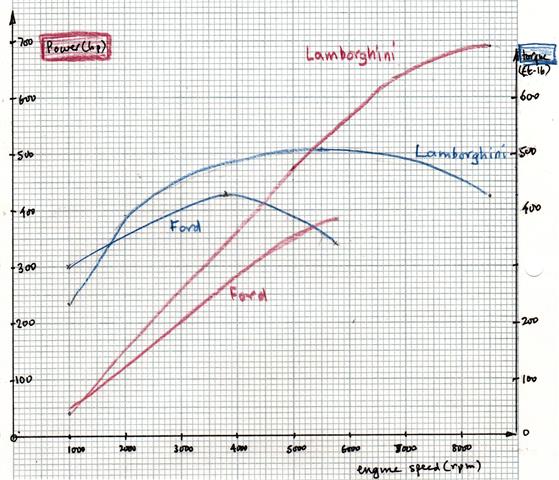
You will note that the Lamborghini engine has a higher maximum engine speed.
As I stated before the mass of the vehicles is a contributory factor to their acceleration and as the Lamborghini (1690 kg) has a mass which is much less than than of the Ford (2745 kg - note the difference in mass from that given by the OP which was the gross value) for a given force the Lamborghini's acceleration will be much larger.
However the torque delivered by the engines changes as one progresses through the drive chain to the wheels.
One must first look at the gear ratios (which "decrease" the speed of revolution and "increase" the torque. then at the transmission ration.
To get the maximum torque delivered to the wheels you need the maximum possible gear ratio.
The Lamborghini has 7 forward gears with ratios
1 - 3.91:1 / 2 - 2.44:1 / 3 - 1.81:1 / 4 - 1.46:1 / 5 - 1.19:1 / 6 - 0.97:1 / 7 - 0.84:1
and a differential ratio of 3.54:1 so when the Lamborghini is in first gear all the torque shown in the graph have to be multiples by $3.91 \times 3.54 \approx 13.8$ assuming that there are losses in the gear trains.
The Ford has 6 forward gears with ratios
1 - 3.97:1 / 2 - 2.31:1 / 3 - 1.51:1 / 4 - 1.14:1 / 5 - 0.85:1 / 6 - 0.67:1
and a differential ratio of 3.73:1 so when the Ford is in first gear all the torque shown in the graph have to be multiples by $3.97 \times 3.73 \approx 14.8$ again assuming that there are losses in the gear trains.
This is slightly higher than that of the Lamborghini.
The turbo version of the Ford has a maximum torque of 925 ft-lb at 1800 rpm and has a gear ratio of 4.17:1 in first gear and can have a differential ratio of 4.10:1 which means that the torque delivered at the wheel is much larger.
There is a last component to consider and that is the radius of the wheels $R$ because
torque at wheels = accelerating force $\times$ radius of wheels
The Lamborghini is a four wheel drive vehicle and has front tyres 335/30YR20 and rear tyres 255/35YR19
Again we have mixed units as the first number is the width of the tyre in millimetres, the second number is the "height" of the tyre as a percentage of the width and the third number is the diameter of the tyre in inches.
So this works out as a radius of the wheels: front - 14 inches and rear - 13 inches.
For the Ford with tyre designation 265/75R16 the radius is 15.8 inches which represents a larger decrease in the force for a given torque.
Taking all this into account the Lamborghini wins hands down primarily because it is much lighter than the Ford and even though the diesel version delivers more torque.
Once the vehicles are moving drag forces play a part and the drag force is using assumed to be proportional to the square of the speed and is given by $F_{\rm drag} = \frac 12 c_D A \rho v^2$ where $\rho$ is the density of the air, $A$ is the frontal area of the vehicle and $v$ is the speed of the vehicle.
The drag coefficient $c_D$ is given by the manufacturers as 0.23 for the Lamborghini and 0.4 for the Ford.
As an aside note that a Formula One car can have a drag coefficient of over one because of the regulation regarding exposed tyres and the necessity to have a down force.
The Ford also loses out in terms of frontal area $8\,\rm m^2$ as opposed to the Lamborghini's $2.3\,\rm m^2$.
At the maximum speed for the Lamborghini or the Ford the power delivered by the engine is equal to the rate of working against the frictional forces.
So as it delivers more power, has a smaller drag coefficient and frontal area you would expect the Lamborghini (217 mph) to have a much greater top speed although one Ford was modified to get close.
The real difference between the two vehicles is their usage.
The Ford needs torque to move heavy loads and does this by adjusting the gear train to magnify the engine torque but as the engine has a relatively low maximum speed the gearing is spaced out so that a reasonable top speed can been achieved in top gear.
Because the Lamborghini is relatively light torque is not a major consideration but there is a need for speed so the gearing is arranged so that the engine speed does not change much and is always close to delivering maximum power.
If you look at the specification of the Lamborghini over the years you will note that the maximum torque does not increase by very much and but the maximum power is increased by making the maximum engine speed higher.

