6
$\begingroup$

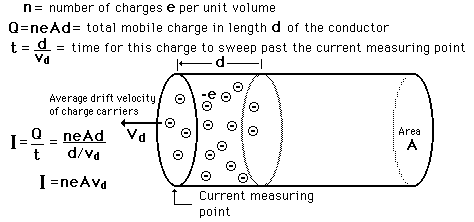
I've always assumed AC to be just another kind of current. However, I've recently heard this statement:

There is no real current in AC, and we use the concept of current only to make understanding of AC easier.

Is this statement true? Why or why not?

The only thing similar I've seen in physics is the concept of centrifugal force (a force which doesn't exist but we imagine to help with our understanding). Could this be something similar? I've found nothing on Wikipedia.

Improve this question
$\endgroup$
7
  • 4
    $\begingroup$ "There is no real current in AC" - do loudspeakers not produce real sound? Do induction motors not produce real mechanical power? $\endgroup$
    – Alfred Centauri
    Commented Aug 12, 2017 at 12:19
  • 1
    $\begingroup$ And does an AC-powered space heater not produce "real" heat? $\endgroup$
    – Jamie Hanrahan
    Commented Aug 12, 2017 at 15:56
  • $\begingroup$ It depends on what the author means by the word real. By any conventional definition of the word real, the statement is just false. $\endgroup$
    – garyp
    Commented Aug 12, 2017 at 16:53
  • 1
    $\begingroup$ Also depends on what the current is composed of. There's no net movement of electrons, as in DC current, so there's no electron current. But there is a current of energy. $\endgroup$
    – jamesqf
    Commented Aug 12, 2017 at 17:03
  • $\begingroup$ Ponder for a moment a swimming pool with a wave machine. when the machine is running you could say "the water is not moving, it stays in the pool," with just as much accuracy, for exactly the same reasons. (all the while being knocked to and fro) $\endgroup$
    – hildred
    Commented Aug 12, 2017 at 19:39

3 Answers 3

Reset to default
13
$\begingroup$

Is this statement true?

Nope.

If not, why not?

Simply because there is a current. Charges do move in AC.

What this statement might have tried to convey (in my opinion) is that in AC, charges do not cover large distances as they move. AC is an oscillating current rapidly changing its direction many times a second. What this means is that the charges in the wire carrying AC keep moving to and fro. So, although they do move and may do work in the process (such as power a light bulb), the mean value of the current remains zero, i.e. $$\lim_{t\to \infty}\frac{\int_0^t I\ dt}{\int_0^t dt} = 0 $$

So, I think by 'real current', this statement tries to say 'steady current'. Of course there is no steady current in AC. However, if the Root Mean Square value of the actual alternating current is made to flow as steady current in another wire of same resistance, it will dissipate the same power as the actual AC in the original wire. $$\sqrt{\langle I^2\rangle} = \lim_{t\to \infty} \sqrt{ \frac{\int_0^t I^2 \ dt} {\int_0^t dt}} = \frac{I_0}{\sqrt 2}$$ (second equality holds in case of sinusoidal current, where $I_0$ is the maximum value of current)

It is this RMS value that the statement might be referring to.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I agree, the statement is bizzare and that the author of the original statement may well be getting at a notion of steady, or average current. Another point is that it might be more accurate to say that a steady current of the given by the RMS value of an AC current dissipates the same power as the AC current when the two currents flow through the same resistance. Other effects can be quite different: there is no steady current equivalent of reactance, for example. $\endgroup$
    – Selene Routley
    Commented Aug 12, 2017 at 10:04
5
$\begingroup$

This is the microscopic view of the current in a conductor:

current

It is a semiclassical one, treating the electrons as localized

The electrons have a small drift velocity, within the conductor. If it is DC they move in one direction, if it is AC they oscillate on an average point, depending on the frequency of the change of field that attracts them. The potential difference moves this effective point giving an effective current which moves with the velocity of light .

Please see my answer here for the more complicated quantum mechanical real situation.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ what do you mean by "The potential difference moves this effective point giving an effective current which moves with the velocity of light"? the current does most certainly not move at the speed of light... $\endgroup$
    – AccidentalFourierTransform
    Commented Aug 12, 2017 at 17:13
  • $\begingroup$ Thanks for your answer, I've accepted it. The person who made the statement agreed that the point behind the statement was the one you mentioned (in AC, the electrons oscillate about a mean position) Thanks again (+1)! $\endgroup$
    – Myungjin Hyun
    Commented Sep 22, 2017 at 12:48
-1
$\begingroup$

AC stands for Alternating Current. That means that there is current, but it's changing its direction periodically (in 50Hz-Europe 1/100th second forward, then 1/100th second backwards).

Improve this answer
$\endgroup$
1
  • $\begingroup$ "AC" doesn't mean "the frequency is whatever your country uses for its electricity distribution network". For example the alternating current in your microwave oven changes direction a few billion times every second, not 50 or 60. $\endgroup$
    – alephzero
    Commented Aug 12, 2017 at 22:36

Not the answer you're looking for? Browse other questions tagged or ask your own question.