Chladni figures: avoided crossings of nodal lines

Question

As khown, Chladni figures display nodal lines of eigenfunctions satisfying the equation $\Delta^2\psi=k^4\psi$ with appropriate boundary conditions. One can note these lines don't like to cross each other:

H.-J. Stöckmann in "Quantum chaos. An introduction", 1999 writes (page 17):

The interpretation of Chladni figures of irregularly shaped plates is thus intimately connected with the quantum mechanics of chaotic billiards. $\langle\ldots\rangle$ Figure 2.2(a) shows one of the nodal patterns for a circular plate observed by a Chladni himself. We find a regular net of intersecting circles and straight lines typical for integrable systems. The central mounting does not disturb the integrability since the rotational invariance is not broken.

The situation is different for rectangular plates (see Fig. 2.2(b)). Here the mounting reduces the symmetry, and the billiard becomes pseudointegrable $\langle\ldots\rangle$.

Figure 2.2(c) finally displays a Chladni figure for a nonintegrable plate in the shape of a quarter Sinai billiard.

M.C. Gutzwiller in "Chaos in Classical and Quantum Mechanics", 1990 writes a similar thing (page 234):

A theorem by Uhlenbeck (1976) states that it is a generic property of eigenfunctions to have non-intersecting nodal lines.

So we see the nodal lines usually avoid to cross. The crossing nodal lines is an exceptional situation which requires some additional conditions (e.g. integrability of the billiard) and can easily be destroyed by perturbations.

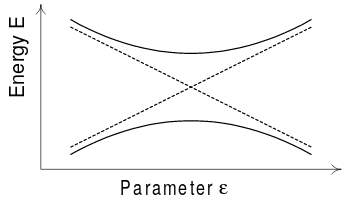
QUESTION: is there any mathematical of physical analogy between anticrossing of nodal lines and anticrossing of energy level in quantum systems? When we change parameters of a Hamiltonian, energy levels cross in integrable systems and repulse in chaotic ones - just the same behavior is demonstrated by the nodal lines, although in coordinate space. Can this analogy be somehow described quantitatively, or it is just a coincidence?

Answer 1

Yes.

The essential idea is that in the seemingly random chaotic systems, the coincidences that correspond to nodal lines crossings or energy levels crossings are typically unlikely.

Both effects, thus, result from the irregularity of chaos and arise in stochastic descriptions of chaotic billiards:

• wave functions modeled by the Random Wave Model (RWM); and

• spectra described by the statistics of Random Matrix Theory (RMT).

Chladni figures

Expecting the ergodic properties of the chaotic orbits to be reflected in their wave counterparts in non-integrable billiards, it's conjectured [Berry, 1977] that the corresponding Chladni figures can be simulated by an appropriate Gaussian random wave ensemble (the RWM).

In this stochastic description, the non-generic nature of nodal crossings becomes especially relevant, as Uhlenbeck's theorem implies crossings appear with vanishing probability:

as the point where two nodal lines intersect, not only does the wave function vanish, $\phi(x,y)=0$, but so does its gradient because the partial derivatives are zero along each nodal line. Therefore, three simultaneous conditions have to be imposed on $\phi$ at the nodal intersection; but whereas a single condition defines a line in the $(x,y)$ plane, and a double condition defines an isolated point, a triple condition cannot be satisfied in general.

Which is Gutzwiller (your reference) very clear description of the gist of Uhlenbeck's theorem.

Recent reviews are Jain & Samajdar's (2017) Nodal portraits of quantum billiards: Domains, lines, and statistics and Nonnenmacher's (2010) Anatomy of quantum chaotic eigenstates (eprint, arXiv).

Energy levels repulsion

In contrast to regular systems, many chaotic quantum systems don't present closely spaced energy levels, compared to the mean spacing. And that remains true even as system parameters are varied, leading to the so-called energy level repulsion.

According to the Bohigas–Giannoni–Schmit conjecture$^1$, this distribution of level spacings can be understood in the framework of Random Matrix Theory, which posits that complicated (quantum) systems can be described by matrices of random elements (respecting a given symmetry).

Copying from Michael Cross Introduction to Chaos notes, Chapter 29 (pdf):

This can be understood in terms of the well known phenomenon of level repulsion: two energy levels that appear to be going to cross as a parameter is varied appear to repel one another, so that no crossing occurs. This is easily motivated by the $2\times 2$ case: $$ H = \left[ \begin{matrix} \epsilon & \delta \\ \delta & -\epsilon \end{matrix} \right] $$ where energy level crossing might be expected for $\epsilon=0$. In fact (see figure above) the energy levels are $E = \pm \sqrt{\epsilon^2+\delta^2}$ so that for non-zero $\delta$ the levels stay apart by the distance $\delta$, and for crossing both $\epsilon$ and $\delta$ have to be zero, with corresponding reduced probability for random matrix elements.

So again, as for Uhlenbeck's theorem, in the stochastic description it becomes unlikely that the conditions necessary for a crossing (here of energy levels) are all simultaneously satisfied.

$^1$ _{The BGS conjecture has been ofered a semi-classical explanation.}