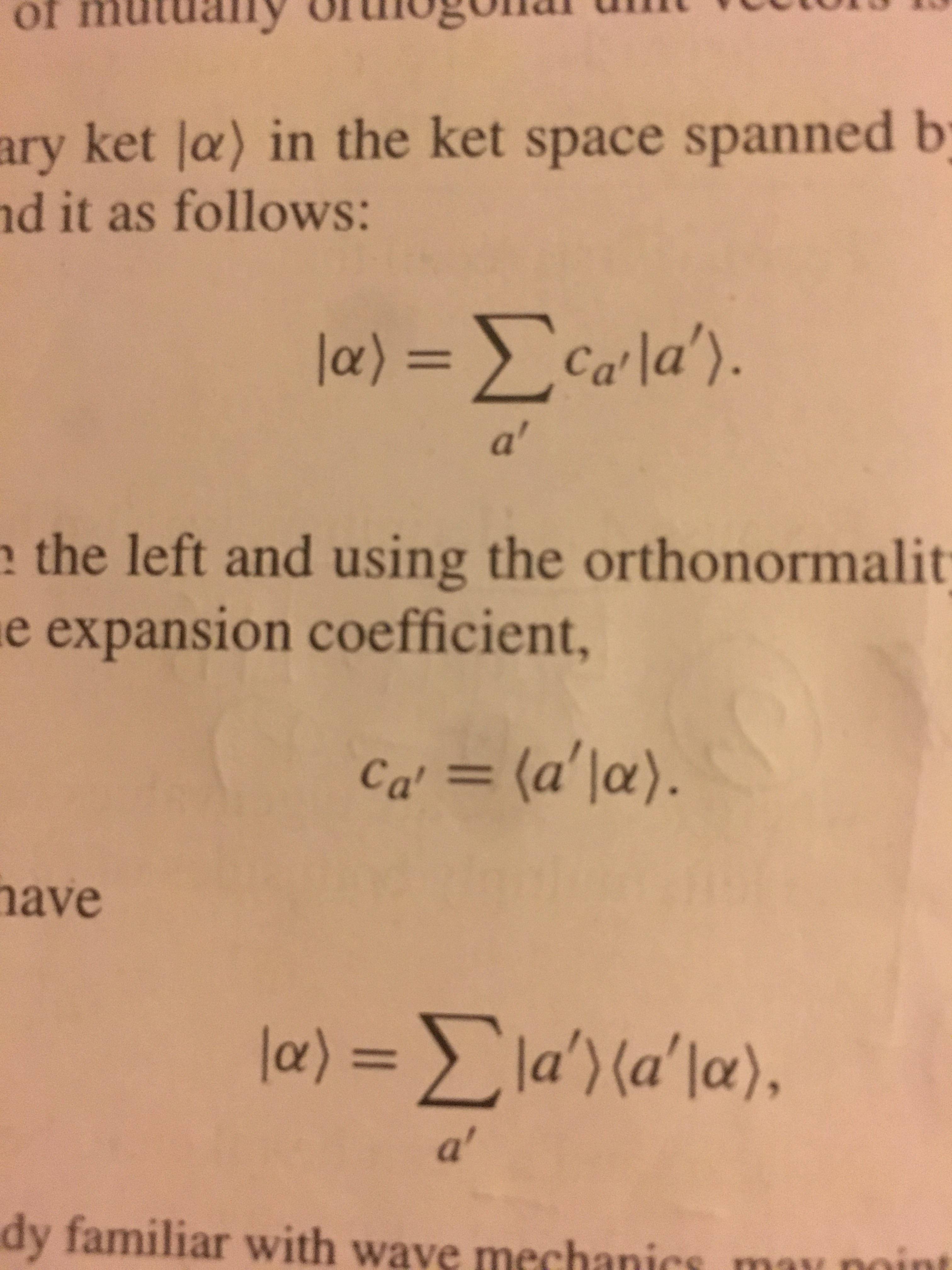
How is the second equation derived with the orthonormality property?
The orthonormality property is
< a"|a'> = kronecker delta_a",a'
I ask because I don't know why the summation in the first equation disappears in the second equation, especially since I could imagine a' and a" being the same while varying.
The second equation $c_{a'} = <a'|\alpha>$ says "the coefficient of $|\alpha>$ corresponding to eigenstate $|a'>$ is computed by projecting $|\alpha>$ onto $|a'>$". Think of it in the scope of linear algebra, where a vector in n-dimentional space is decomposed over a given orthonormal basis. The orthonormality of the basis is required to make this formula valid: for any given basis element $|a>$, $$ <a|\alpha> = <a|\sum_{a'} c_{a'}|a'> = \sum_{a'} <a|a'><a'|\alpha> = \sum_{a'}\delta_a^{a'} <a'|\alpha> = <a|\alpha>$$. So, the summation does not disappear from a formula; it's just an expression for a single coefficient, while the formula for $|\alpha>$ (third one on your page) still has the summation.
I think the confusion comes from the fact that the $a'$ variables in the two lines mean different things - it's a dummy index in the first line but not in the second line. Change the dummy summation index in the first line from $a'$ to $a''$, then hit it with $\langle a'|$, and you should be able to get it.
