From the speed of light being an invariant to being the maximum possible speed

Question

As I understand it, the special theory of relativity is based on two principles - that there is no preferred inertial frame (which is common sensical once we realize that all motion is relative motion) and that the speed of light has the same value when measured in any inertial frame (which is implied by Maxwell's equations and can be verified experimentally).

To reconcile these two principles, Einstein postulated that measured values of length-intervals and time-intervals between two events be dependent on the frame of reference and thus he arrived at the Lorentz transformations.

Now, the formula for the Lorentz transformations forbids any speed higher than the speed of light in order to keep the intervals "real" and therefore light must be the maximum possible speed.

So my question is: Is this (light having maximum speed) an implication only of the kind of transformations involved in STR or is there some general physical principle that would be violated if we have a body traveling at a higher speed than light? Is some thought experiment possible to argue that if the speed of light is invariant then it must also be the maximum speed?

Answer 1

I suggest you work on the first two problems in Chapter 11 in Jackson's book. Basically, the homogeneity and the isotropy of the spacetime, the equivalence of the inertial frames and the requirement that the coordinate transformations form a group together require that the coordinate transformation take the form of a Lorentz transformation with the light speed $c$ replaced by a constant speed $v_c$, which is also the speed limit. Have fun!

Answer 2

I didn't see this when you first posted it but I have three standard mental tools to understand why "invariant" means "maximum possible speed" that I wanted to share with you.

Race a light pulse

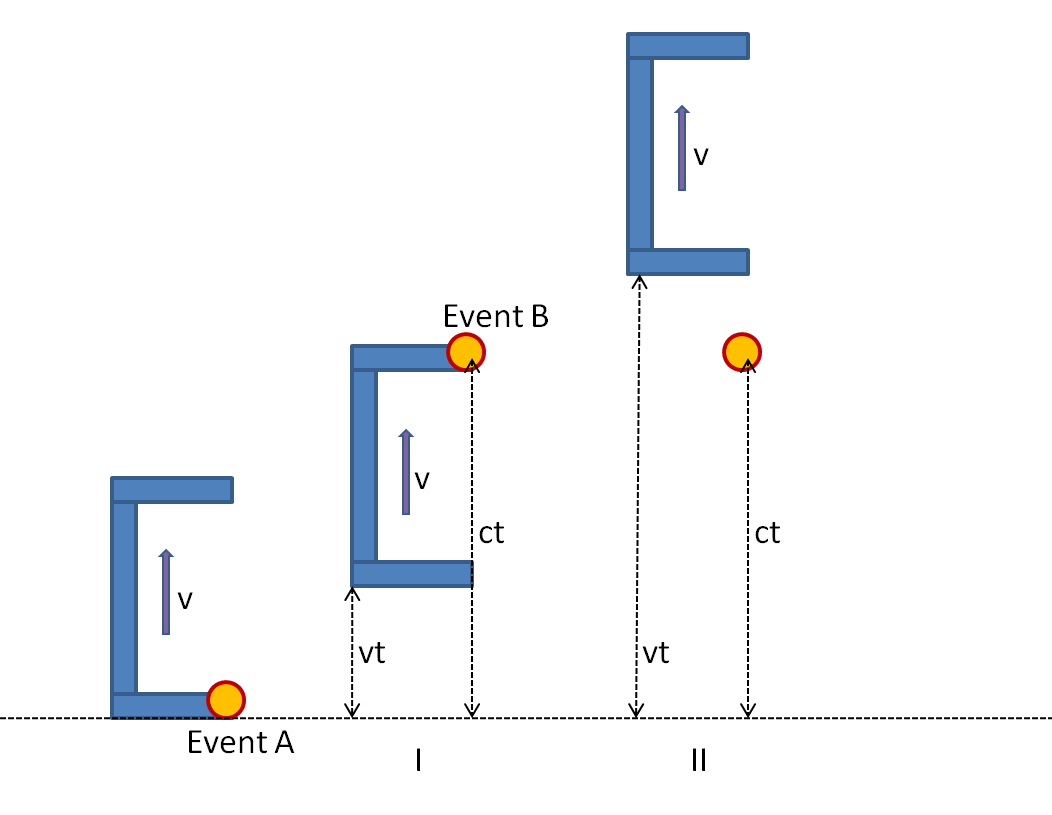
Suppose you want to race a light pulse and beat it, proving that it's not the maximum possible speed. We set up a track for you to fly your spaceship on, out in space, and I scatter some sparse dust uniformly next to that track, for the laser pulse. (The dust will reflect little bits of the light so that we can see where the heck it is, the laser pulse will however be very strong so that this does not attenuate it too far beyond detection.)

What follows is actually a real-life Zeno paradox. Let's suppose that right after we start, you accelerate to $c/2$ relative to me, and try to figure out how fast this light is moving away from you. What answer will you get? Well, if it's truly invariant, you'll find that it's still moving away from you at speed $c$. Frustrated, you drop a reflector and accelerate to $c/2$ relative to that reflector, and see if you've caught up yet: no, it's still moving away from you at speed $c$. So you drop another reflector and accelerate to $c/2$ relative to that. It's still moving away at $c$: you cannot win! Unlike with Zeno's real paradoxes, the "distance you need to travel" (really, the velocity change you need to effect by accelerating) is not actually decreasing as you travel halfway to where you're going.

Just call her, already!

The above technically proves that continuous accelerations can't go faster than light but we might be more interested in the idea that information can't be broadcast faster than light. To easily understand this, consider two consequences of relativity: (1) that everyone has valid laws of physics related to moving reference frames by these Lorentz transformations, and (2) that these Lorentz transformations predict that moving clocks run in slow motion.

Alice is in a spaceship moving relative to Bob, so Bob sees Alice's clocks ticking in slow motion. But Alice also sees Bob's clocks ticking in slow motion. This is a frustrating situation! You want to say: whose clocks really are ticking slow, here?! I like to imagine that I get frustrated with this situation and say to Bob, "Just call her up and one of you will be talking fast and one of you will be talking slow and we will both know which one of you is talking slowly!"

Well, not so fast. My intuition above is treating phone conversations more or less the way they work in my conventional experience with my friends, where the communication is instantaneous between us both. But how is Bob going to call Alice up--with a cell phone? How do those work? Microwaves, which are light waves with a wavelength about the size of a hand or so. So those bits of conversation transfer at the speed of light! But that means that between whenever Bob says something and when Alice gets it, there will be a transmission time gap between those two events. That time gap will swallow up any ability to detect who is talking slow.

So we have plainly seen that instantaneous communication breaks the equivalence postulate, so some limit of faster-than-light communication in general probably lets us figure out objectively who is talking slow vs. who is talking fast. But we can preserve this equivalence of all reference frames simply by stating that no information moves faster than $c$.

Bubbles and time-travel.

I've talked a few times about expanding light bubbles on this site, e.g. here, it's just a way of talking about what in relativity is more formally called a "future-pointing light cone." The idea is that when some sudden event happens, light rushes out in all directions at speed $c$ to notify everyone about this event that's happened: that structure of expanding-at-$c$ bubbles of light (they're thin because the events are instantaneous) is one way of thinking about what relativity is all about.

The Lorentz transforms that relativity allows maps all expanding bubbles to other expanding bubbles, but it might grow or shrink different bubbles differently. However, Lorentz transforms will always respect the bubbles' topology: if one light-bubble is contained within another light-bubble then if I shrink the outer one I cannot grow the inner one until they collide; the one must remain topologically inside the other one. Similarly if two expanding bubbles intersect on a circle, I cannot do anything to put one of them wholly inside the other one; as they shrink smaller and smaller they must become disconnected bubbles.

The first topology is called a "timelike separation" of the two events; the second topology is called a "spacelike separation" of the two events. (There is one other option, where one of them is inside the other one but they both share exactly one point on the surface of the sphere, and this is called a "null separation" of the two events, it is just on the border between these, where if the inner one were just a little bigger they'd intersect on a circle and if it were just a little smaller they'd not intersect at all.)

Importantly, if two events are timelike-separated then there is no objective space separation; there is some allowed Lorentz transform such that both light bubbles are centered on the same point and these reference frames think that both events happened at the same place. Similarly if they are spacelike-separated then there is no objective time separation; there is a reference frame which scales both bubbles to the same size and therefore thinks that in the past they both were shrunk down to points at the same time.

Once you can appreciate these you can see that if you can do arbitrary ad-hoc faster-than-light travel as well as arbitrary slower-than-light travel, you can travel backwards in time too.

It is very simple: Consider some event in your past, you are stuck in this expanding bubble of light. Well, you're not stuck: you can travel faster than light to eventually break through the bubble and get outside of it. Now do something outside that bubble, and you'll find yourself in a new bubble, one that is spacelike-separated from the other bubble. Boosting into one of the other normal reference frames, you can shrink the original event from your past down to a point while the bubble you're in grows very large. If you do it right, you can now travel faster-than-light out of your bubble and set up a new bubble which will contain that bubble from the event in your past, proving that you have time-traveled with just two faster-than-light jumps in different directions. Evidently your starting reference frame must view this second jump as a timetravel backwards in time; and in fact it must be a theorem that all faster-than-light travel of information looks to someone like the information travels backwards in time, though you do need to be able to travel superluminally in two different reference frames in order for this to permit provable time-travel.

Answer 3

This is an example of what immutable physical property "light speed" might have, which would have to be violated if a body moved faster than light. It draws upon some "fringe" physics of my own, but I don't think that detracts from its answering your question in so far as it demonstrates what that physical property would have to be.

If we say that everything is effectively moving at the speed of light, and when we perceive it to be stationary this is because it is travelling parallel to us and when we perceive it to be moving at light speed this is because it is travelling orthogonal to us, we can rewrite the same predictions of relativity with a new maths in which only directions change.

Fascinatingly this predicts the rotation group $SO(3)$ would describe transformations, which is exactly what relativity says.

This begs the question; what, in this model is the "vector" component of speed, namely that "we perceive speed to have direction and magnitude, how can it only have direction?" Well this is down to the degree to which an object's internal motion is parallel. If all of its components are in parallel, from some observer's point of view, then it is moving at light speed relative to that observer and all of its energy is in its motion. On the other hand the more its internal components are moving relative to themselves, the less parallel they are and the slower it may move as a whole and the more energy it contains internally.

Since a body's internal motion is its ageing process, time dilation follows too.

All this, of course, is the same universe described by Einstein and will help to understand why light speed's invariance is locked in to its inexceedability, but it is a different abstraction and one which won't necessarily be easy to reconcile with Einstein's equations.

Answer 4

You are correct. Relativity itself does not prohibit motions faster than $c$. What it does say is that there are essentially three disconnected domains of speed : $v < c$, subluminal speed, corrresponding to timelike paths in spacetime, $v = c$, luminal speed, corresponding to lightlike paths in spacetime, and $v > c$, superluminal speed, corresponding to spacelike paths in spacetime. They are "disconnected" in the sense that it is impossible to accelerate an object from one domain to the other. In particular, one cannot force a particle that moves with $v < c$ to reach $c$ by speeding up: the speed instead "plateaus" near $c$. This is one sense in which you could interpret $c$ as a "maximum" speed. But relativity doesn't strictly say that $v > c$ is impossible. Theoretically, a particle could exist in any of the three domains: it just can't cross between them. Which one it occupies is determined by its mass - a positive real mass makes it subluminal, a zero mass, luminal, and an imaginary mass, superluminal.

But despite this, we do say $c$ is the maximum absolutely, that is, the $v > c$ domain is unoccupied. Why? The answer to this can be thought of as follows. Due to the "relativity of simultaneity", points in spacetime far enough apart they could only be reached by a motion with $v > c$ do not have well-defined temporal ordering: a reference frame change can switch the order. This means that a particle moving with $v > c$ in one frame can be seen to move backwards in time in another. By cleverly arranging two such particles, it is possible to exploit this to send a message into one's own unambiguous past (past light cone). This creates a "time causality paradox" of the type that is often played with on Sci-Fi movies, like the "grandfather paradox". A non-violent form of the paradox is you just send yourself a message telling your past self to not send a message. Because of this paradoxical situation, it seems to challenge the consistency of the universe and thus suggests it is doubtful there should be anything with $v > c$. Moreover, quantum field theories tend to reinterpret the "imaginary mass" associated with the $v > c$ domain to be "maximally unstable" particles (in particular, unstable particles are particles with complex masses, and the more the complex mass favors the imaginary part, the more ephemeral the particle, with pure imaginary mass we have no particle at all) which creates an effect called "tachyonic condensation" and ends up with only real mass, thus $v \le c$, particles existing. But even with these two caveats, clever theorists have suggested ways they could be gotten around, e.g. "self-consistency" rules and "non-canonical kinetic terms".

So then the real, genuine, and most honest answer to why this is is that it is what we have observed. The paradoxes are easily resolved by simply leaving the domain $v > c$ unpopulated, and so far, it appears this is how the natural universe actually does it, and indeed the use of quantum fields that burn out with imaginary mass might be considered a more detailed exposition of how this choice is implemented. Of course, we could be wrong, but that's what our current evidence base says. There are no particles we have ever observed which belong to the $v > c$ domain, and as mentioned above relativity forbids acceleration between domains and this too is well borne-out by our particle accelerators which can bring particles extraordinarily close to $c$, but never surpassing it (if they did, it would need to be taken into account in accelerator designs. All accelerators are designed using Einstein's theories and have run into no hiccups of this type, so they look very good.). It is unfortunate, perhaps, if we want to travel to the stars, but the upshot is that biology is considerably more malleable than the laws of physics, and so perhaps the real way to the stars is not through trying to get around the $c$ limit, but instead get around the natural death limit, whether through genetic engineering, or through brain-computer merger, or any of a number of other such "transhumanism" approaches.

Cheers :)

Answer 5

Working on @mike4ty4's answer, I think I figured out a simple thought experiment to demonstrate that if there is an invariant velocity it must also be the maximum velocity (if causality is assumed to hold true).

Suppose, in an inertial frame a person switches on a light pulse (event A) and makes it fall a little distance away (event B).

For any other inertial observer moving in the same direction as the light pulse with velocity, $v < c$, the sequence of events would look like as shown in part (I) because the relative velocity of the torch operator is now $v$.

But if the velocity was greater than $c$, he would never see the pulse of light hitting the other end at all! Because now the torch operator is moving relatively faster than the speed of light. In fact, for this superluminal observer, event B would take place before event A!

And the notion of causality is, I think, strong enough that anything that seems to go against it must be rejected as wrong.

Answer 6

A simple thought experiment does the trick -- consider a train moving faster than light, and it has headlights (it's a glass train). According to a stationery observer (stationery in a reference frame where the train is faster than light), the train must always be in front of the light, but according to an observer hanging out of the train, the light must be in front of him, since light speed is still $c$.

It might not seem like this relativeness of the order of the two objects is a problem, but it is -- say, for instance, the train is moving towards a high-tech wall which is trained to do this when switched ON: (1) if hit by a train, make world explode (2) if light is incident, switch OFF. The wall is currently switched ON. According to one observer, the world explodes, whereas according to another, it doesn't. This is an inconsistency.

Why wouldn't this argument apply to any speed and prohibit all motion? For example, why can't the wall be programmed to switch off a certain amount of time after which light is incident? Relativity says this is okay, because time can dilate and transform scale between reference frames.

But in order to make FTL speeds okay, you need to allow time to flip direction -- this is why the real condition is "to go faster than light, you must forgo causality", or simply, "locality = causality".