I know the ‘Twin Paradox has been done to death, and I think I understood every answer as given. But I have NEVER seen any answer the question I have. This question is about THE REAL paradox of relativistic time dilation:
Assume: Two spaceships (A and B) are in the vast blackness of space. They pass each other going in opposite directions at 80% of the speed of light (relative to each other of course. These ships are equipped with Bluetooth like clocks that, that transmit their time continuously, while monitoring the receive. When they receive a signal from another transmitter (the other ship, but only when in very close proximity) they reset their clocks. Now as it turns out: in relative terms, these ships, fly in a giant circle, 10 light years in circumference.
Assumption 1: There is no acceleration, just a constant speed. Since with each meeting the clocks are reset to zero, there is no need to “get up to speed or slow down” . We are looking simply at the time transmitted by each ship since the last transmission, and from these numbers we deduce the relativistic time dilatation.
Assumption 2: Although some may want to assume there to be a body bending space-time into a 10 Light year ring, you can just as easily imagine a coincidental collection of forces (space dust, static charges etc.) that just happen to bend the path(s) of the spaceship(s) into this huge circle. In either case, for the sake of this thought experiment, the requirement is to provide relativistic speeds that are symmetrical between spaceships A & B. We have no stopping, reversing direction, or changing acceleration issues to contend with.
Assumption 3: Let us just assume spaceship A is stationary and all of the relative motion is due to spaceship B, then relativistic time dilatation and space contraction would be due to B (as far as A is concerned anyway) . So 12.5 years later (A’s time) they pass each other again. A transmits a time that says: “it is 394,200,000 seconds since I last reset my clock” and promptly resets the clock. Spaceship B however has been moving at the 80% of the speed of light (again, as far as A is concerned). So, as far as A is concerned; time on spaceship B is moving at 60% of the speed of spaceship A. (If we were talking about the proverbial twins, we would say that the spaceship B twin aged 7.5 years while the A twin aged 12.5 years, but we don’t care to talk too much about those two trouble makers here.) We expect spaceship B to send a signal that says : “It is 236,500,000 seconds since I last reset my clock” and then promptly reset its clock.
Assumption 4: If I understand Relativity correctly, the clocks on both ships see the time on the other spaceship as moving slower than their own. This means it is just as correct to say; spaceship B is stationary and all relative movement was due to spaceship A.( Spaceship B's perspective) To spaceship B; it is A’s clocks that are moving slowly. But if A’s time is truly moving slowly (as far as B is concerned); then A will send upon arriving in close proximity to B: “It is 236,500,000 seconds since I last reset my clock” and then promptly reset the clock.
Assumption 5: This is an impossible Paradox. Surely A’s clock cannot be in a superposition of Assumption 3 and Assumption 4, transmitting BOTH 394,200,000 (Ass.3) seconds and 236,500,000 (Ass.4) seconds AT THE SAME INSTANT. It can only transmit One message or the Other.
Assumption 6: If I have erred and (somehow), the A’s clock transmits the same number of seconds from the point of view of either spaceship. Then one or the other of the ships was truly at rest, then space “knows” that one spaceship is truly moving while the other is stationary. Thus TIME is NOT FULLY RELATIVE. Time can still be relative with respect to speed, but it is not relative to each observer. Thus Spacetime is capable of dilatation or warping BUT it is with respect to velocity and shape the FABRIC of SPACE.
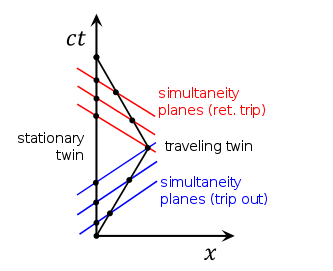
Assumption 7: Although the conventional telling of the “Twin Paradox” requires one of the twins to stay on earth, Special Relativity demands only relative speeds. So the retarded aging of a twin, like the slowing of the clock(s) should only depend on the reference frame of the observer.
Question, Simply put: Since motion alone must cause time dilatation, and since we can CHOOSE to assume Space A is stationary, (or we can CHOOSE to say Spaceship B is stationary), What does Spaceship A , transmit when they pass each other?
This is an accurate paraphrase of Professor Herbert Dingle’s telling of the famous paradox.