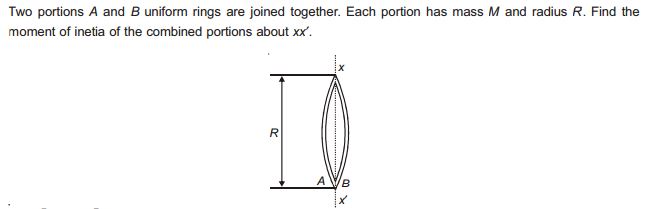
The source tells me to use the formula for a ring, but it is not possible, as the portions are nearer to the axis than a normal ring. How can I find the moment of inertia?
$\begingroup$
$\endgroup$
6
-
$\begingroup$ After working out the moment of inertia of an arc of a ring about the axis through the centre of the ring, you may then be able to use the parallel axis theorem. $\endgroup$– diraculaCommented Mar 6, 2017 at 21:30
-
2$\begingroup$ The $R$ in the drawing does not correspond to a radius. $\endgroup$– John AlexiouCommented Mar 7, 2017 at 0:48
-
2$\begingroup$ The R is also the radius of the rings. $\endgroup$– Aaron John SabuCommented Mar 7, 2017 at 19:35
-
$\begingroup$ Thus angle subtended by each portion of ring at the corresponding centres become 60 degrees or pi/3 rad. $\endgroup$– Aaron John SabuCommented Mar 7, 2017 at 19:36
-
$\begingroup$ So for each ring, R is the radius of the ring and also the length of the chord that defines the minor arc pictured in the figure? $\endgroup$– DevsmanCommented Mar 7, 2017 at 19:37
|
Show 1 more comment
2 Answers
$\begingroup$
$\endgroup$
3
You know that to solve this problem you will have to use the integral form for MoI.
$\int_0^Mr^2dm$ where $dm$ is the mass element (geometry of the problem) and $r$ is the distance from the axis of rotation.
You express the mass element in terms of $r$ so you get linear density.
Ex. Rod of mass $M$ has linear density $M/L$ so you get $dm=\frac MLdr$
Ex.2 A solid cylinder has volume density of $dm = \rho L2\pi rdr$ (density * length * circumference or $\rho dV$)
etc.
So, you find the distribution of mass (geometry) and plug it into the integral.
Another way you could solve it is by using the Parallel Axis Theorem ($PAT$)
$I_{parallel} = I_{cm} + Md^2$.
You know that the $I=\frac{MR^2}2$ for the whole ring.
To sum up, imagine that you have a whole ring, which you cut on both sides and you get a sort "brackety" system () (removing the "middle" part from a nice round circle) which you can then solve using the integral method of $PAT$ method ($\frac{MR^2}2 + Md^2$ where $d$ is the distance from the axis and the same point where you cut to get one portion of the ring $->$ substituting)
-
$\begingroup$ What I wanted was there in the integral form. I solved it. Thanks although the rest of the answer was not of any direct help. $\endgroup$ Commented Mar 7, 2017 at 19:34
-
$\begingroup$ So, did I help in any way, if not, sorry, did my best. I gave both versions of coming to the solution, one through direct integration of $r^2dm$ and one through manipulation of the parallel axis theorem. $\endgroup$ Commented Mar 7, 2017 at 20:37
-
$\begingroup$ Your help has been acknowledged, in the form of upvotes and in the form of 'Best Answer'. $\endgroup$ Commented Mar 8, 2017 at 2:39
$\begingroup$
$\endgroup$
0
Use the parallel axis theorem for calculating the moment of inertia relative to a point which is not the center of mas (COM). See for example here in Wikipedia.
$I=I_{\rm COM} - m d^2 ,$ where $d$ is the distance between COM and the point of rotation.
