At this Wikipedia page, we've that the 'Transformer universal EMF equation' looks like:
$$\text{E}_{\text{rms}}=\frac{2\pi\times\text{f}\times\text{n}\times\text{a}\times\text{B}_{\text{peak}}}{\sqrt{2}}\approx4.44\times\text{f}\times\text{n}\times\text{a}\times\text{B}_{\text{peak}}$$
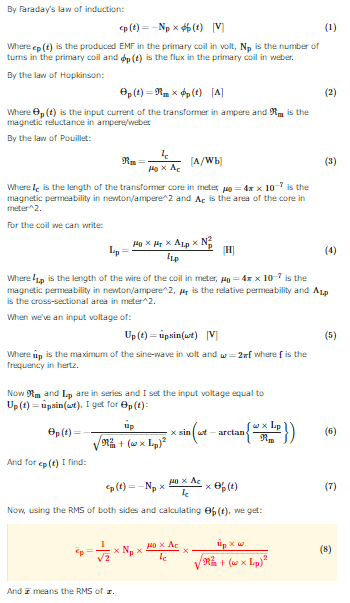
In this question there is a picture that derives the EMF equation but it does not is the same as the one that is stated at wikipedia (which is supposed to be the EMF-equation):
$$\overline{\epsilon}_\text{p}=\frac{1}{\sqrt{2}}\times\text{N}_\text{p}\times\frac{\mu_0\times\text{A}_\text{c}}{\mathcal{l}_\text{c}}\times\frac{\hat{\text{u}}_\text{p}\times\omega}{\sqrt{\Re_\text{m}^2+\left(\omega\times\text{L}_\text{p}\right)^2}}$$
Question: Why does those not equal eachother, where am I wrong?
Picture: