Regarding point 1: if you consider the center of mass stationary (observe the two blocks in their center of mass frame), then the two blocks will move towards each other and away again. The velocity of one ($m_1$) will always be $\frac{m_2}{m_1}$ of the other (conservation of momentum). Considering the c.o.m. as the origin, that origin will be fixed and each mass will move as though it only "sees" the bit of spring on its side of the c.o.m. (dashed line = location of center of mass):
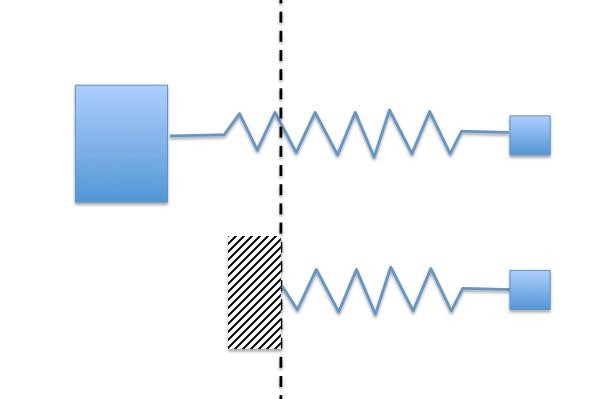
It follows that you can use reduced mass (although I prefer, from the visual above, to use the normal mass and scale the $k$ of the spring. Same result, mathematically). And if you look for conservation of energy, at any moment the sum of kinetic energies of the two blocks plus the elastic energy stored will be constant. However, it's not clear that your expression in (2) would be correct - there is no reason that the velocity of the two blocks would be a maximum at the same time (except in the c.o.m. frame).

