Number of collisions of one ball between two another balls

Question

May be you know about famous problem for its answer.

Place two billiard balls ($B_1$ and $B_2$) on the half-line $[0,\infty)$: the $B_1$ is at rest, the $B_2$ is to the right of it and is moving the left. At $x=0$ there is an elastic wall. Assume perfectly elastic collisions: the kinetic energy is preserved. How many collisions will happen depends on the masses of the ball, or rather their ratio.

For more details see Here.

---

Let me change condition of this problem a bit.

Place two billiard balls ($B_1$ and $B_2$). Both are at rest. Next, place one more ball $B_0$ between $B_1$ and $B_2$ which moves to the ball $B_2$. Every collision is elastic: kinetic energy is preserved. The same question.

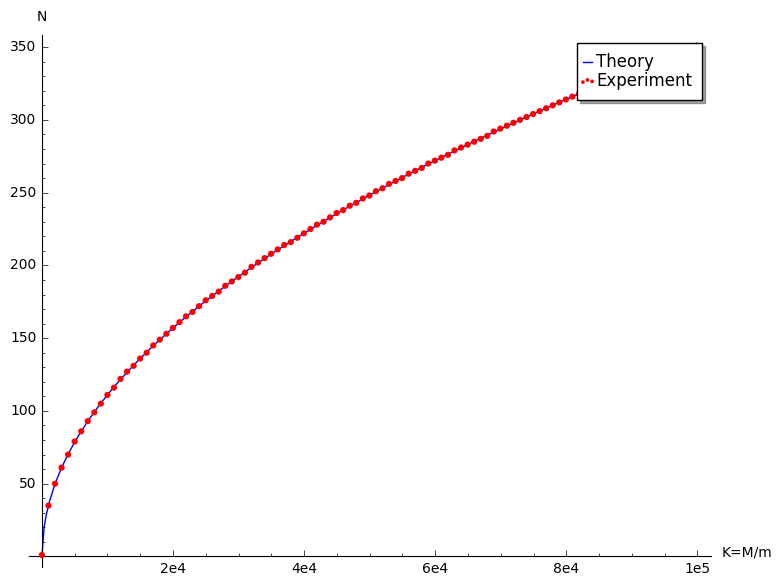
We can begin with simple case when masses of $B_1$ and $B_2$ are equal to $M$ and mass of $B_0$ is equal to $m$.

---

I tried to solve this and my result is a system of recurrences which I am not able to solve. I will post my solution below.

Answer 1

Let us introduce some notations.

Notations

$M, m$ for mass of $B_1$ ($B_2$) and $B_0$ respectively.
$K$ for $\frac{M}{m}$
$V$ for velocity of $B_0$
$W$ for velocity of $B_1$ or $B_2$
$V_i$ for velocity of $B_0$ after $i$th collision.

---

Next, it easy to get some helpful results. But firstly note that answer is not depends on initial value $V_0$ of $B_0$ therefore without loss of generality we can assume $V_0 = 1$. So, see, fig. $$W_1 = \frac{2}{1+K},\quad\text{i.e. velocity of $B_2$ after 1st collision}$$ $$V_1 = \frac{1-K}{1+K},\quad\text{i.e. velosity of $B_0$ after 1st collision}$$ $$W_2 = \frac{2}{1+K}\cdot V_1,\quad\text{i.e. velocity of $B_1$ after 2nd collision}$$ $$V_2 = \Bigl(\frac{1-K}{1+K}\Bigr)^2,\quad\text{i.e. velocity of $B_0$ after 2nd collision}$$ And so on. You get the point. We can write it in general: \begin{align} V_{i+1} &= (V_i - W_{i-1})\frac{1-K}{1+K} + W_{i-1}\\ W_{i+1} &= (V_i - W_{i-1})\frac{2}{1+K} + W_{i-1} \end{align} with initial conditions $$V_0 = 1, W_{-1} = W_0 = 0$$ That's all. Obviously, that if for some $i$ it is fulfilled that $$|V_i| \leq |W_{i-2}|$$ then process terminated. But how to know the first such $i$. I solved these recurrences numerically and this what I have got. Where Theory is $$f(K) = \frac{\pi\sqrt{K}}{2\sqrt{2}}.$$ Where this function comes from? In paper mentioned by @sammygebril in comments similar problem solved. That result is two times bigger, i.e. $$f(K) = \frac{\pi\sqrt{K}}{\sqrt{2}},$$ for $K\gg1$.