Fracture happens under tension - that is, when you pull on something hard enough, it rips. The key to understanding the arc design hinges on understanding that it lowers the maximum tensile force.
Take a simple beam, support it at the ends, and hang something off the center:
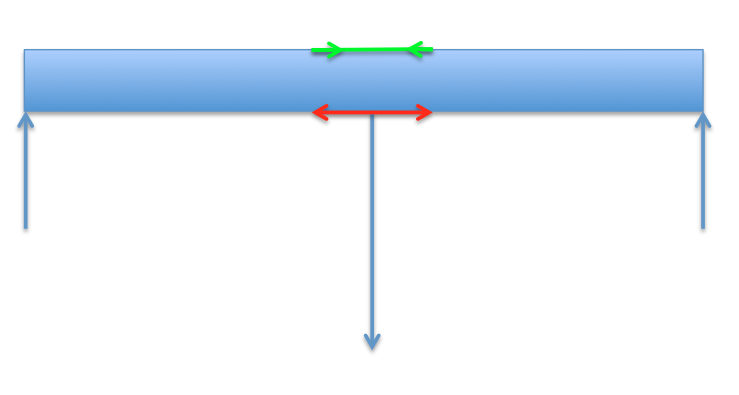
Tension at the bottom, and compression at the top, are needed to balance the torque created by the vertical forces of the supports, and the load in the middle. Obviously, the further apart the supports are, or the greater the load, the greater the tension. When that tension reaches a critical value the beam will fail.
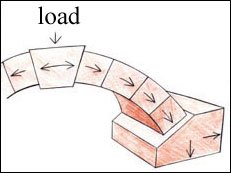
Now if we shape the bridge into an arc, we get this:
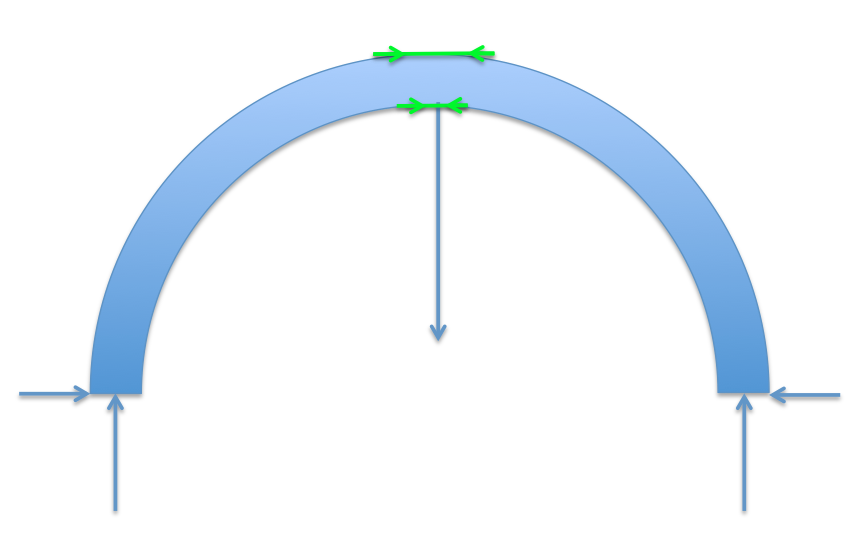
The additional lateral forces on the arc cause compression in the beam, this reduces the net tension at the bottom and makes the beam better able to support the load. You can make things even better by spreading the load more evenly, designing the shape of the arc to better optimize the load distribution, etc - but the diagram should give you a sense of the underlying principle.
Update
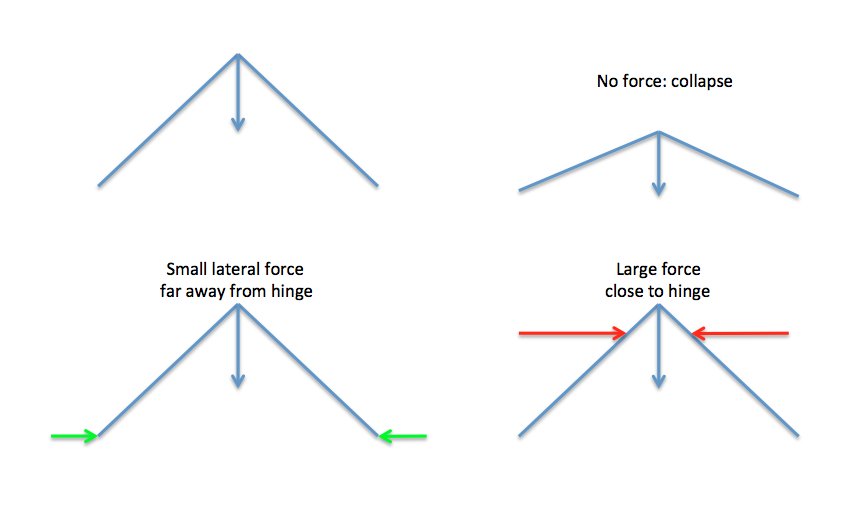
The lateral forces are perhaps most easily understood by looking at a V shaped structure: you know intuitively that such a structure would collapse unless you provide some torque at the apex to keep the legs together, or provide sufficient friction at the base of the legs to keep them together. You can also see that the force needed near the hinge (which is provided by the red "tension" stress in my upper diagram) would need to be much greater than the force provided by friction at the bottom (lateral forces from the support on the arch).