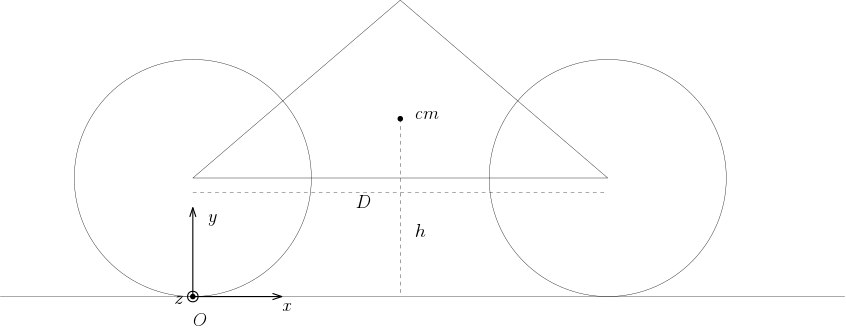
I am having trouble understanding The principle behind adding the angular momentum of the wheels to the angular momentum of the centre of mass of the bike with respect to a coordinate system with its origin at where the back wheel touches the ground . I understand how to calculate the angular momentum of each wheel around its centre, and how to calculate the angular momentum of the centre of mass, it's finding the total angular momentum I have trouble with. The question is as such: A bike moves forward with velocity V and it's wheels rotate with angular velocity w (without slipping). The distance between the two wheels is D and the centre of mass of the whole bike is located half way between the wheels and at a height h above the ground. Find the total angular momentum.
1 Answer
The first point to realize is that the bike is not a rigid body. You can decompose it in three different parts (the two wheels and the bicycle frame), evaluate separately the angular momentum of each and sum.
For each wheel, the total angular momentum is given by the angular momentum with respect to the wheel's center of mass, plus the angular momentum of the center of mass. This gives
$$\vec{L}_1 = \vec{L}_2 = -\left(I_{w,cm} \frac{V}{R_{w}} + m_{w} V R_{w}\right) \hat{z}$$
for the two wheels, where $I_{w,cm}$ is their momentum of inertia with respect to their center of mass, $R_w$ their radius and $m_w$ their mass (I supposed that the two wheels are identical). Note that $-V/R_w = \omega$ is the angular velocity of each wheel.
For the bicycle frame we get
$$\vec{L}_f = - M V y_{cm} \hat{z} $$
because it just translate horizontally. Here $y_{cm}$ is the vertical position of the frame's center of mass, and $M$ the frame's mass. Adding together all the pieces we get
$$\vec{L} = -2 I_{w,cm} \frac{V}{R_{w}}\hat{z} - 2 m_{w} V R_{w} \hat{z} - M V y_{cm} \hat{z}$$
but this can be rewritten as
$$\vec{L} = -2 I_{w,cm} \frac{V}{R_{w}}\hat{z} - \frac{2 m_{w}R_w + My_{cm}}{M+2 m_{w}} (M+2 m_{w}) V \hat{z} $$
but
$$M_{tot}=M+2 m_{w}$$
is the total mass of the bicycle and
$$h = \frac{2 m_{w}R_w + My_{cm}}{M+2 m_{w}}$$
is the horizontal position of the bicycle's center of mass. So
$$\vec{L} = -2 I_{w,cm} \frac{V}{R_{w}}\hat{z} - h M_{tot} V \hat{z} $$
The final result is the sum of the angular momentum of the rotating wheels with respect of their center of mass, plus the angular momentum of the center of mass of the bicycle.