Equations of motion along different paths

Question

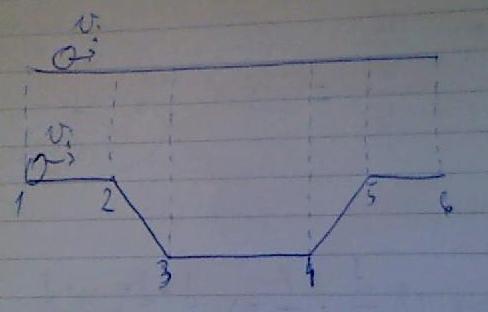
I remember a question that goes down like this. Imagine two balls starting with the same initial velocity on separate paths. It looks like this:

(ignore that they aren't at the same position in the drawing - a mistake).

Which one finishes the path quicker?

I remember this way of reasoning that concludes that the 2nd ball will come quicker.

We are observing only the x-axis, since y-axis motion is irrelevant for the question posed. Both balls cover the path from 1 to 2 in the same time. However, from 2 to 3 the 2nd ball accelerates and covers that path in less time than the 1st ball. From 3 to 4 the 2nd ball is moving with a new velocity higher than the velocity of the 1st ball thus again the 2nd ball covers that path as well faster than the 1st ball. Now from 4 to 5, the 2nd ball is experiencing negative acceleration, however the velocity of the 2nd ball is again always greater than the velocity of the 1st ball and thus again the 2nd ball covers that portion in less time than the 1st ball. Now the 2nd ball has made substantial advantage over the 1st ball, and thus their separation distance is constant from 5 to 6. Now this seems reasonable to some extent but incredibly counterintuitive and I am having trouble understanding this to the fundamentals.

Answer 1

The motion along the y-axis is absolutely not irrelevant. You are right to feel bad about that answer!

Assuming the balls stay attached to the ground underneath them, the second ball has to do two things differently from the first:

• Travel the distance up and down while changing speed, and
• Cross the valley gap faster than otherwise.

The ball with the complex motion will only beat the simple one if the gains crossing the gap at speed overpower the losses traveling the extra distance up and down. Think about the limit as the width of the gap goes to zero - if the complex-motion ball just stops in the middle to travel up and down there's no way it could beat the first.

Let's treat the problem as if the pit's sides were vertical and the ball was on a track that attached it to the edges. The part of the motion we care about is only the part that involves the pit, as the rest is the same. With initial rolling velocity V, pit depth H and pit width W, we have that:

• The time spent moving down is $\sqrt{V^2/g^2+2H/g}-V/g$.
• The time spent moving back up should be the same.
• The time spent moving across is $W/\sqrt{V^2+gH}$

Adding them all together gives us: $$ 2\left(\sqrt{V^2/g^2+2H/g}-V/g\right) + W/\sqrt{V^2+gH} $$

Which, as we would expect, reduces to $$ W/V $$

when H is 0.

Clearly, (1) being greater or less than (2) is a complicated situation!

The answer that you remember would be true if the "pit" was not an actual pit, but was instead a potential well whose presence didn't add anything to the distance. If both balls were on a straight track but the second one had repelling magnets on either side, the magnet-ball would win by the argument your question posed.