It's true that photons can create electron-positron pairs. However, these photons must have quite a lot of energy - at least 1.022 MeV. This falls solidly into the energy range of gamma rays. Now, gamma rays may be produced in varying amounts in stars of all different masses, but they don't become significant sources of pair production until temperatures of $\sim10^9$ or $\sim10^{10}$ Kelvin, about 100 times as hot as the center of the Sun. Photodisintegration becomes a possibility at $\sim10^{10}$ Kelvin. Neither of these processes become quite as important in stars under about 100 solar masses.
Now, radiation pressure can become very important or even dominant over gas pressure at much lower temperatures and masses, often at $\sim10^8$ Kelvin, corresponding to stars on the order of 10 or so solar masses. There's a substantial mass/temperature range between these stars and stars where pair production is important.
This pdf has a couple interesting graphs in the $\rho-T$ plane that you might be interested in. Here are two on radiation pressure and gas pressure:
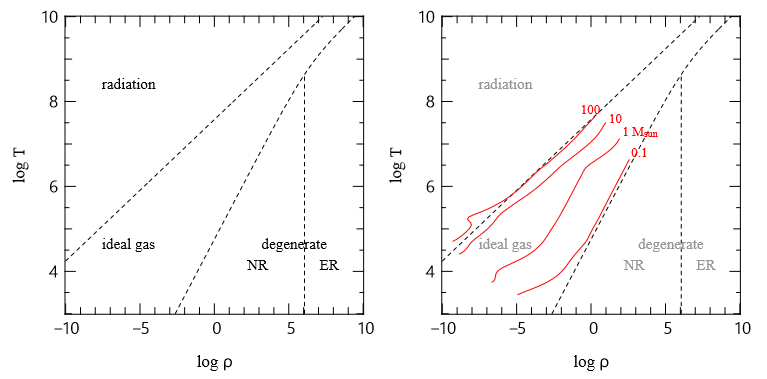
Here's one on pair production:
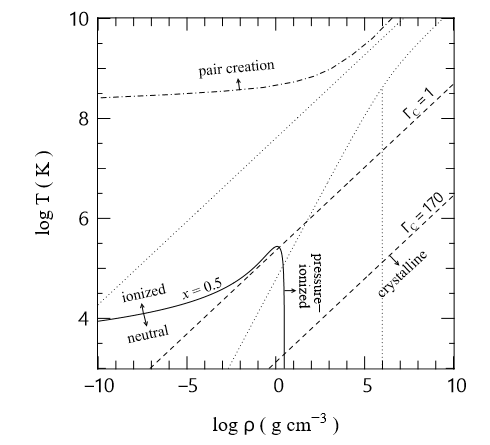
(I'll admit that the 100 M$_{\odot}$ track seems suspiciously low, but it's still not yet near the threshold for pair production to become important.)
What happens in stars that have masses high enough for pair production to become truly significant? This limit isn't at 100 solar masses, but a bit higher; Wikipedia states that at 130 solar masses, a pair instability supernova may occur. At this point, you do have conflict between pair production and radiation pressure.


