Solar System resides in a plane, thanks to Conservation of Angular Momentum. The Milky Way is also a disc, not sphere.
What angle does our Solar System's plane (or, normal to plane) make with The Milky Way's plane (or, normal to plane)? Does our Solar System also resides in the same plane of our Galaxy, making the angle zero? If yes, why? If no, what exactly is the angle? Is it not constant? Any function describing the relationship?
The Sun is approximately in the plane of our Galaxy - see this Astronomy SE question. The ecliptic plane (plane of the solar system) and the Galactic plane (the plane of the disc of the Milky Way) are inclined to each other at an angle of 60.2 degrees.
This is a point you can confirm yourself by noting that the Milky Way does not follow the signs of the zodiac (which follow the ecliptic plane).
There is really no reason that there should be any alignment. Star formation is a turbulent, chaotic process. The evidence so far is that this leaves the angular momentum vectors of individual stars, their discs and ultimately their planetary systems, essentially randomised.
The question only asks "What angle does our Solar System's plane (or, normal to plane) make with The Milky Way's plane" -- to which 60 degrees is the answer. To completely specify the relative geometry of the planes we can ask what are the Galactic coordinates of the ecliptic north pole?
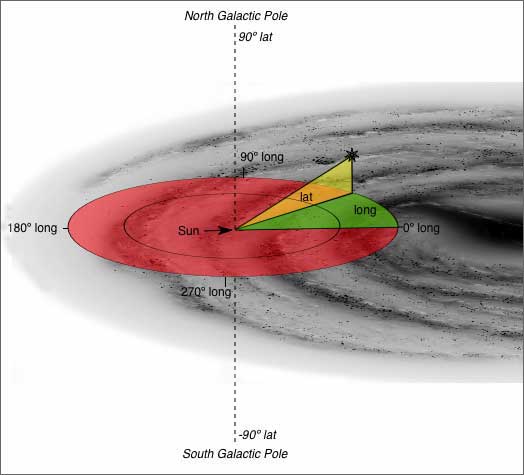
The ecliptic north pole (the pole of the Earth's orbit and the direction in which a normal to the ecliptic plane points) is currently at around RA$=18$h, Dec$=+67$ degrees in the constellation of Draco. In Galactic coordinates this is $l=97$ degrees, $b=+30$ degrees, compared with the normal to the Milky Way plane which is at $b=+90$ degrees (where $l=0$ points towards the Galactic centre and $b=0$ is roughly defines the plane of the Milky Way).
Edit: Note that as pointed out in comments, the ecliptic plane is not quite the same as the plane of the solar system. They differ by about 1.5 degrees.
Celestial sphere is in angle a of 63° toward galaxy disk, but Ecliptic plane (plane of the solar system) is only 60,2° - galactic latitude and this plane is facing toward 120° (Cassiopeia) (0° is Sagittarius etc) of galactic longitude.
I am showing two pic from wiki, which are clearly stating, that eliptic plane is in 60, 2°to galaxy - which is in contrast of previous comment Rob Jeffries (astrophysics), which I highly respects.
It is also not clear from Rob Jeffries that galactic north is close toward Polaris Galacticum Borealis (const. Coma Berenices) which is in Galactic coordinates 120° (Cassiopea) - which also confirm my test on stellarium (bellow) and I also read this 120° in a Springer¨s article, but can not find it anymore to give link (so I could be wrong).
Plausible check on Stellarium: autumnal equinox (23. 9. 2019) at noon is on 27° Longitude, if you remove ground and check 180° opposite night sky and subtract 60° (Ecliptic plane angle, 23° Earth pole axis now does not play a role) you see that you point toward 120° (Cassiopeia).
23° rotation around sun causing 360° rotation of milky way on the night sky and it´s shift 23° during the year.
