0
$\begingroup$

What is the direction of our solar system in the Milky way galaxy and in the whole universe? Are we heading toward another constellation, not including Andromeda?

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Would Astronomy be a better home for this question? $\endgroup$
    – Qmechanic ♦
    Commented Feb 22, 2015 at 22:16
  • $\begingroup$ Related question on Physics: physics.stackexchange.com/q/108033 Related question on Astronomy: astronomy.stackexchange.com/q/8901 The latter explains the fallacy that there is a preferred direction in the universe. $\endgroup$
    – HDE 226868
    Commented Feb 22, 2015 at 22:45
  • 1
    $\begingroup$ We don't necessarily have uniform motion towards a constellation as each star in it may have a different velocity. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Feb 22, 2015 at 23:06
  • $\begingroup$ I once asked this question on a school excursion to a planetarium. You could hear the crickets chirping afterwards! $\endgroup$
    – CJ Dennis
    Commented Jun 19, 2015 at 13:21

1 Answer 1

Reset to default
1
$\begingroup$

Pretty much everything in the Milky way orbits around in the same direction. It's a big spinning disk. The Solar System completes and orbit every 200 million years or so. Now, what "direction", other than "the same direction as anything else", I'm not sure there's an answer cause there's no clear frame of reference.

Andromeda and the Milky Way should collide in about 4 billion years, or 20 orbits or so from now.

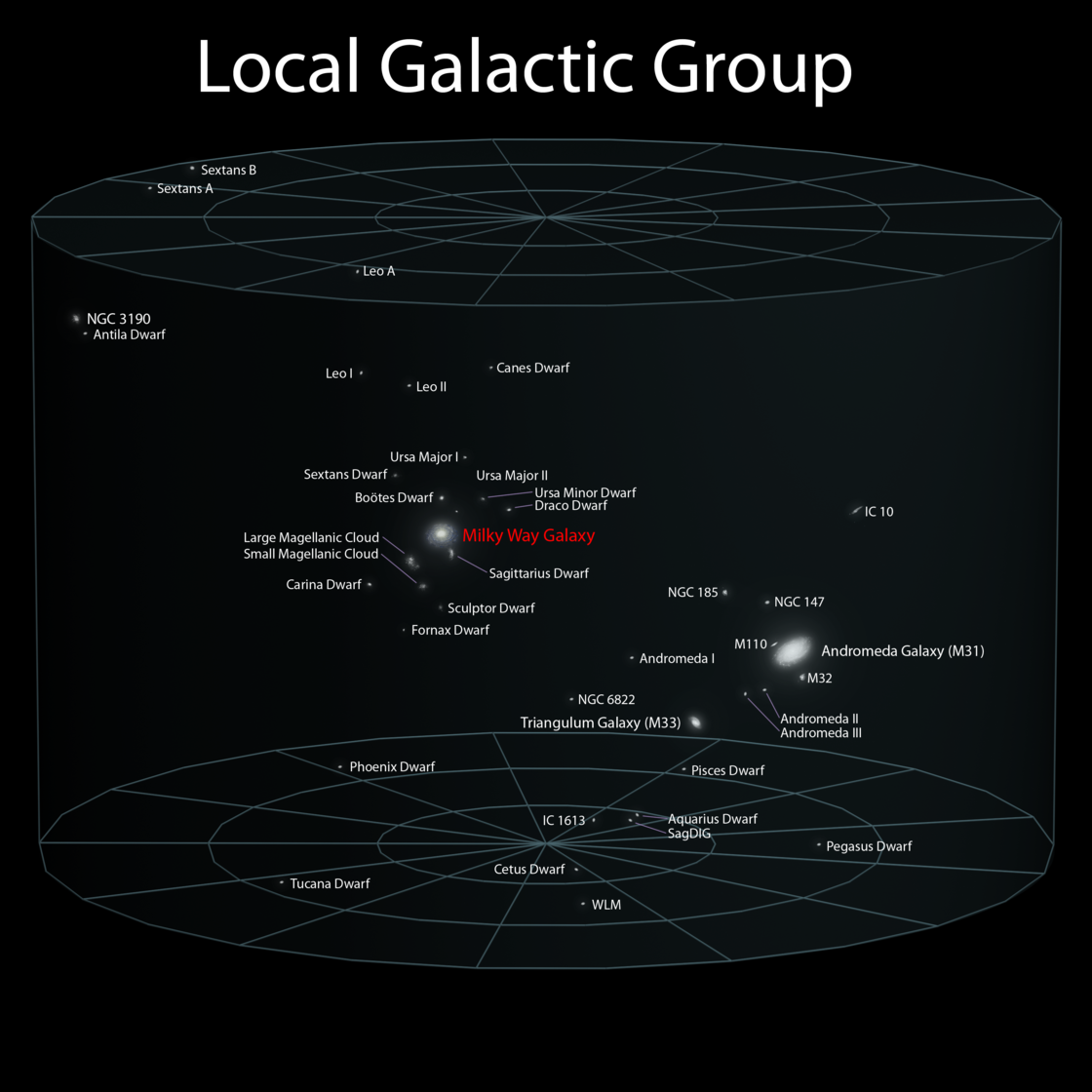
As to whether we're heading for another collision, well, probably, but none as exciting as the Andromeda one cause Andromeda's much bigger. There's a number of dwarf galaxies in our local group and many of them a lot closer to us than Andromeda.

We may actually be in the process of a collision with a dwarf galaxy, Canis Major. (see article below). http://www.universetoday.com/21914/the-closest-galaxy-to-the-milky-way/

here's a map of the local group (you won't see Canis Major in local group map cause it's already inside the Milky Way.

Sagittarius is the closest dwarf galaxy.


(source: wikispaces.com)

Improve this answer
$\endgroup$
1
  • $\begingroup$ appreciate this map $\endgroup$
    – verdai
    Commented Mar 2, 2015 at 20:07

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.