11
$\begingroup$

I am a little confused about this question. I cannot imagine that it is possible to rise through a rope (assuming inextensible) that isn't fixed in its upper end. But when I solve this problem, I see that it is possible. 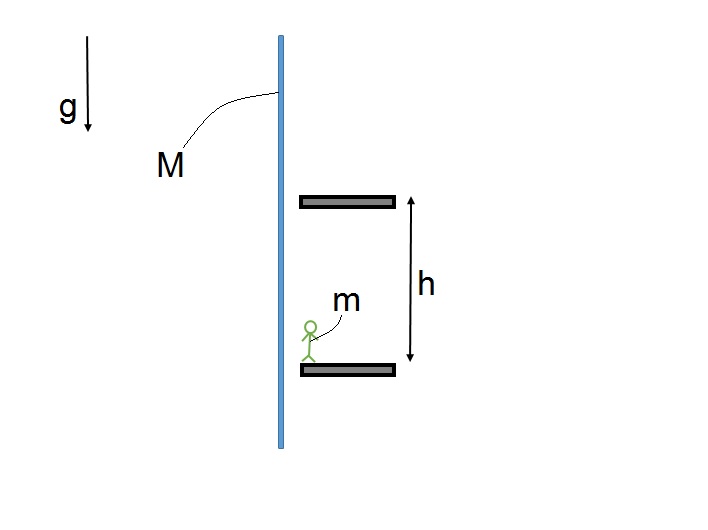enter image description here If the person exerts downwards force of $F$ on the rope, according to the third law of Newton, the rope will exert a force by the same magnitude but in the opposite direction on the person. So, his acceleration ($a_m$) will be: $$a_m=\frac Fm-a_M$$ That $a_M$ is the magnitude of the acceleration of the rope.

On the other hand, we have: $$a_M=g+\frac FM$$ Thus, $$a_m=F\left(\frac 1m-\frac 1M\right)-g$$ Now, if $F\left(\frac 1m-\frac 1M\right)\ge g$, then $a_m\ge 0$ and this means that it is possible to rise through a free-falling rope.

But I suspect I am missing something (maybe because of overthinking!) because the upper end of the rope is free and I wonder if it is possible to rise!

Improve this question
$\endgroup$
6
  • $\begingroup$ you would have to pull a lot of rope in quick succession if you want to negate the effect of gravity. Correct me if I'm wrong but this would be easier to do in a medium than without one (Since you're pushing the rope against air as well, providing more resistance and supplying you with more force in the opposite direction of the free-fall) $\endgroup$
    – Obliv
    Commented Jul 19, 2016 at 16:18
  • $\begingroup$ That's what rockets do. You have to ask Elon Musk and NASA if they are just faking all those launches. Who knows... maybe we have never been to the Moon? :-) $\endgroup$
    – CuriousOne
    Commented Jul 19, 2016 at 16:23
  • 2
    $\begingroup$ As a practical matter, a human being can't move his or her arms fast enough to accomplish this feat in a $1g$ field. As a physics problem it is obviously consistent with the principles of mechanics: it is neither more nor less than Newton's laws or the principle of Conservation of Momentum. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Jul 19, 2016 at 20:33
  • $\begingroup$ @dmckee Thanks! I think so. It is so similar to cartoons that I was seeing when I was a child! And I don't know why I felt it is impossible!!! :-) $\endgroup$
    – lucas
    Commented Jul 20, 2016 at 1:59
  • 2
    $\begingroup$ Some fishes are known to swim vertically up to some extent, against free falling water. Humans can not, due to limitations. It also depends upon how long the rope or water has fallen. Longer it falls, harder it is to be used as a support for rising. Fishes can do it because the water falls only a couple of feet. If it fell 100s of feet, they can not either. $\endgroup$
    – kpv
    Commented Jul 20, 2016 at 3:08

2 Answers 2

Reset to default
10
$\begingroup$

Yes, it is possible to rise theoretically w.r.t a ground frame. But the rope-man system's center of mass must keep moving downwards because of the only external force acting on the system (gravity). The lighter $M$ gets, the harder it will be for the man to rise, and it will become impossible in the limit the rope becomes mass-less. This is intuitive because then the center of mass of the system is effectively that of the man's alone, and it must therefore move down only.

Improve this answer
$\endgroup$
0
$\begingroup$

Yes you could rise up the rope. You need to exert a force more than your weight, f = your mass x g. But you have to rappel exceedingly faster.
Basically you could look at this as a rocket which is constantly refueling. If you exert less than your wait you break your fall to the degree of your force. You'd need lots of rope though depending on its mass.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.