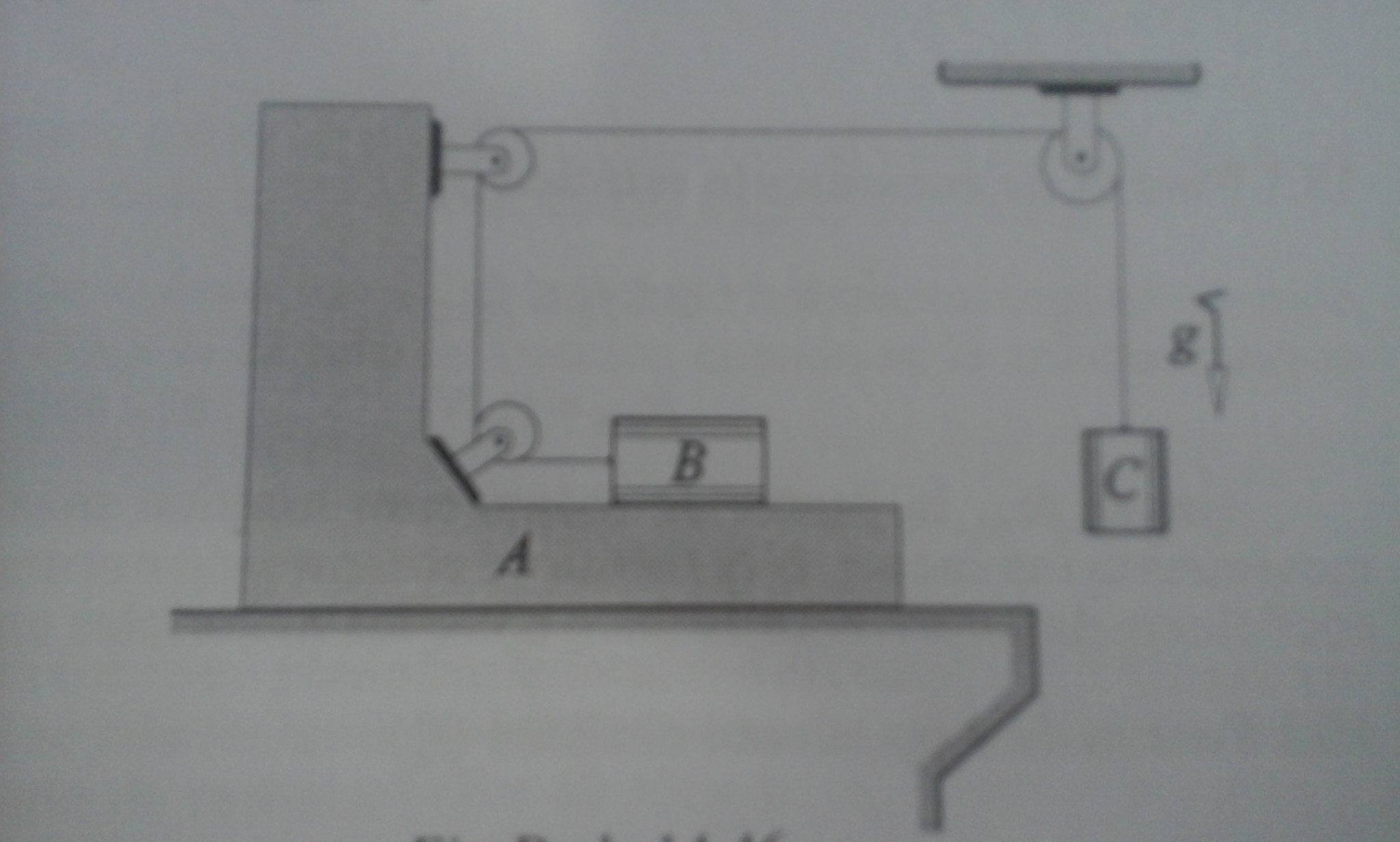
The system in the figure is initially at rest . If the system starts to move , evaluate the acceleration of the block $A$. Mass of the block $A$ is $m_A = 60\;\mathrm{kg}$ ; mass of blocks $B$ and $C$ are same and $m_B=m_C=20\;\mathrm{kg} $. (There's no friction between the bodies and the mass of the pulleys is negligible).
Answer: $a_{A} = 2\;\mathrm{m/s^2}$
First problem: my solution isn't working.
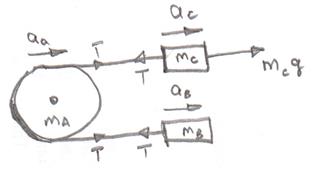
I've been trying to solve this problem in the non inertial frame of reference, i.e., in the frame of reference of the block $A$, using Einstein's Equivalence Principle, where inertial forces are acting. Inertial forces acts on $A$, $B$ and $C$, and $A$ is at relative rest in it's own frame of reference. In the frame of reference of $A$, $B$ is moving to the left while $C$ move to the left and moves down simultaneously. I've written the equations using the Newton's Second Law for the three bodies, but my answer differ from the answer of the book.
Second problem: I dont understand the solution's manual.
The book solve the problem in the inertial frame of reference of Earth. He writes Newton's Second Law for the three bodies (no problem here) and then say that we can consider the block $A$ as a "giant moving pulley", so we have $\vec{a_{A}} = \frac{\vec{a_{B}}+\vec{a_{C}}}{2}$, and then, combining the Second Law with this vector equation, we have $a_{A} = 2\;\mathrm{m/s^2}$ I understand the vector equation for moving pulleys, but I don't understand why we can take the block $A$ as a "giant moving pulley". Can someone explain this please?