Question on the constraint force of a string

Question

I would like to know if the following statement is true or false even if I expect that it is true.

Notation: I will consider a string that has no mass and that can not be extended. Saying that a force has downward trajectory I will mean a force such that goes toward the floor, so this force is not necessarily vertical.

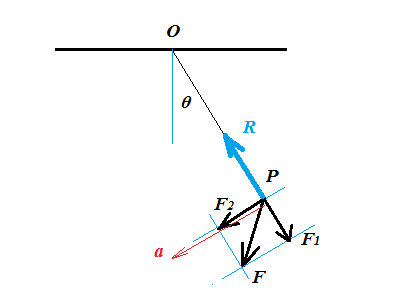
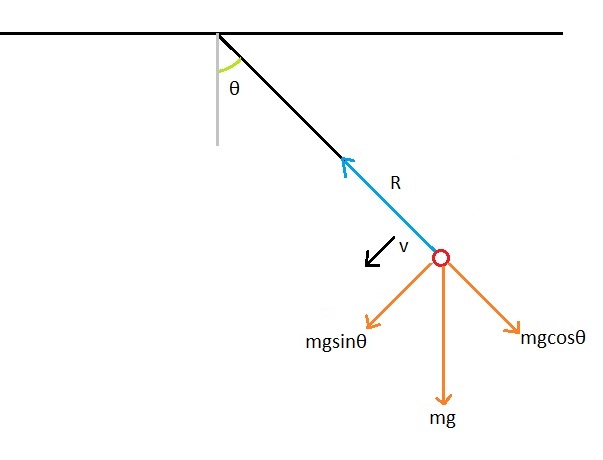
Statement: Consider a vertical string with an extremity fixed on the ceiling. If we attach on the other extremity a point (with or without mass it's the same) and there is a force $\vec F$ acting on this point with downward trajectory, then only the component of $\vec F$ that is parallel to the string "causes" the constraint force $\vec R$ of the string. Furthermore, this $\vec R$ has the same intensity of the parallel component of $\vec F$ that we have just mentioned, but opposite direction.

Moreover, if it is true, can we say that it is an experimental law ?

Answer 1

You would be right, if the string is like a real string in that it's flexible (that is, can neither exert transverse force nor bear transverse loads). I have to point out here that you haven't described a system (classically, you couldn't draw a static free body diagram for the string)--to be static, the entire force $\vec{F}$ would have to be parallel to $\vec{R}$. Otherwise, the string would simply pull parallel to $\vec{F}$ (it is a string, after all--it would simply bend and pull straight in that direction). This is a simple result of Newton's Third Law applied to the end of the string--the force applied to the end of the string must be equal in magnitude and opposite direction to the force applied by the end of the string to whatever's pulling on it. If the end of the string isn't moving, then by necessity $\vec{F} = -\vec{R}$.

Answer 2

I think you are confusing static and dynamic or are trying to separate the two in a way that isn't helpful and leads to 'over-thinking' of a simple problem. Let's look at the force diagram again:

In the point $P$ a downward (but as you stated not necessarily vertical) force $\vec{F}$ acts on the end of the string. We decompose it into two components: $\vec{F_1}$ which is parallel to the line $OP$ and $\vec{F_2}$ which is perpendicular to the line $OP$.

Assuming the string is strong enough it will provide a reactive force $\vec{R}$, so that $\vec{R}=-\vec{F_1}$.

Now when we look at the forces acting on point $P$ along the axis perpendicular to $OP$ we can see there's a net force acting on $P$, that is the force $\vec{F_2}$. By Newton's second law this means there must now be acceleration along that axis and in the direction of $\vec{F_2}$. It's shown as the red vector $\vec{a}$.

We can't know the magnitude of the vector $\vec{a}$ yet, as no masses have been specified. Some mass must reside in the point $P$ or the string or both (without mass the acceleration would be infinite!)

In addition, as pointed out by philip_0008, as soon as the point $P$ has gained even the tiniest velocity (a consequence of the acceleration it's subject to) a centripetal force $\vec{F_c}$ must act on $P$, along the $OP$ axis and in the direction of $O$. Without it the point $P$ cannot stay on its circular path.

This means that at $t=0$ then $\vec{R}=-\vec{F_1}$, but at $t>0$ then $\vec{F_c}=\vec{R}-\vec{F_1}$.

So, in the absence of forces other than $\vec{F}$ this by definition a dynamic problem: without some counter-acting force motion will always be the result.

Answer 3

Your statement is quite a complex one, involving several clauses. It is not clear which clause is the focus of your question.

You state that the string is vertical and the applied force is downward, so the force $F$ has no component perpendicular to the string, only in the direction of the string.

You do not explain what you mean by the "constraint force" $R$ of the string. I presume that you mean the tension within the string. This is indeed equal in magnitude to the component of force $F$ in the direction of the string. However, the tension in the string acts in both directions - upwards and downwards.

If the applied force $F$ did have a component perpendicular to the string, this component would not contribute to the tension in the string.

---

In reply to your comment :

Yes, R and F are equal in magnitude because of Newton's 3rd Law. The paired forces in this law act on different bodies : F acts on the string, R acts on whatever body X is supplying force F.

However, the force R which the string exerts on X is not the same thing as "the tension within the string". Whereas R is an external force acting on X, the tension in the string is an internal force which acts on and between the particles of the string. Each section of the string is pulled both downward and upward by forces of magnitude R from adjacent sections.

If the string's mass were taken into account then the tension in the string would decrease from top to bottom, because the upper part must suppport more weight than the lower part. The force which the string then exerts on a mass hanging on the end would not equal the variable tension in the string, only that at the end.

Answer 4

This might further clarify (or confuse) things:
The force that an object exerts on the string is equal in magnitude to the force that the string exerts on the object.
The force that an object exerts on the string (or similarly the force that the string exerts on the object) is not necessarily equal in magnitude to the component of a force acting on the object parallel to the string (especially in non-static cases, where the object actually has mass). Consider this example from the pendulum:

In this situation, The component of the object's weight is $mg\cos\theta$ and the tension force is $\vec R$. It is necessary that the tension force $R$ is greater than $mg\cos\theta$ if the mass is moving, in order to obtain a net centripetal force $F_c = mg\cos\theta - R$, necessary to keep the object following a circular path. Note that $R$ here is still the magnitude of the force the object exerts on the string (and vice versa), but the component of the total force acting on the object parallel to the string is $F_c$, and the component of the weight acting on the object parallel to the string is $mg\cos\theta$.

---

In response to your comment:

@Richard It's different if you actually put mass on the stick, as opposed to just forces. If you deal with masses, and you assume it to be non-static (i.e. accelerating), you have to deal with the additional force (which will be provided by the string, assuming someone is holding the other end of string) needed to accelerate (or decelerate) the masses, so that the magnitude of the tension force will be different from the component of the weight acting on the object parallel to the string.
However, if you assume it to be just forces acting on the stick or string (without masses), then there is no mass to accelerate, and you only need to balance it with a force with equal in magnitude, but opposite in direction.
Also note , as Ben G. pointed out, that without mass, if the total force acting on the string is not entirely parallel to the string, "it would simply bend and pull straight" in the direction of the force (instantly), because there is no mass to accelerate, so that you would instantly achieve a static configuration.
In the static case where you have 2 or more forces with different directions, then "only the component of any force $\vec F_i$ that is parallel to the string "causes" the constraint force $\vec R$ of the string". Furthermore, "this $\vec R$ has the same intensity of the 'total sum' of the parallel components of the 'forces' $\vec F_i$ that we have just mentioned, but opposite direction."