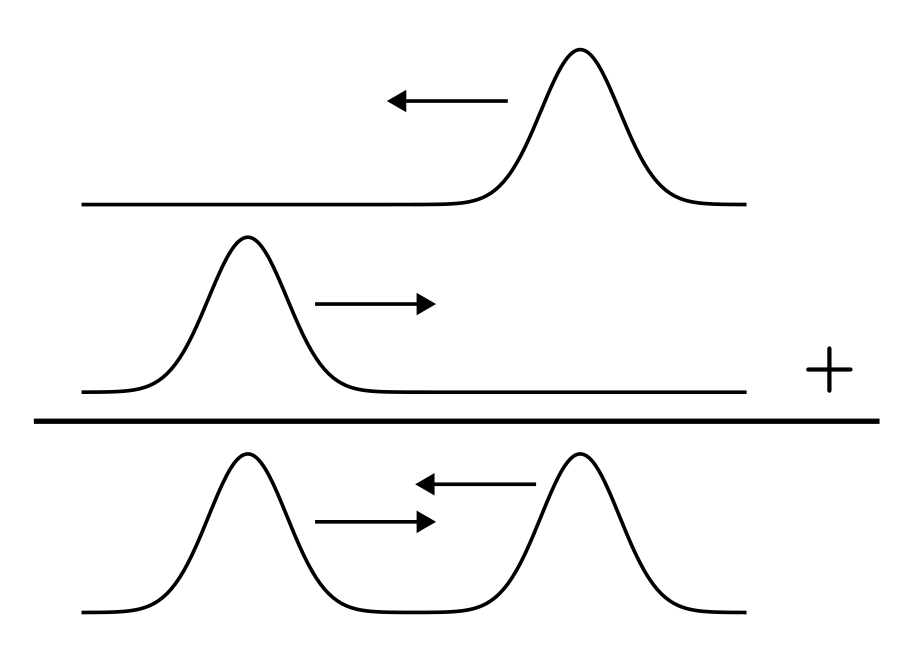
I was told that a quantum mechanical system is completely determined by its wave function. But superposition principle says that given two wave functions of some system, a linear combination of them is also a wave function for the same system.
I'm confused, how can it be that the same system have more than one wave function. How to clarify this confusion?
I think that this means that every wave-function corresponds to a particular state of the system, but I don't understand what does that mean? What is the exact meaning of a state of a system in this context? Can you give some examples of states of a system?