I want to know the proof for the following statement:-
The net electric field due to the "induced charges on the surface of the cavity and the charge inside the cavity" is zero everywhere outside the cavity.That is if the charge distribution of the surface of the cavity and the charge inside were imagined to be isolated and with the same configuration the net electric field outside it would be zero .
Perhaps one can think this way: When you place a, say, positive charge in the cavity, what happens is that the electric field generated by it spreads around. This field drives the positive charges on the surrounding conductor away from the center, and attracts the negative charges towards the center. This seperation creates an internal field which counterbalances the inducing field exactly (otherwise, the process continues to go on), making the total field inside the conductor zero. Use Gauss's theorem on the graph you draw will know that total Q inside should be zero, and negative charges should exist on the internal surface.
The electric field inside a conductor must be zero so the net charge within the Gaussian surface must be zero.
Thus the charge $+Q$ must induce a charge of $-Q$ on the inside of the conductor.
If the conductor was originally uncharged then this in turn must lead to the conclusion that the charge induced on the outside of the conductor must be $+Q$.
This arrangement is a capacitor with zero electric field outside the capacitor.
Is possible to give a proof of this statement (See Griffiths pag. 121):
In a volume $V$ surrounded by conductors the electric field $\textbf{E}$ is uniquely determined if the total charge on each conductor is given.
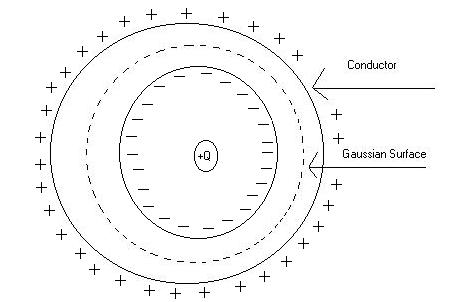
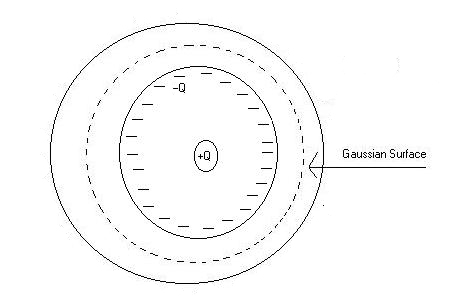
Now consider your example. I want to know the field due to the charge inside and on the surface of the cavity in a point $P$ external to the cavity. What happens if I let expand the outer surface of the conductor? The charge on the conductor remains the same (zero) and also the cavity remains the same (its shape), so the field inside the cavity doesn't change, thanks to the theorem.
There's more, also the charge configuration on the cavity surface doesn't change because of $$ \textbf{E} = \sigma/\epsilon_0 \textbf{n} $$ on the inner surface of the conductor. So the situation in the cavity is exactly the same as before.
But we can enlarge the outer surface as we want, such that the field due the plus charge outside evaluated in $P$ goes to zero (if the outer surface is far away) and $P$ is now inside the conductor. So we are in the same situation of your answer but we have eliminated the contribution from the outer surface. Since $\textbf{E}$ in $P$ is zero (we're now inside the conductor), the field due to the charge inside and on the surface of the cavity is zero.
