I want to know how the following equation comes from. $$f_D=\frac{1}{2\pi} \frac{\Delta\phi}{\Delta t}=\frac{v}{\lambda}\cos\theta$$
The picture above is drawn by me in mspaint (sorry for bad quality.)
Suppose an object is moving to right-side and transmitter is fixed.
Why $f_D=\frac{1}{2\pi}\frac{\Delta\phi}{\Delta t}$ and $\Delta\phi \approx 2\pi\frac{v}{\lambda}\Delta{t}\cos\theta$?
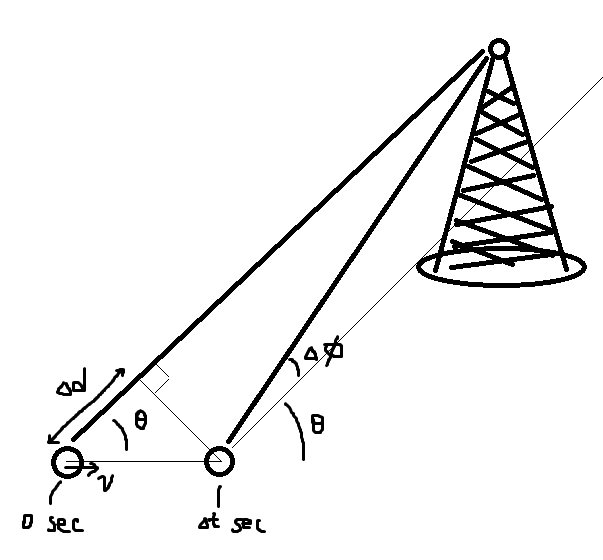
Frequency can be viewed as rate-of-change of phase, so in the formula there are 2 position at which the mobile device observes the transmitted wave field. There is one position at $t=0$ and another a $t=\Delta t$, with respective phases $\phi=\phi_0$ and $\phi=\phi_0+\Delta \phi$, giving an additional rate-of-change of phase $\frac{\Delta\phi}{\Delta t}$ above and beyond to the time-dependent part of the transmitted wave (at the carrier frequency). This is the Doppler shift, $f_D$. The $2\pi$ normalization converts from angular frequency (radians per second) to cycles-per-second (as a cycle is $2\pi$ radians).
To compute the phase change, consider your radial distance change from the source in the time $\Delta t$: it's $\Delta t v \cos{\theta} \frac{2\pi}{\lambda}$.
This is the pulsed-Doppler radar view of the Doppler shift, where you sample a wave field at different positions with respect to a stable-local-oscillator.
