1-Loop Mass Splitting of vector-like Fermions

Question

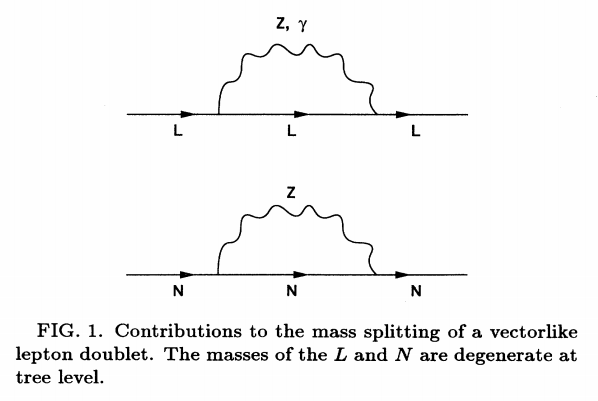
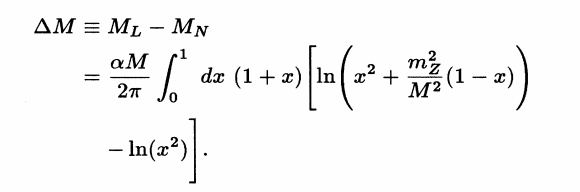In this paper the author argues that for a vector-like fermion doublet, with degenerate mass $M$ at tree level, we always have a mass splitting between the charged component of the doublet $L$ and the neutral component $N$

through the diagrams

The only further information he gives here is that "the mass splitting is easily calculated". At least for me it is not that obvious, but maybe someone more knowledgeable here can answer two little questions (for which I would need weeks to answer).

• Is there a similar splitting between possible vectorlike quarks with equal tree level mass $M$ and these leptons? In the paper the author states "For vectorlike quarks, the results are identical, with an additional factor of 1/3 due to the quark charges", however I'm not sure which mass splitting he means. A mass splitting between a vectorlike quark and the leptondoublet, between a vectorlike quark and the charged component $L$ or between a vectorlike quark and the neutral component $N$?
• Do we get a similar, bigger, splitting if we have an enlarged gauge symmetry and therefore additional, heavier gauge bosons that can appear in the loop?

Answer 1

Q1: The mass splitting he is referring to is between the components of a vector-like quark (VLQ) doublet, $Q=\begin{pmatrix} U \\D \end{pmatrix}$, i.e. between U and D. There is such a splitting because they have different charges, so they get different loop corrections to the tree- level mass term $M_Q \bar{Q}{Q}$. What Sher is saying is simply that we pass from VL leptons (VLL) to VLQ as \begin{equation} \Gamma_L = \frac{g^2 \Delta {M_L}^5}{960 \pi^3 M_W^4} \,\to\,\Gamma_Q = N_c\frac{g^2 \Delta {M_Q}^5}{960 \pi^3 M_W^4} \end{equation}

Hence the 1/3 in lifetime. (incidentally, I think there's a typo in quoting the new lifetimes, it should be nsecs instead of $\mu$secs.)

However, the expression of $\Delta M$ for VLL and VLQ is not the same of course (different hypercharges).

Q2: The (gauge bosons induced-)splitting between the components of a multiplet charged under a product of groups is uniquely determined by its charges under the different groups. In the example at hand of $SU(2)$ doublets with some hypercharge $y$ the splitting is fixed by isospin $t_3 = \pm \frac{1}{2}$ and $y$ only (plus the parameter of the gauge sector and the tree-level mass, of course). If we have higher $SU(2)$ multiplicities, or other groups, the expression of the mass splitting(s) change(s) accordingly.

Note that in specific models, the VL fermions can also have coupling to scalars. In which case the splitting can get a contribution from scalar loops as well.