Is it possible for a harmonic to be louder than the fundamental frequency?

Question

Using most sound equations, it is quite clear that increasing frequency decreases amplitude, but is there any example where this is not true?

Answer 1

It is not uncommon for some of the higher harmonics to have a larger amplitude. Take a look at the frequency spectrum of a trumpet for example.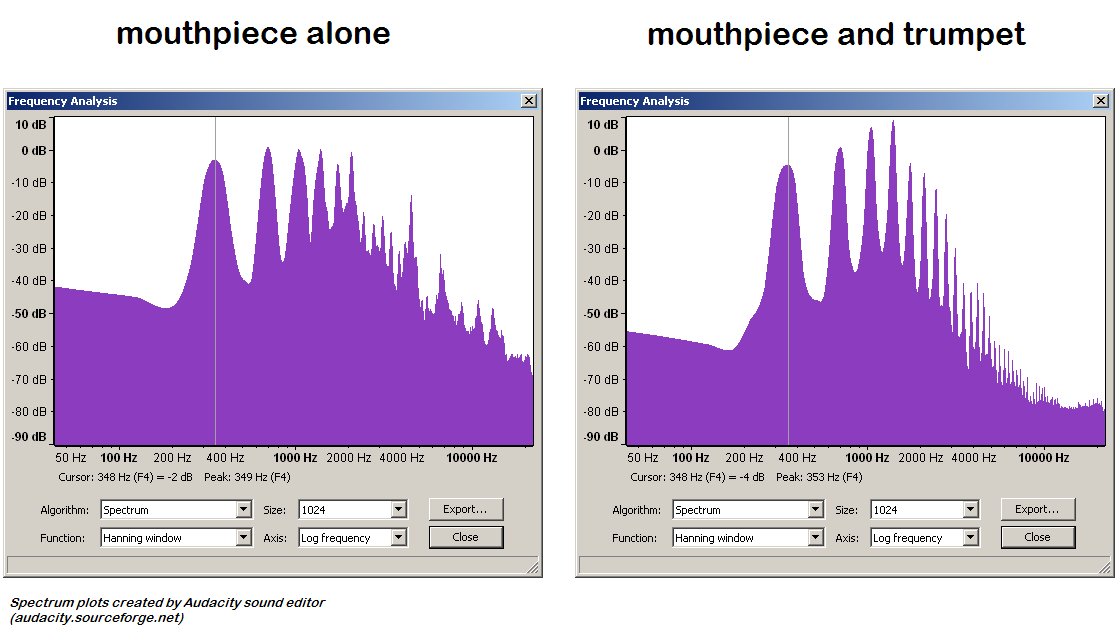

^{Image source: Subtractive Synthesis Concepts by Ed Doering, CC-BY licence.}

Answer 2

An example from the world of string instruments:

Strum a guitar string like normal, near the sound hole. Then gently touch near the 12th (octave) fret to mute the fundamental and the harmonics with antinodes at the center. Somewhere between "freshly strummed" and "fundamental muted" you can produce a sound which still has all the harmonics but where the fundamental is at low intensity.

Answer 3

Any non-linear resonator or amplifier can produce a phenomena called frequency-doubling. This was once used to produce blue light from red LEDs.

Often the input frequency is filtered out.

Answer 4

As said already, many sound sources etc. produce a spectrum where some of the harmonics exceed the fundamental in amplitude. As far as musical instruments are concerned, this is particularly typical for perceived loud instruments like trumpet. This has to do with the fact that the human ear is most sensitive around 3 kHz. Most musical notes have fundamental below 1 kHz, but much of the harmonics happens above. The loudness of an instrument depends a lot on how much energy it emits in the harmonics in the 2 ~ 5 kHz range (instruments like trumpet or snare drum concentrate their sound in that range, whereas e.g. bass recorder has much lower fundamental frequency and the overtones have little share in amplitude).

Interestingly, it turns out that the amplitude of the fundamental (or any other single harmonic) isn't even so influential on the total sound perception. In fact, it can be completely filtered out without altering the sound very dramatically – provided the first few harmonics are strong enough! This is not just theoretical. Actually, narrow filtering of single frequencies happens all the time in typical acoustic environments: reflections from straight walls create concrete delayed echoes, and that incurs comb filtering. This kind of filtering doesn't alter the relative amplitude of whole frequency bands (which is what's most crucial for the sound character), but it does cancel narrow intervals. If a tone happens to have its fundamental on one such response-notch of the room, you won't hear much of the fundamental at all, hence almost all harmonics may be louder than it!

---

In particular, as tfb remarks, the fundamental of bass notes is often filtered right out in small loudspeakers, and this may still give a decent hearing experience, though obviously not optimal – something is missing, and in particular, only the really deep bass range is felt with the body.