10
$\begingroup$

Suppose we have a positive +q charge and a -6q charge at some separation. Then will every field line originate from the +q and end up to -6q or will there be some extra lines coming to -6q from infinity because of higher charge to get 6 times the number of field lines? That is, will there be any line that does not originate from the positive charge but terminates at the negative one?

I think it should be that every line will originate from the positive and go to the negative, only difference will be in the density of the field lines. Am I right?

Also, if I talk about the flux now, why can I say that the flux near +q will be equal to that near -6q?

Improve this question
$\endgroup$

5 Answers 5

Reset to default
14
$\begingroup$

Then will every field line originate from the +q and end up to -6q 

No, every field line won't end to negative charge.

will there be some extra lines coming to -6q from infinity because of higher charge to get 6 times the number of field lines?

  Yes, many extra lines will come.

I think it should be that every line will originate from the positive and go to the negative, only difference will be in the density of the field lines. Am I right?

No, you are not right. Only some of field lines will end in -6q from +q.Yes, there will be a change in density gradient around charges.

 why can I say that the flux near +q will be equal to that near -6q?

Flux around two charges would be different. You can simply use Gauss law for flux.

Improve this answer
$\endgroup$
11
  • 1
    $\begingroup$ The blue lines on the right are not correct. There is no way that lower line can point upwards on the right. The field along the line joining the two charges points along the line, but you don't want to draw that field line because there is a zero field to the right and that field line would terminate there in empty space. But that's the field line that makes it farthest out, the rest turn back before there. For every five lines coming from infinity there is one that starts at the positive charge and terminates on the large negative charge. $\endgroup$
    – Timaeus
    Commented Mar 23, 2016 at 22:05
  • $\begingroup$ @Timaeus Is it okay now? $\endgroup$
    – Anubhav Goel
    Commented Mar 28, 2016 at 7:51
  • $\begingroup$ Hard to tell in a 2d drawing. You can talk about the field lines in general. But it's usually impossible to draw a consistent version with a finite number of field lines (where each one represents a certain amount of flux) and also have one of the finite number be one of the ones that terminates at the zero of the field. Further, you haven't actually addressed the original question. You correctly point out field like a come from infinity and hit the negative charge. But what about field lines coming out of the zero point? If you draw the field line going right and towards the zero $\endgroup$
    – Timaeus
    Commented Mar 28, 2016 at 14:12
  • $\begingroup$ Then there is also one going left towards the zero and coming from infinity. But you can't have two field lines, both going in to the zero point, and both terminating in the vacuum, not without some coming out as well. So field lines must come out of the zero as well, if you have some come in. And they terminate on the negative charge, which is relevant to the OP. In 2d you can have 2 go in and 2 go out, but in 3d you still only have two go in but a many could come out (but can only draw two). You didn't actually draw the zero point, it's not even on your picture. $\endgroup$
    – Timaeus
    Commented Mar 28, 2016 at 14:19
  • $\begingroup$ You are allowed to have multiple answers. So you could revert this one back to the version that got lots of votes and add a post script (or a header section) saying you now think it is wrong. Then you can keep all the points and don't have existing votes get applied to an essentially new answer. Plus you aren't misleading anyone if you have the post script, it tells people that a lot of other people liked that answer but you don't. And then you can still write a second answer if you want to try to give a complete and correct answer. $\endgroup$
    – Timaeus
    Commented Mar 28, 2016 at 15:15
13
$\begingroup$

This is really just a footnote to Anubhav's answer so accept his not this one!

Anubhav mentions Gauss's law, and this states:

The net electric flux through any closed surface is equal to $1/\varepsilon$ times the net electric charge within that closed surface.

So if you consider a spherical surface around the $+q$ charge the total flux through this surface will be $+q/\varepsilon$, and similarly the total flux through a similar surface around the $-6q$ charge will be $-6q/\varepsilon$. This tells you immediately that there must be flux lines from the $-6q$ charge that don't end on the $+q$ charge, because for this to happen the total flux through both surfaces would have to be equal.

Improve this answer
$\endgroup$
8
  • 2
    $\begingroup$ You are so modest. +1. $\endgroup$
    – user36790
    Commented Mar 23, 2016 at 10:20
  • $\begingroup$ @user36790: you did upvote Anubhav as well, yes? :-) $\endgroup$
    – John Rennie
    Commented Mar 23, 2016 at 10:21
  • 1
    $\begingroup$ @Benjamin: suppose I choose a Gaussian surface big enough to enclose both charges, then the total flux through that surface is $-5q/\varepsilon$ i.e. there must be a net flow of flux lines through this surface, and there are no other charges in the system outside the surface. So $-5q/\varepsilon$ worth of flux lines must go off to infinity. I don't understand why this is controversial. Suppose you had just one charge, then that would have flux lines going off to infinity because there are no other charges for the lines to end on. $\endgroup$
    – John Rennie
    Commented Mar 23, 2016 at 17:56
  • 1
    $\begingroup$ Obviously in the real world the universe is charge neutral (probably) so flux lines won't really go to infinity, but in the idealised model of a single isolated charge they would. $\endgroup$
    – John Rennie
    Commented Mar 23, 2016 at 17:57
  • 1
    $\begingroup$ @JohnRennie Almost all the field lines come from infinity or the positive charge, and almost all of them terminate on the negative charge. But in your answer you make is sound like they start on the negative charge and terminate on the positive charge. Which could be confusing to beginning students. You could stay there must be field lines that end on the -6q that don't start on the +1q charge (instead of saying there must be field lines that start from the -6q that don't end on the +1q). $\endgroup$
    – Timaeus
    Commented Mar 23, 2016 at 22:13
2
$\begingroup$

Then will every field line originate from the +q and end up to -6q or will there be some extra lines coming to -6q from infinity because of higher charge to get 6 times the number of field lines?

Some field lines will definitely come from infinity.

why can I say that the flux near +q will be equal to that near -6q?

I think you should focus on the flux through any surface enclosing the charges +q and -6q. And they are, according to gauss law, definitely not same.

Flux from surface enclosing -6q will be 6 times more than the flux from surface enclosing +q, even though the nature will be different.

Improve this answer
$\endgroup$
1
$\begingroup$

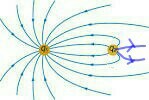
Electric field line start on a positive charge and finish on a negative charge or start/finish at infinity of start/finish at a neutral point.

Here is a computer generated diagram of electric field lines plotted for a +1 charge on the left and a -3 charge on the right (-6 was not available).

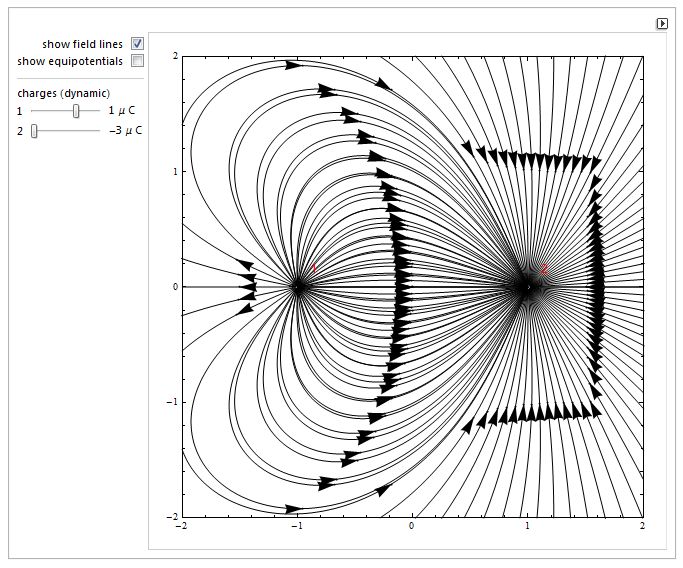
enter image description here

There seem to be a lot of electric field lines which go off the edge some of which never come back and even more which appear from nowhere? In an ideal world some/most? will in fact come back to a charge.

Two equal +1 charges give this diagram.

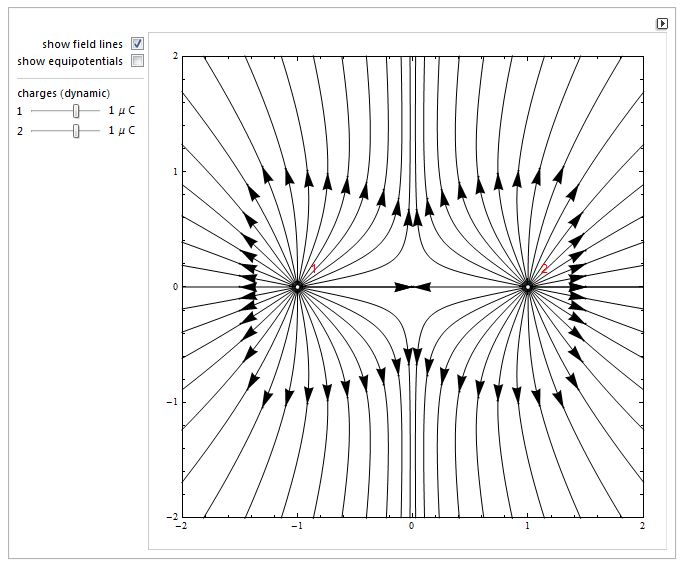
enter image description here

As well as electric field lines disappearing into the distance there are two lines between the two charges which terminate in the middle where the is no electric field (neutral point).

Faraday invented lines of force to explain his observations when he was doing experiments in electricity and magnetism.
He "counted" these lines and according to Faraday there should be three times more lines arriving at the -3 charge then leaving the +1 charge. This ties in with Gauss and the electric flux (number of lines passing through unit area).
So if electric field lines are conserved then where do the extra lines come from which finish at the negative charge?
Perhaps that does show that electric field lines are not conserved but you you have to remember that that this an ideal situation which tries to show what happens in the real world.
The diagrams of field lines which are drawn are visual aids and do not represent real lines and as such there is nothing wrong with allowing lines to start and finish at infinity.

One last point is that all the diagrams drawn in this question are not three dimensional which makes counting lines in a 2D representation inappropriate.

Improve this answer
$\endgroup$
-1
$\begingroup$

In such ideal problems, it is always implicitly assumed that there is no other charges in the Universe. So, if our Universe consists of only these two charges and everything else is neutral, then we cannot assume that field lines start at some infinity and end at individual charges. In fact, lines start from one charge and travel to infinity and then come back to the other charge. This picture is in line with the infinite-range nature of electromagnetic interaction.

Since flux is defined as integrated electric field over a surface, you can enclose your charges with identical (Gaussian) surfaces and attempt to calculate the integral. By Gauss's law, however, this integral is proportional to the enclosed charge. Then, it is trivial to see why bigger charge contains more flux than the smaller charge.

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ then you can also enclose both charges -- and see, that not every line coming from infinity has to have gone there, the number is simply different $\endgroup$
    – Ilja
    Commented Mar 23, 2016 at 7:07

Not the answer you're looking for? Browse other questions tagged or ask your own question.