The intensity of a sound wave is defined to be $$\vec{I}=p(x,t)\vec{v}(x,t)$$
I can see that the units on either side of the equation balance, but I can't find a derivation online that shows why $\vec{I}$ defined this way represents the power per area - the "wordsy" definition of intensity.
Here's a more-or-less rigorous discussion. The work done by a force acting over some small distance is $dW = \vec F\cdot d\vec s$. If the work is being done by gas with pressure $p$ pushing on some area $\vec A$ (where the "direction" of the area is perpendicular to the surface), and moving this surface by some distance $d\vec x$, then the work over the entire area is \begin{align} dW &= \vec F \cdot d\vec s \\ &= (p\vec A) \cdot d\vec x \\ &= p (\vec A \cdot d\vec x) = p\,dV. \end{align} The work per unit area is $$ \frac{dW}A = p \frac{dV}{A} = p\,dx, $$ where $dx$ is again the displacement of the surface. Then the power per unit area is $$ \frac{dP}{A} = \frac{dW}{A\,dt} = p\frac{dx}{dt} = pv, $$ where $v=dx/dt$ is the speed of the surface. It doesn't feel like too much of a stretch to say that the direction of the energy transfer will be in the direction of the velocity of the surface, as well.
To see that this treatment works for sound waves, imagine one-dimensional sound waves moving left-to-right in a pipe. (Image source)
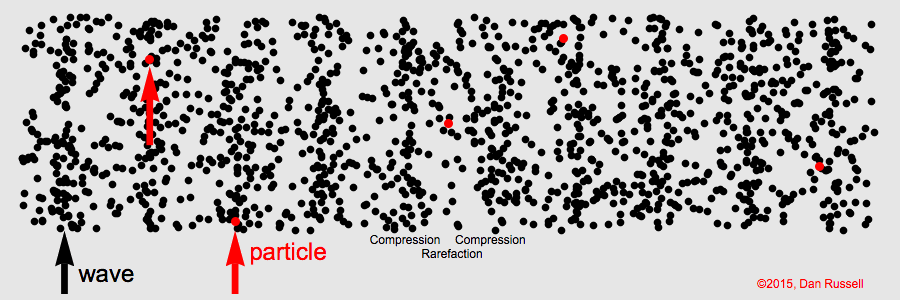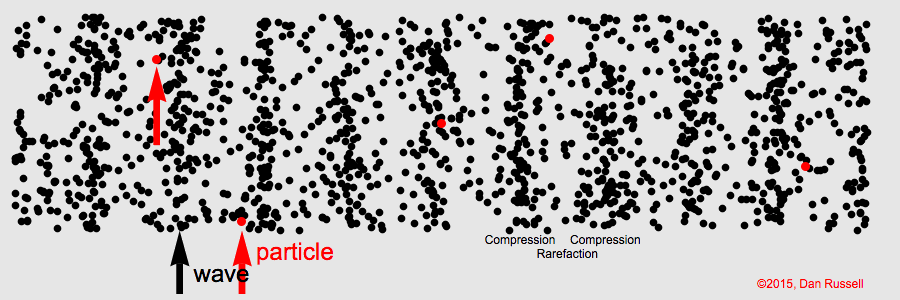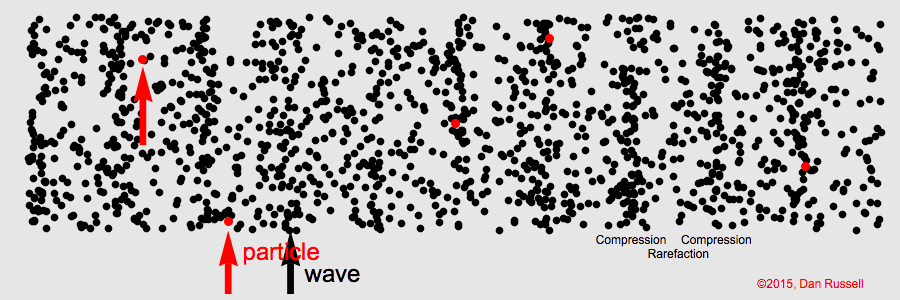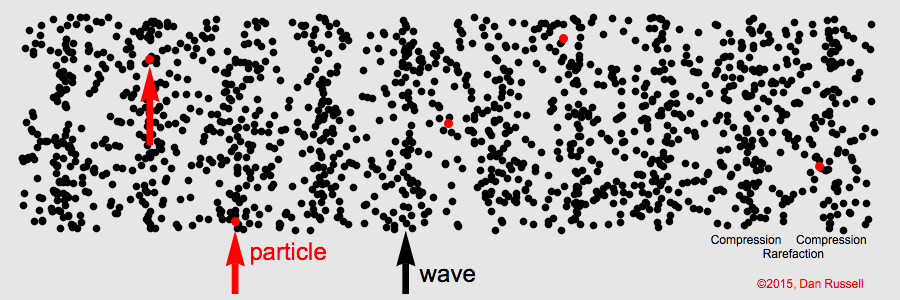
Make a small volume with constant mass with the location and approximate width of one of the red arrows: as the density near the arrow changes, your constant-mass volume will have to get bigger or smaller to accomodate. A compression entering your small volume from the left compresses your small volume, doing work on it. When the compression leaves your small volume to the right, then your small volume is expanding and doing work on the next little bit to the right. And so in this way work gets done by each little bit of gas in the pipe on the little bit to its right, and energy is transfered down the pipe.
If we model the pressure as a base pressure plus an oscillating term, $$ p = p_\text{atm} + p_\text{wave}\sin(kx-\omega t), $$ and the particle velocity as $$ \vec v = \vec v_\text{max} \sin(kx - \omega t), $$ then you can see that the instantaneous power at any given position $x$ may be positive or negative: energy enters and leaves each part of the air column. However only the oscillating part of the pressure, $p_\text{wave}$, will contribute to the power when averaged over many periods. The average power in the tube will therefore go like $P = p_\text{wave} v_\text{max} A$, in the same direction as $\vec v$.
