-1
$\begingroup$

As we go faster, our field of view increases, but would happen if we travelled at (almost) the speed of light?

My questions:

  1. Can we see a 360 degree view while travelling at (almost) the speed of light?

  2. Is there any formula to calculate the field of view w.r.t speed?

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ 1)no object with non-zero mass can accelerate to the speed of light. 2) it does not make any sense to speak of what the 'photon' observes, there's no frame of reference attached to it. $\endgroup$
    – Omar Nagib
    Commented Jan 26, 2016 at 19:50
  • $\begingroup$ @omar I have updated the question. $\endgroup$
    – chandrayaan1
    Commented Jan 26, 2016 at 20:49
  • $\begingroup$ One simply can't travel at the speed of light any more than one can go back in time. $\endgroup$
    – CuriousOne
    Commented Jan 26, 2016 at 20:56
  • $\begingroup$ @editinit No traveling at the speed of light, but the question may make sense at 0.999999c. In this case the answer is still no. The front shrinks to a flash of light due to the beaming effect, while the rear becomes pitch dark since light takes a long time to catch up with the observer. The RTR simulator, people.physics.anu.edu.au/~cms130/vrproject/rtr.html, can give you a realistic idea on how things look like visually. See also RTR clips on Youtube, "optical effects in relativity", and a "Slower Speed of Light" from MIT. $\endgroup$
    – udrv
    Commented Jan 26, 2016 at 21:38
  • $\begingroup$ It would appear as if the universe was receding away from you as you accelerated (increase of the field of view).Also, at the same time all the light reaching you would be blue-shifted until you would see a large white-blue-ish light (that's the cosmic background radiation blue-shifted). Check out a trip from earth to moon at the speed of light here : youtu.be/lD08CuUi_Ek?t=8m4s $\endgroup$
    – griffinwish
    Commented Jan 27, 2016 at 9:03

1 Answer 1

Reset to default
3
$\begingroup$

There is a fairly general discussion of relativistic aberration on John Baez's Physics FAQ site, and a more mathematical treatment on Wikipedia. The formula telling how the original angle is changed for the moving observer is:

$$ \cos\theta_O = \frac{\cos\theta_S - v/c}{1 - \cos\theta_S\,v/c} $$

I knocked up a quick graph in Excel to see what happens with increasing speed:

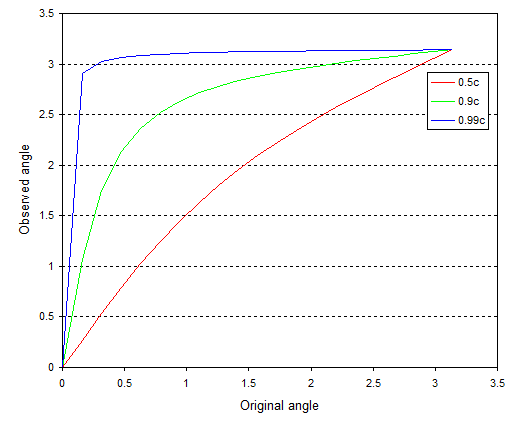
Aberration

The angle zero means directly behind while the angle $\pi$ means directly ahead. The graph shows that for values of $v/c$ near unity the filed of view is concentrated ahead of the observer as you describe.

As you approach $c$ the whole field of view is concentrated into a spot directly ahead of you. However light coming from directly behind you experiences no aberration even in the limit of $v \rightarrow c$. So you would never see the whole $2\pi$ no matter how fast you travel.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.