1
$\begingroup$

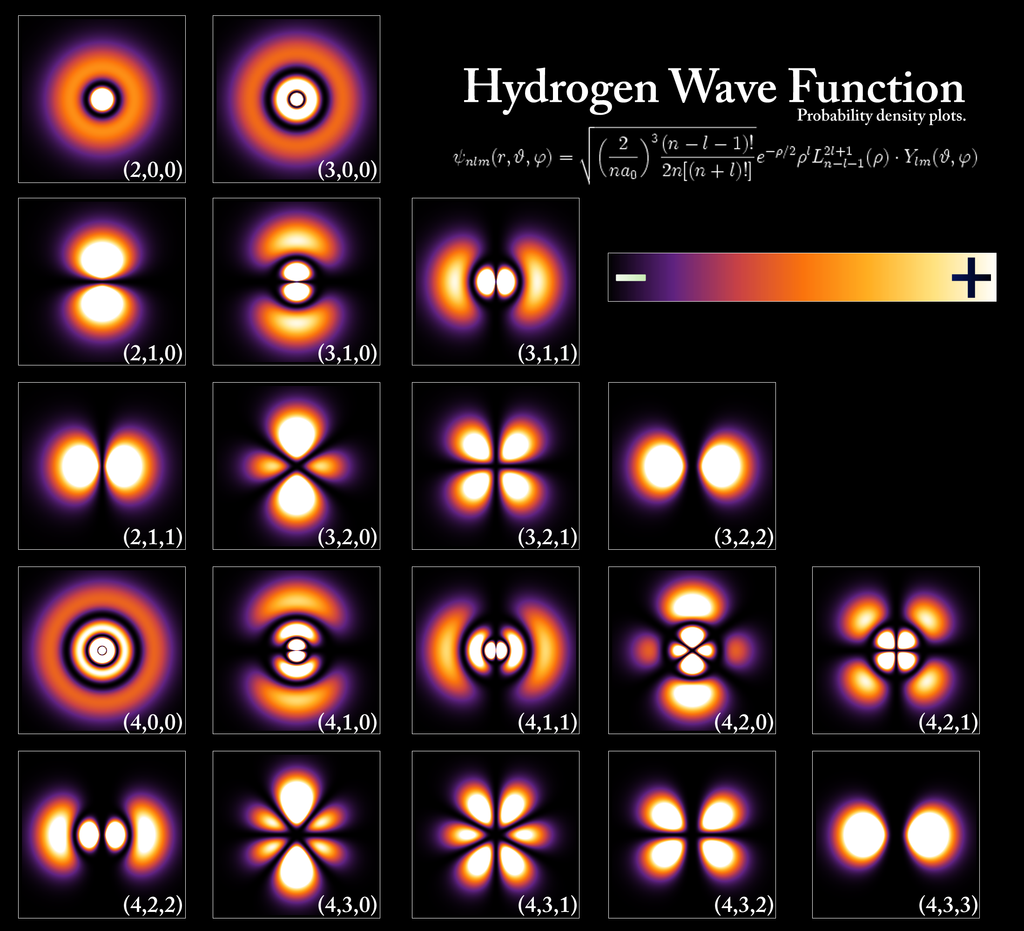
For example, this one:

enter image description here

I'm familiar with probability density functions, basic quantum mechanics, however, I don't know how to make sense of the chart. Specifically:

  1. Are the three numbers in the lower-right corner quantum numbers?
  2. What does each shape 'mean'/represent?
  3. It seems there are ~4 general "types/categories" of charts: concentric circle-type, reproducing cell-type, flower-type, pac-man-type (I hope these are easy to spot). Do members of each type/category share underlying properties?
  4. Is there a 'straightforward' relationship between the numbers and the shapes? Alternatively, is there a general principle that explains the relationship between the numbers and the shapes, such that knowing that principle (without rote memorization), you can tell roughly which numbers belong to each shape?
  5. How are these charts used, in current scientific inquiry (be it theoretical or in application)?

In short, what meaning/content do these graphics convey, and what application (if any) does this have?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

The shapes can be predicted if you know the azimuthal quantum number. L=0 s orbital and hence, spherical. L=1 p orbital dumbell shape. L=2 d orbital, double dumbell shape, or biconical shape. L=3 would be f orbital. The second number written corresponds to the azimuthal quantum number. Each shape shows you the electron density about the nucleus. Principal quantum number and azimuthal quantum number are entirely independent. For a given principal number n, azimuthal number can vary from 0 to n-1. Both of the quantum numbers contribute to the energy of the electron. The dark places you see between shapes are nodes. At a node probability of finding an electron is 0. There are two types of nodes-radial and angular. Take (2,0,0) for example. The dark spot would be of 0 probability. In radial nodes, at a given radius, probability of finding electron is 0. In radial nodes, probability of finding electron is 0 along a surface.in 3,1,0 the zx plane is an angular node and the region separating the two lobes of different sizes would be radial node. If principal quantum number is n and azimuthal quantum number is l then number of radial nodes are n-l-1 and number of angular nodes are l .check this https://en.m.wikibooks.org/wiki/General_Chemistry/Shells_and_Orbitals

Improve this answer
$\endgroup$
2
  • $\begingroup$ The answer was a bit hard to follow, but the link helped. $\endgroup$
    – Khashir
    Commented Jan 27, 2016 at 20:13
  • $\begingroup$ tell me which part to elaborate $\endgroup$
    – Syomantak
    Commented Jan 28, 2016 at 14:32

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.