If you chose the frame to rotate with $w_1$ instead of $w_2$, wouldn't the angular acceleration be
$w_2 \times (w_1 + w_2)$?
and it would have $+20 \jmath$ instead? Shouldn't the absolute acceleration be the same both ways? Am I missing something? I understand the angular acceleration formula to be the angular acceleration with respect to your frame plus the angular velocity of the reference frame with respect to the fixed frame cross the total angular acceleration.
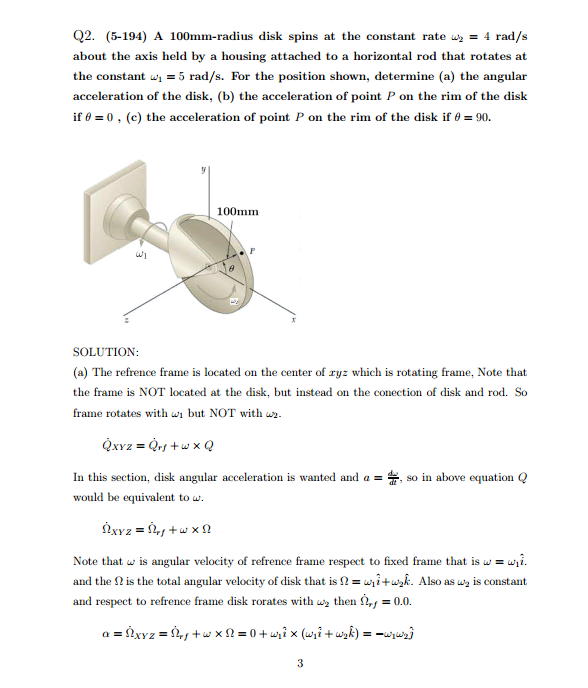
When trying to consider rotating frames you can easily get confused by the rotations and the notation. I struggled with this, until I decided to take a step by step approach when dealing with a serial chain of rigid bodies each with a relative rotation about a single axis. The key here is to move from the absolute rotations to relative rotations such that
$$ \begin{align} \vec{\omega}_1 & = \hat{x} \dot{q}_1 \\ \vec{\omega}_2 & = \vec{\omega}_1 + \hat{z} \dot{q}_2 \end{align} $$
where the relative (scalar) rotations are $\dot{q}_1 = 5$ and $\dot{q}_2= 4$. Now to differentiate the above you have to consider on which frame are the unit axes $\hat{x}$ and $\hat{z}$ rotating about. For each body the rotation axis moves with the previous body such that
$$ \begin{align} \dot{\hat{x}} & = \vec{\omega}_{ground} \times \hat{x} = 0 \\ \dot{\hat{z}} & = \vec{\omega}_1 \times \hat{z} = (\hat{x} \times \hat{z}) \dot{q}_1 \end{align}$$
So the rotational accelerations are
$$ \begin{align} \vec{\alpha}_1 &= \dot{\hat{x}} \dot{q}_1 + \hat{x} \ddot{q}_1 =0 \\ \vec{\alpha}_2 & = \vec{\alpha}_1 +\dot{\hat{z}} \dot{q}_2 + \hat{z} \ddot{q}_2 = (\hat{x} \times \hat{z}) \dot{q}_1 \dot{q}_2 \end{align} $$ since $\ddot{q}_1 =0$ and $\ddot{q}_2 = 0$ due to the constant rates. You will find that $\hat{x} \times \hat{z} = -\hat{y}$.
