Apparent failure of SUSY nonrenormalization theorem

Question

I am having trouble reconciling two pieces of information.

Consider supersymmetric QED, i.e. a supersymmetric U(1) gauge theory with two chiral superfields of opposite charges, $h$ and $\hat{h}$. The Kähler potential $K$ is canonical, $ K = h^\dagger e^{2\,g\,q\,V} h + {\hat{h}}^\dagger e^{-2\,g\,q\,V} \hat{h} , $ while the superpotential $W$ is the simplest possible: $$ W = m\, h \, \hat{h}. $$

On the one hand

Renormalized mass and fields are related to bare/original ones by: $$m_0 = Z_m m_r, \qquad h_0 = {Z_h}^{1/2}\, h_r,\qquad \hat{h}_0 = {Z_h}^{1/2}\, \hat{h}_r.$$

The SUSY non-renormalization theorems say that $W$ is not perturbatively renormalized, implying that $$ Z_m Z_h = 1 \quad \Rightarrow \quad \delta_m = - \delta_h, $$ at the one loop level (as usual $Z_m = 1 + \delta_m$, $Z_h = 1 + \delta_h$). The counterterm Feynman rule for the scalar propagator of $h$ will then be: 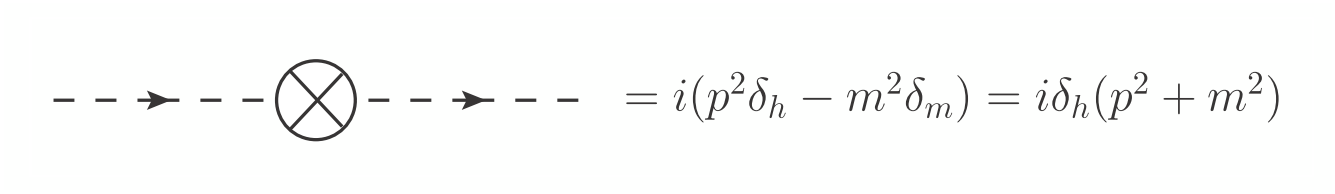i.e. the scalar propagator counterterm is proportional to $(p^2+m^2)$.

On the other hand

If one explicitly computes the divergent part of the $h$ self energy at one loop in dimensional regularization, one finds* that: $$ i \Sigma_h (p^2) \bigg|_\textrm{div} = i \frac{g^2 q^2 }{(4\pi)^2} \frac{2}{\epsilon} \big(-4 m^2\big). $$ _{*In the literature one can find this result e.g. in arXiv:hep-ph/9907393, section 4.3, equation (150), by playing with the integrals. This result is obtained in the Feynman gauge ($\xi = 1$).}

i.e. the divergent part of the self-energy at one loop is proportional to just $m^2$.

QUESTION: I was expecting a divergent part proportional to $(p^2 + m^2)$, which is what can be cancelled by the aforementioned counterterm. Is this reasoning correct? What might have gone wrong?

Comments

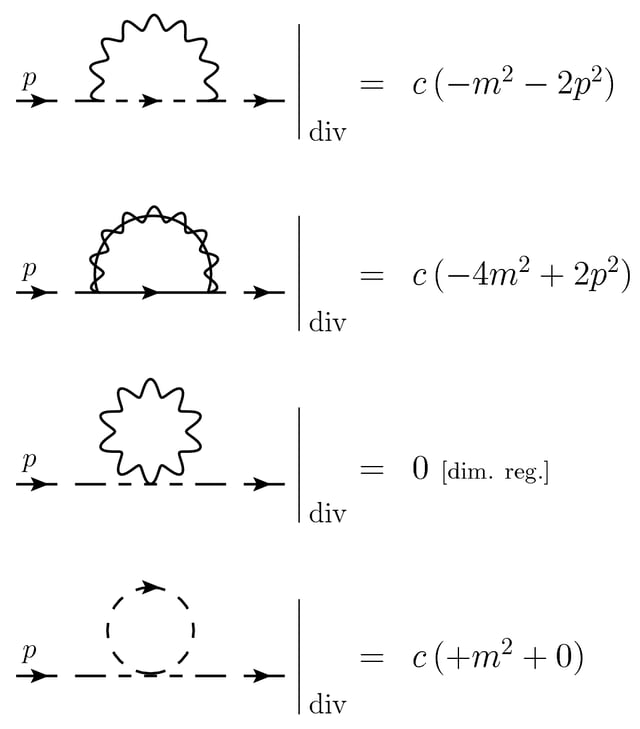
Diagrams

To make this question more accessible, here are the diagrams (usual Feynman diagrams, not supergraphs) which sum to give $i \Sigma_h (p^2) \big|_\textrm{div}$:

I am assuming there is something naïve in my approach. Perhaps some subtlety with the gauge diagrams, or Wess-Zumino gauge (I don't know any more at this point).

Dimensional regularization

At the outset there doesn't seem to be a problem with using dimensional regularization. The SUSY violation introduced by it should be proportional to $\epsilon$ (Martin's SUSY Primer, p. 61), thus only affecting finite terms.

Symmetries and missing terms

An R-symmetry under which both $h$ and $\hat h$ have charge $+1$ forbids adding gauge invariant terms like $h \hat h$ to the Kähler.

The discrete symmetry under which $h \leftrightarrow \hat h$ and $V \rightarrow - V$ forbids adding a Fayet-Iliopoulos term.

Answer 1

There actually can be a violation of no-renormalization theorem in case where massless fields are involved in the the computation as in the first and second graphs. These inconsistencies appear as finite corrections to effective potential and are a result of a non-local term which can modify superpotential.

A non-local term like $\int d^4x d^4\theta \frac{1}{\Box} D^2 W(\Phi)$ is not taken into account while proving no-renormalization theorem (to maintain locality) but can cause a correction to superpotential as using, $\int d^4\theta\rightarrow \int d^2\theta \bar{D}^2$ and $\bar{D}^2D^2\Phi=\Box\Phi$ gives precisely a term like $\int d^2\theta d^4x W(\Phi)$ and modifies the superpotential.

This non-local term can arise while computation in perturbation series and has been known for quite some time. Consult following references and references therein-

1- P.C. West, Phys. Lett. B 258, 375(1991).

2-I. Jack, D.R.T. Jones and P.C. West, Phys. Lett. B 258, 382(1991).

3- N. Seiberg, Phys. Lett. B 318, 469(1993)