I am having trouble reconciling two pieces of information.
Consider supersymmetric QED, i.e. a supersymmetric U(1) gauge theory with two chiral superfields of opposite charges, $h$ and $\hat{h}$.
The Kähler potential $K$ is: $$ K = h^\dagger e^{2\,g\,q\,V} h + {\hat{h}}^\dagger e^{-2\,g\,q\,V} \hat{h} , $$ canonical, $ K = h^\dagger e^{2\,g\,q\,V} h + {\hat{h}}^\dagger e^{-2\,g\,q\,V} \hat{h} , $ while the superpotential $W$ is the simplest possible: $$ W = m\, h \, \hat{h}. $$
On the one hand
Renormalized mass and fields are related to bare/original ones by $m_0 = Z_m m_r$, $h_0 = \sqrt{Z_h} h_r$, $\hat{h}_0 = \sqrt{Z_h} \hat{h}_r$. We also define $Z_m = 1 + \delta_m$, $Z_h = 1 + \delta_h$.: $$m_0 = Z_m m_r, \qquad h_0 = {Z_h}^{1/2}\, h_r,\qquad \hat{h}_0 = {Z_h}^{1/2}\, \hat{h}_r.$$
The SUSY non-renormalization theorems say that $W$ is not perturbatively renormalized, implying that
$$
Z_m Z_h = 1 \quad \Rightarrow \quad \delta_m = - \delta_h,
$$
at the one loop level (as usual $Z_m = 1 + \delta_m$, $Z_h = 1 + \delta_h$).
If we then proceed to write the The counterterm Feynman rule for the scalar propagator of $h$, we get will then be:
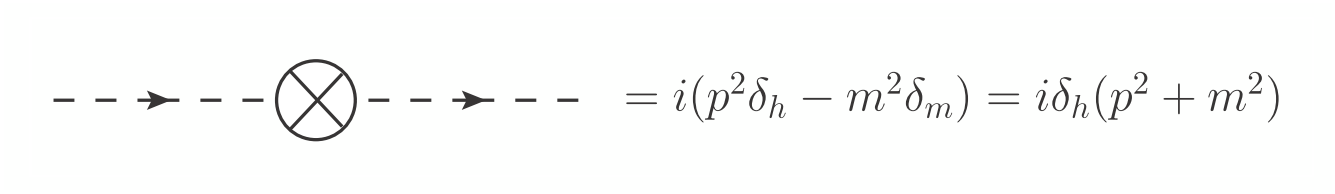
i i.e. the scalar propagator counterterm is proportional to counterterm is proportional to $(p^2+m^2)$.
# On the other hand
If one explicitly computes the divergent part of the $h$ self energy at one loop in dimensional regularization, one finds* that: $$ i \Sigma_h (p^2) \bigg|_\textrm{div} = i \frac{g^2 q^2 }{(4\pi)^2} \frac{2}{\epsilon} \big(-4 m^2\big). $$
i.e. the divergent part of the self-energy at one loop is proportional to just $m^2$.
However, I was expecting a divergent part proportional to $(p^2 + m^2)$, which is what can be cancelled by the aforementioned counterterm. Is this reasoning correct?
Any suggestions as to what might have gone wrong?
*In the literature one can find this result e.g. in arXiv:hep-ph/9907393, section 4.3, equation (150), by playing with the integrals. This result is obtained in the Feynman gauge ($\xi = 1$).
i.e. the divergent part of the self-energy at one loop is proportional to just $m^2$.
**QUESTION:** I was expecting a divergent part proportional to $(p^2 + m^2)$, which is what can be cancelled by the aforementioned counterterm. Is this reasoning correct? What might have gone wrong?
# Extra detailsComments ## Diagrams
To make this question more accessible to anyone willing to help, I can say that I have performed the computation in components, and I foundhere are the following diagrams (usual Feynman diagrams, whosenot supergraphs) which sum isto give $i \Sigma_h (p^2) \big|_\textrm{div}$:
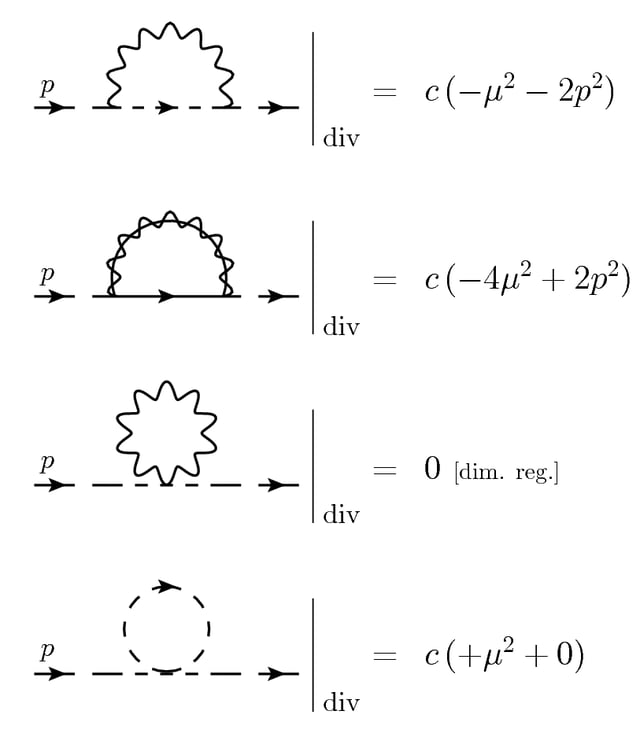
Surely the mistake must be on the computation of one of these. Since I repeated the math so many times,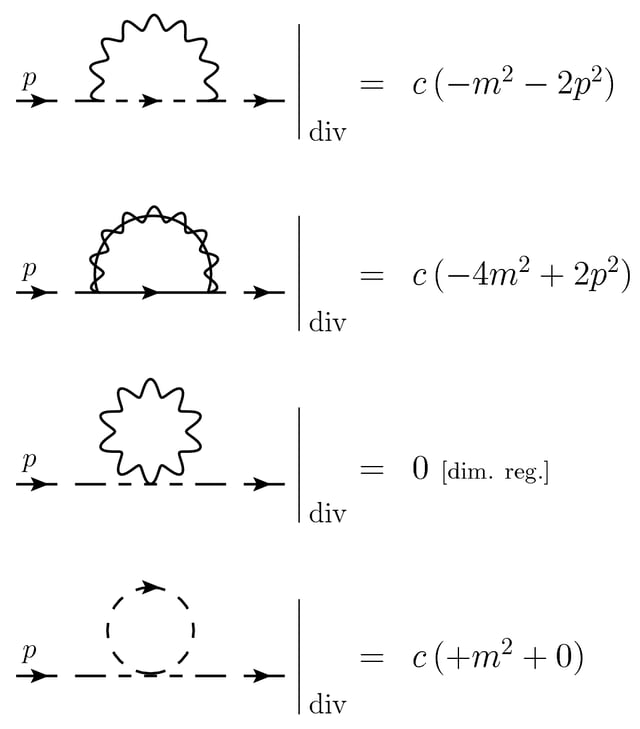
I am assuming there is something naïve in my approach. Perhaps some subtlety with the gauge diagrams, or Wess-Zumino gauge (I don't know any more at this point).
Dimensional regularization
At the outset there doesn't seem to be a problem with using dimensional regularization. The SUSY violation introduced by it should be proportional to $\epsilon$ (Martin's SUSY Primer, p. 61), thus only affecting finite terms.
Symmetries and missing terms
An R-symmetry under which both $h$ and $\hat h$ have charge $+1$ forbids adding gauge invariant terms like $h \hat h$ to the Kähler.
The discrete symmetry under which $h \leftrightarrow \hat h$ and $V \rightarrow - V$ forbids adding a Fayet-Iliopoulos term.
