How can photons cause charges to attract? [duplicate]

Question

Photons are the force carrier of the electromagnetic force. I do not see how this could result in a transfer of momentum that attracts objects together.

Answer 1

For the forces between elementary particles we have Feynman diagrams, where there exists a mediating particle for the interaction. In the simplest diagrams: for the strong it is the gluon, for the weak it is Zs and Ws and for the electromagnetic it is the photon.

Here is Bhabha scattering, where the electron and the positron ( attractive force) are first order in the expansion and of low energy:

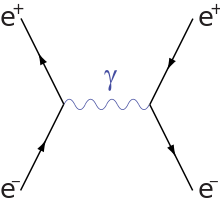
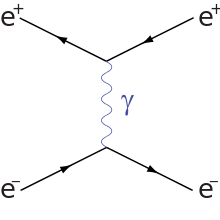
      annihilation                                  scattering

x axis the time axis.

For e-e- scattering only the second diagram exists at first order.

So the question should be how can there be attractive and repulsive forces. To really answer one would have to do the mathematics that the Feynman diagrams dictate and the result will tell us the the force is attractive.

I have found useful for intuitive understanding the analogue with boats throwing balls to each other, and transferring momentum for repulsion, and boomerangs for attraction.

repulsive analogue

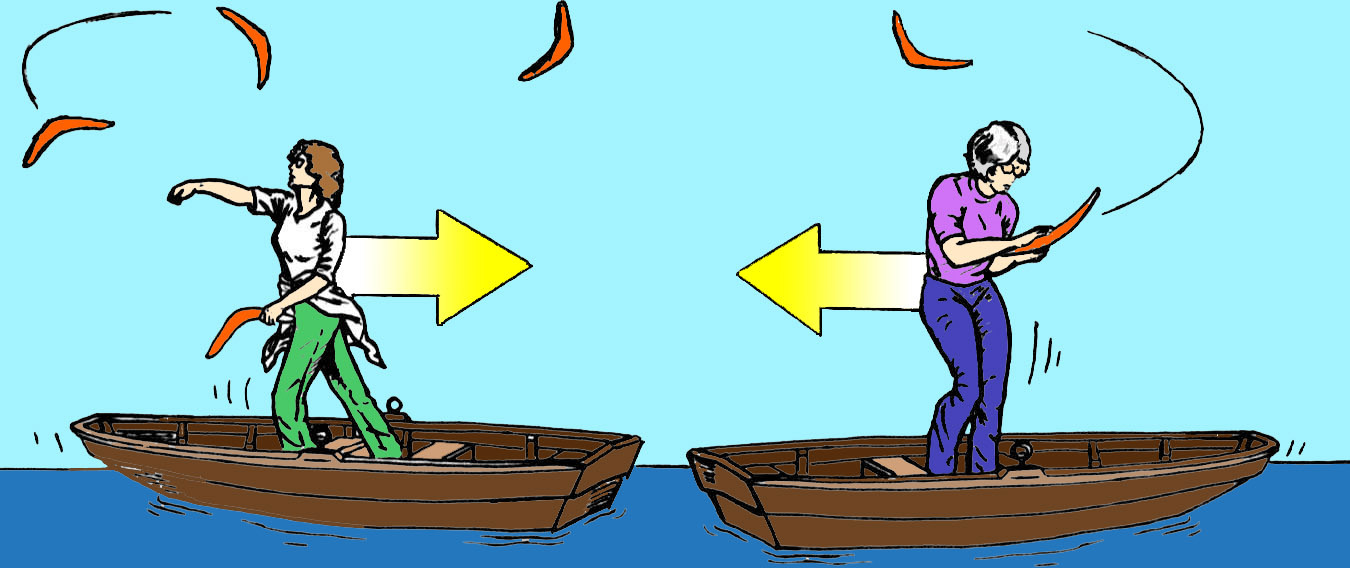
Momentum conservation directly for the repulsive, angular momentum conservation in the attractive. As all analogues it should not be stressed too much. Here we have a ball and a boomerang. It is a way to see that the boats can be "attracted" to each other.

In the Feynman diagrams the e+e- has an extra diagram to add to the calculation, and induce kinematically the attractive effect which the e-e- or e+e+ does not have.

Answer 2

Actually, A. Zee's book on "QFT in a nutshell" has a very nice explanation on this in chapter I.5, and I will briefly sketch it (this is a very rough sketch):

$$Z=\int DA e^{iS(A)} =e^{iW(J)}$$ where $W(J)$ is given by: $$W(J)=-1/2 \int \int d^4xd^yJ(x)D(x-y)J(y)$$ where D(x-y) is the photon propagator and J(x) and J(y) refer to two lumps of matter. Plugging in a photon propagator, we get: $$W(J)=1/2 \int d^4kJ^\mu (k) \frac{1}{k^2}J_\mu (k)$$

$J^0$ is the charge density which for two lumps of positive charge is positive and hence the result has a positive sign. If one lump was positive and other was negative, one of the J would be positive and the other J would be negative and we will get an overall negative sign, hence showing that there is an attraction between unlike charges and repulsion between like charges.

So photons basically act like carriers of information from one source to another. Extending this analysis further to other spin particles we get,

Exchange between like charges gives following forces according to spin

Spin 0 = Attractive

Spin 1 = Repulsive(photon)

Spin 2 = Attractive