1
$\begingroup$

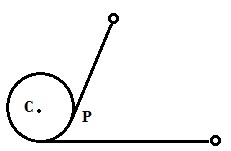
For a particle attached to a rope moving along a circle that has the length of rope as radius, the tension provides centripetal force and work done by tension is zero since velocity of particle is perpendicular to tension at any instant. But I am thinking about this case: top view

Here, a particle is tied to a vertical cylinder and is given a velocity perpendicular to rope. My doubt is the work done by tension. The length of rope decreases and the particle moves towards P which is along the direction of tension. So the work done is non zero. Am I correct? (Let the particle move in a frictionless surface)

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

No it is still zero.

Take $\theta$ to be the angle between the point at the bottom of the cylinder, C and P. Take the radius of the cylinder to be $R$ and max length of rope $L$.

The vector from C to P is $$r_{CP} = R(\sin \theta,-\cos\theta).$$

The vector from P to the particle is $$r_{T}=(L-R\theta)(\cos\theta,\sin\theta).$$

So the position vector of the particle is $r = r_{CP}+r_{T}.$ The tangent vector to the path of the particle is $$\frac{dr}{d\theta}=(L-R\theta)(-\sin\theta,\cos\theta)$$ which is perpendicular to the direction of tension $r_T$,

Improve this answer
$\endgroup$
6
  • $\begingroup$ So you are saying the particle has velocity perpendicular to tension. $\endgroup$
    – Aditya Dev
    Commented Aug 30, 2015 at 4:54
  • $\begingroup$ How did you get the tangent vector? I am not getting the same answer. $\endgroup$
    – Aditya Dev
    Commented Aug 30, 2015 at 4:58
  • 1
    $\begingroup$ Use the product rule on $dr_T/d\theta$. The term from taking the derivative of $R\theta$ cancels with the term from $dr_{CP}/d\theta$ $\endgroup$
    – octonion
    Commented Aug 30, 2015 at 5:02
  • $\begingroup$ This is my doubt. If you differentiate position vector of particle, shouldn't you get a vector perpendicular to that position vector? $\endgroup$
    – Aditya Dev
    Commented Aug 30, 2015 at 5:24
  • 1
    $\begingroup$ What you might be thinking of is if the position vector does not change its length, then you can show the tangent vector must be perpendicular. $\endgroup$
    – octonion
    Commented Aug 30, 2015 at 14:25

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.