1
$\begingroup$

I did some measurements on how tire pressure changes due to different loads in a car.

There are still some open questions I can't seem to solve on my own, even though I could already use parts of some answers from here: Does car tire pressure change with weight of car load?

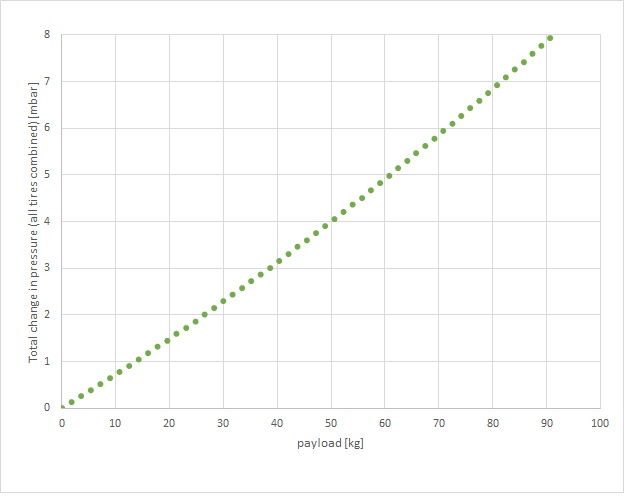
  1. This graph shows how much pressure is added by a specific payload put into the car. The car's net-weight is about 1,1tons. The initial pressure of all 4 tires was 3,20bar (absolute). How could I model and explain this quadratic-equation like behaviour? I would love to have an equation that I can give 80kg as input and then receive about 7mbar as output. (Tire is elastic; for the moment of change the behaviour is adiabatic I assume so we got p~1/V from pV=nRT; p=F/A; F=M*g; M increases and so does F, but A (between tire and street) does also change and so does V of the tire. But how exactly(well of coure more or less exactly, we don't know the exactly composition of the tire and neither do I want a too complex model of the tire)? The absolute values won't have to be true for this specific data, just the general behaviour. tire pressure vs car payload

  2. The change in pressure for the same weight on one of the front seats is higher. (70kg front is the same change in pressure like 80kg in rear seat) Pro Argument: The car's enginge is in the front, so it's like there is already more load, and when e.g. the first 50kg results in 4mbar pressure change, another 50kg leads to more than 4mbar change in pressure. But argument against: The weight is more well distributed between the front and rear tires, because the rear seats are like directly over the rear tires while the front seats are more in the middle of front and rear tires. This alone would therefore lead to a less increase in pressure. Maybe the first affect is dominant and the seconds just dampens the whole thing a little?

pressure distribution onto single tires

Improve this question
$\endgroup$
4
  • $\begingroup$ Hint - the tires' pressure in, say, $\frac{lbs}{sqr_inch}$ must be such that the number of square inches touching the ground multiplied by the pressure equals the car's weight. Now try generating a graph of tire pressure vs. the amount of deformation (change in contact area) $\endgroup$
    – Carl Witthoft
    Commented Aug 25, 2015 at 11:52
  • $\begingroup$ I just feel like there are too many unknown variables. I am looking for something like pressue = f(mass). I do have some pressure(mass) values. More mass -> F increases -> p increases -> V decreases but in the meantime also A increases so p decrease a little less. In which order do I need to unravel this? $\endgroup$
    – muhkuhdsp
    Commented Aug 25, 2015 at 12:58
  • 1
    $\begingroup$ Note that the pressure in all four tires will only be equal if the centre of gravity of the car projects exactly onto the centre of gravity of the rectangle made up by the four points contact between tires and floor. $\endgroup$
    – Gert
    Commented Aug 25, 2015 at 13:09
  • $\begingroup$ On load pressure: +increases due to Volume decrease and also due to P=F/A; F increase? Or would this be recursive? And the increase is dampened by the increase of contact are A $\endgroup$
    – muhkuhdsp
    Commented Aug 27, 2015 at 14:27

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Browse other questions tagged or ask your own question.