I know that the Foucault pendulum rotation in relation to Earth is a proof that the object is inertial in relation to the distant stars. But what makes them more important than the Earth? Are they an absolute and universal inertial frame? How can we prove that? Please elaborate.
$\begingroup$
$\endgroup$
3
-
$\begingroup$ Nobody claims that the distant stars are an inertial frame. But the center of mass of a sufficient number of distant stars is expected to be (the total momentum of the universe is 0). $\endgroup$– FabianCommented Jan 26, 2012 at 18:06
-
$\begingroup$ @Fabian why the center of mass of distant stars are more important than the mass of the Earth? AFAIK The gravitational effects of this small planet over the object are stronger. $\endgroup$– Jader DiasCommented Jan 26, 2012 at 18:09
-
1$\begingroup$ @Vam'çá it's not gravity that is causing the apparent rotation. I say "apparent" rotation because that's what it is. We are seeing the effect of a combination of real and fictitious forces that, when observed from our viewpoint as stationary with respect to the accelerating Earth, looks like rotation. $\endgroup$– Mark BeadlesCommented Jan 26, 2012 at 19:02
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
3
Actually the path of the Foucault Pendulum is not "fixed" (even approximately!) to the "fixed" stars. Unless the pendulum is installed at one of the Earth's poles (as someone has done), then the point of suspension is in constant rotation with the Earth itself. $\therefore$ the pendulum is really not in an intertial frame.
Consider a pendulum at the equator, swinging in a North South plane. It's obvious from symmetry that the plane of this pendulum doesn't rotate with respect to the earth and that, relative to an inertial frame, it rotates once every 24 hours. - UNSW, Austl.
A very good discussion of the forces (real and fictitious) on the pendulum can be found at this UNSW site. The vector that points from the suspension point toward the Earth is in constant acceleration and has a precession period that varies according to latitude.
This animation from the Wikipedia article on the Foucault pendulum may help show how the plane of the pendulum is rotating.
-
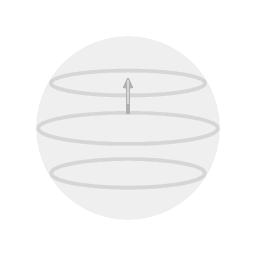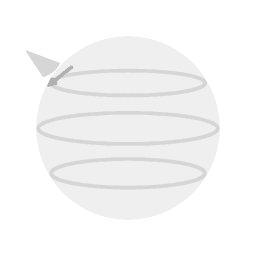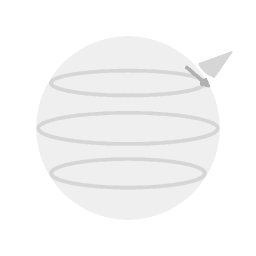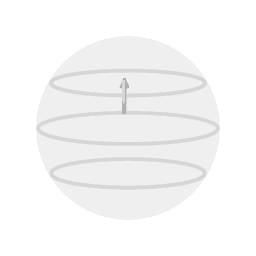
$\begingroup$ I thought it rotated in periods of 32 hours (the sidereal day) $\endgroup$ Commented Jan 27, 2012 at 13:14
-
$\begingroup$ The sidereal day is 23.93447 hours. $\endgroup$ Commented Jan 27, 2012 at 14:23
-
1$\begingroup$ Maybe he got confused when he read "At the latitude of Paris a full precession cycle takes 32 hours" from the Wikipedia article $\endgroup$ Commented Jan 28, 2012 at 16:21
$\begingroup$
$\endgroup$
8
It is not true that the Foucault Pendulum is "inertial in relation to the distant stars". The distant stars are moving in various random directions at various random speeds and are certainly not in the same inertial frame as the pendulum. Our galaxy is rotating, so it can't be used as an inertial frame. The visible universe is expanding and probably accelerating, so it's certainly not an inertial frame.
In Foucault's day the movement of the stars wasn't visible because they are so far away, so it was common to assume that the stars were fixed, and therefore represented a fixed framework that you could use as an absolute frame of reference. Actually that's not a bad approximation, but it is only an approximation.
answered Jan 26, 2012 at 18:25
-
$\begingroup$ If the distant stars aren't the inertial frame, why does the Foucault Pendulum rotates? I think it is inertial to something other than the Earth, what is that other thing? $\endgroup$ Commented Jan 26, 2012 at 18:43
-
$\begingroup$ The Foucault's Pendulum doesn't rotate. It carries on swinging in the same plane while the earth rotates beneath it. $\endgroup$ Commented Jan 26, 2012 at 19:06
-
2$\begingroup$ @JohnRennie What you say is true only at the poles. Elsewhere the pendulum must rotate. $\endgroup$ Commented Jan 26, 2012 at 19:54
-
$\begingroup$ @JohnRennie What determines the "same plane"? What is this plane referece? $\endgroup$ Commented Jan 27, 2012 at 13:05
-
1$\begingroup$ I suspect this is moving away from what Vam'çá will find helpful. I'm guess he's puzzled why what is apparently "locking" the pendulum in place. For the purposes of this discussion I suggest we assume the pendulum is at one of the poles - if you try the experiment at the equator you'll find there is no apparent rotation of the pendulum anyway. $\endgroup$ Commented Jan 27, 2012 at 15:15