Neutrinos have mass. But how much mass?

Question

Can somebody explain to me how much mass neutrinos have. According to my understanding neutrinos oscillate, therefore they should have some mass. If this is right then how much mass do they have?

Answer 1

The particle data group has a thorough article on neutrino oscillation experiments.

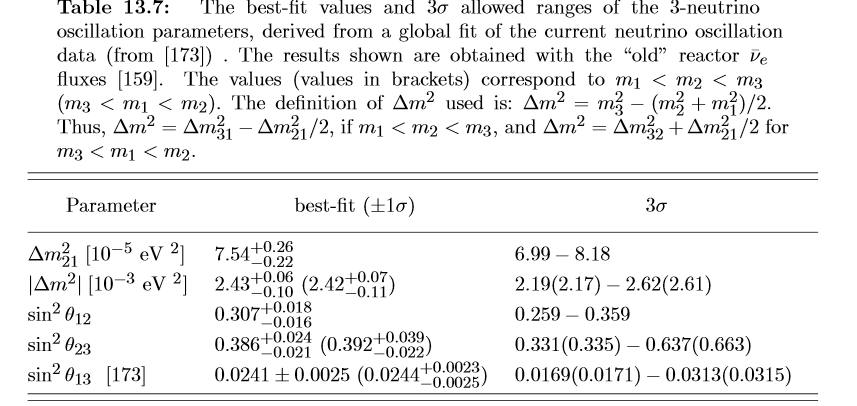
Oscillations are the way to measure mass differences between neutrinos, and in table 13.7 experimental values are given for delta(m^2) of the three neutrino species. These put a lower limit to the mass values.

From the definition it is obvious that the limits are of the order of electron volt or less, for the "heavier ones".

The experiments are not simple.

Answer 2

The mass of each of the three mass types of neutrinos is unknown (although a lower limit has been placed, as Anna V's answer indicates), chiefly because they exist in three weak classifications as well as the three mass classifications, and the combinations can not be simultaneously isolated and identified with certainty. The combined mass of all three mass types has been estimated, however.

Any one of the mass type neutrinos exists as a superposition of the three weak types, and any one of the three weak types exists as a superposition of the three mass types. So you can't identify a neutrino simultaneously as a definite mass type and a definite weak type. If you know one classification with certainty, you can't know which of the other three types it is with certainty.

When a neutrino propagates through space, the three mass types travel with three slightly different velocities. Because mass is determined by both energy and velocity, their masses differ slightly. This linked article explains how it's possible for three types of neutrinos that travel together to have slightly differing velocities: http://profmattstrassler.com/articles-and-posts/particle-physics-basics/neutrinos/neutrino-types-and-neutrino-oscillations/velocity-differences-of-neutrinos/.

As the neutrino moves through space, the different velocities cause it to oscillate among mixtures of the six types. Here is a better explanation of the six types of neutrinos and the process of oscillation: http://profmattstrassler.com/articles-and-posts/particle-physics-basics/neutrinos/neutrino-types-and-neutrino-oscillations/. The linked article was written by Matt Strassler in 2011, before the combined mass of the three types of mass neutrinos had been estimated by looking at anomalies in the Cosmic Microwave Background and gravitational lensing.

An experiment currently is being carried out in Germany (KATRIN: https://en.wikipedia.org/wiki/KATRIN) to precisely measure the mass of one type of weak neutrino.

The combined mass of all three types of mass neutrinos has been estimated to be 0.320 ± 0.081 eV/c^2, based on a 2014 study of anomalies in the Cosmic Microwave Background, combined with predicted gravitational lensing as though neutrinos were massless, compared with actual gravitational lensing. Here is an abstract of that study: http://adsabs.harvard.edu/abs/2014PhRvL.112e1303B.

It may be that some cosmological mass hitherto unaccounted for can be explained by the mass of neutrinos: http://physicsworld.com/cws/article/news/2014/feb/18/could-sterile-neutrinos-solve-the-cosmological-mass-conundrum.

Answer 3

Nobdody knows. There are many experiments that try to find this out right now. For example the Karlsruhe Tritium Neutrino (KATRIN) experiment. We only know that they must be very light $\approx$ eV.